Perfect Perimeters
Six dark-grey symbols (L, H, U, S, T, ) are drawn on six identical pieces of square paper.
How many of these symbols have the same perimeter as the square paper itself?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
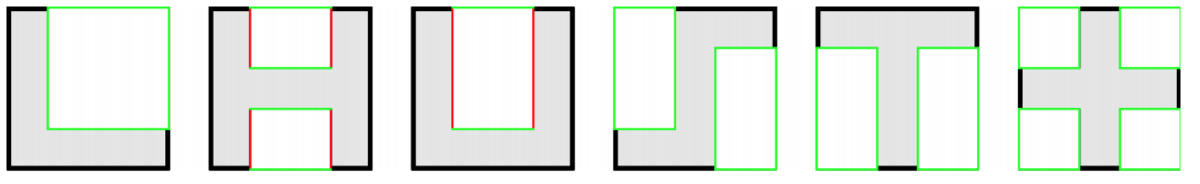 We can "extend" the sides to fit the entire square. Notice that L, S, T, and + all fit exactly, but H and U have extra parts (marked red in the picture). Thus the answer is
4
.
We can "extend" the sides to fit the entire square. Notice that L, S, T, and + all fit exactly, but H and U have extra parts (marked red in the picture). Thus the answer is
4
.