Tough Perimeter
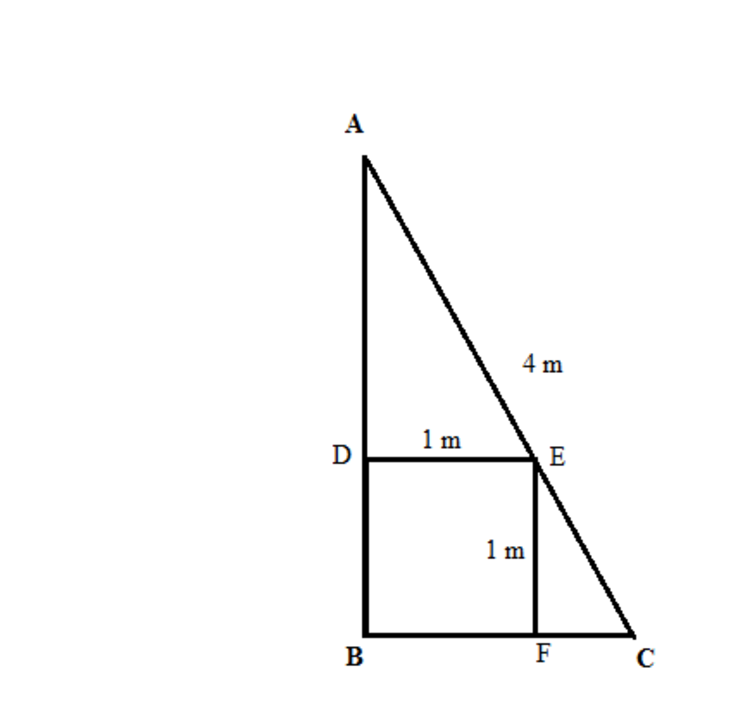 Here in
,
and
is a square of side length 1 unit. The perimeter of the
can be written as
where
and
are non-negative integers.What is the value of
?
Here in
,
and
is a square of side length 1 unit. The perimeter of the
can be written as
where
and
are non-negative integers.What is the value of
?
The answer is 22.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
let, A D = x and F C = y
and we know ∠ A B C = 9 0 ∘
we can write this equation(using Pythagoras): x + 1 ) 2 + ( y + 1 ) 2 = 1 6 .......... ( 1 )
again △ A D E and △ E F C is similar.
So,using similarity we acn say y 1 = 1 x or x y = 1 ...... ( 2 )
Now we have to solve ( 1 ) and ( 2 ) equation.
from equation 1 ,
x 2 + 2 x + 1 + y 2 + 2 y + 2 = 1 6
or, x 2 + y 2 + 2 ( x + y ) + 2 = 1 6
or, ( x + y ) 2 − 2 x y + 2 ( x + y ) + 2 = 1 6
or, ( x + y ) 2 − 2 ∗ 1 + 2 ( x + y ) + 2 = 1 6 [using equation ( 2 ) ]
or, ( x + y ) 2 − 2 + 2 ( x + y ) + 2 = 1 6
or, ( x + y ) 2 + 2 ( x + y ) − 1 6 = 0
or, a 2 + 2 a − 1 6 = 0 [ Let a = x + y ]
so, a = 1 7 − 1 o r , a = − 1 7 − 1 [ we can't take this value as it is negative]
so , x + y = 1 7 − 1
hence,perimeter of this triangle is △ A B C
= x + y + D B + B F + A C = 1 7 − 1 + 1 + 1 + 4 = 1 7 + 5
here m = 1 7 and n = 5
finally, m + n = 1 7 + 5 = 2 2