Perimeter / Radius = $2 \pi$

Drop a perpendicular from C onto diameter AB. Let the foot of perpendicular be D. Let centre of circle be O. We shall try to find $\angle B$ by first finding $\angle COA$ .

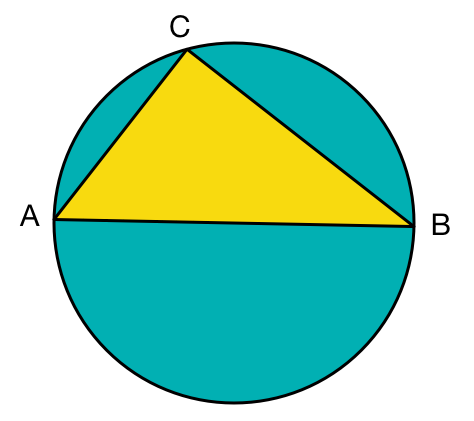
$\frac { \text{Area of Circle} } { \text{ Area of } ABC } = 2 \pi$

$\frac { \pi r^2 } { \text{ Area of } ABC } = 2 \pi$

$\text{ Area of } ABC =r^2/2$

$\text{ Area of } ABC =1/2 AB * CD$

And $AB=2r$

$CD=r/2$

In Triangle COD, we know CD and CO (=r). Thus $\sin ( \angle COA )=\frac{CD}{CO}$ or $\sin ( \angle COA )=1/2$ .

Thus angle COA is 30 while angle B is $\frac{\angle COA}{2}=15$

Thus answer is 15 .

Let $r$ be the radius of the circle. By Thales' Theorem , as $AB$ is a diameter $\Delta ABC$ is right-angled at $C$ , so

$|AC| = |AB|\sin(\angle B) = 2r \sin(\angle B)$ and $|BC| = |AB|\cos(\angle B) = 2r \cos(\angle B)$ .

The area of $\Delta ABC$ then equals $\dfrac{1}{2} |AC||BC| = \dfrac{1}{2} \times 4r^{2}\sin(\angle B)\cos(\angle B) = r^{2} \sin(2 \times \angle B)$ .

As the area of the circle is $\pi r^{2}$ , the given equation becomes

$\dfrac{\pi r^{2}}{r^{2} \sin(2 \times \angle B)} = 2\pi \Longrightarrow \sin(2 \times \angle B) = \dfrac{1}{2} \Longrightarrow 2 \times \angle B = 30^{\circ}$ or $150^{\circ} \Longrightarrow \angle B = 15^{\circ}$ or $75^{\circ}$ .

Since we require $\angle B \lt \angle A$ we see that $\angle A = 75^{\circ}$ and $\angle B = \boxed{15^{\circ}}$ .

WLOG let r=1. So area of circle = $\pi$ .
Area of ABC =1/2 * ab.
So ab=1. But $a^2+b^2=2^2.$ .
$\therefore (a+b)^2=6,........ (a-b)^2=2.\\ \therefore a+b= \pm \sqrt6,.......a-b=\pm \sqrt2.\ \therefore a=\dfrac{1 +\sqrt3}{\sqrt2}\\ \implies B=ArcCos\dfrac{1 +\sqrt3}{2\sqrt2}=\Large 15$

Start with the right triangle. Set one of the legs arbitrarily as 1, the other $a$ . Area of the triangle is $\frac{a}{2}$ .

Radius of the circle is half of the hypotenuse. $r=0.5\times\sqrt{1+a^2}$ .

Area of the circle is $\frac{\pi}{4}\times(1+a^2)$ .

Area of circle is is supposed to be $2\pi$ times area of triangle. This gives us a quadratic equations for $a$ : $a^2-4a+1=0$

The larger solution gives the smaller angle, and it is: $a=2+\sqrt{3}$

The angle is then $arctan(\frac{1}{2+\sqrt{3}})=15^\circ$ .