Perimeters and Areas!

Let $AO=a$ and $OD=b$ . Then the area of the red region is $\dfrac {\pi r^2}4 - \dfrac {ab}2 = 9\pi - 7$ . Assuming that $\dfrac {\pi r^2}4 = 9\pi \implies r = 6$ and $\dfrac {ab}2 = 7 \implies ab = 14$ . Given that $a+b = r + 2 = 8 \implies b = 8 - a$ . Then

$\begin{aligned} ab & = 14 \\ a(8-a) & = 14 \\ a^2 - 8a + 14 & = 0 \\ \implies a & = \frac {8 \pm \sqrt{8^2-4\cdot 14}}2 = 4 \pm \sqrt 2 \end{aligned}$

Assuming $b>a$ , then $a=4-\sqrt 2$ and $b=4+\sqrt 2$ . Then the perimeter of the red region:

$\begin{aligned} P & = \overline{AB} + \overline{AD} + \overline{DE} + \overbrace{BCE}^{\rm arc} \\ & = r-a + \sqrt{a^2+b^2} + r - b + \frac {2\pi r}4 \\ & = 2 + \sqrt 2 + 6 + 2 - \sqrt 2 + 3\pi \\ & = 10 + 3\pi \end{aligned}$

Therefore $\alpha + \beta = 10 + 3 = \boxed {13}$ .

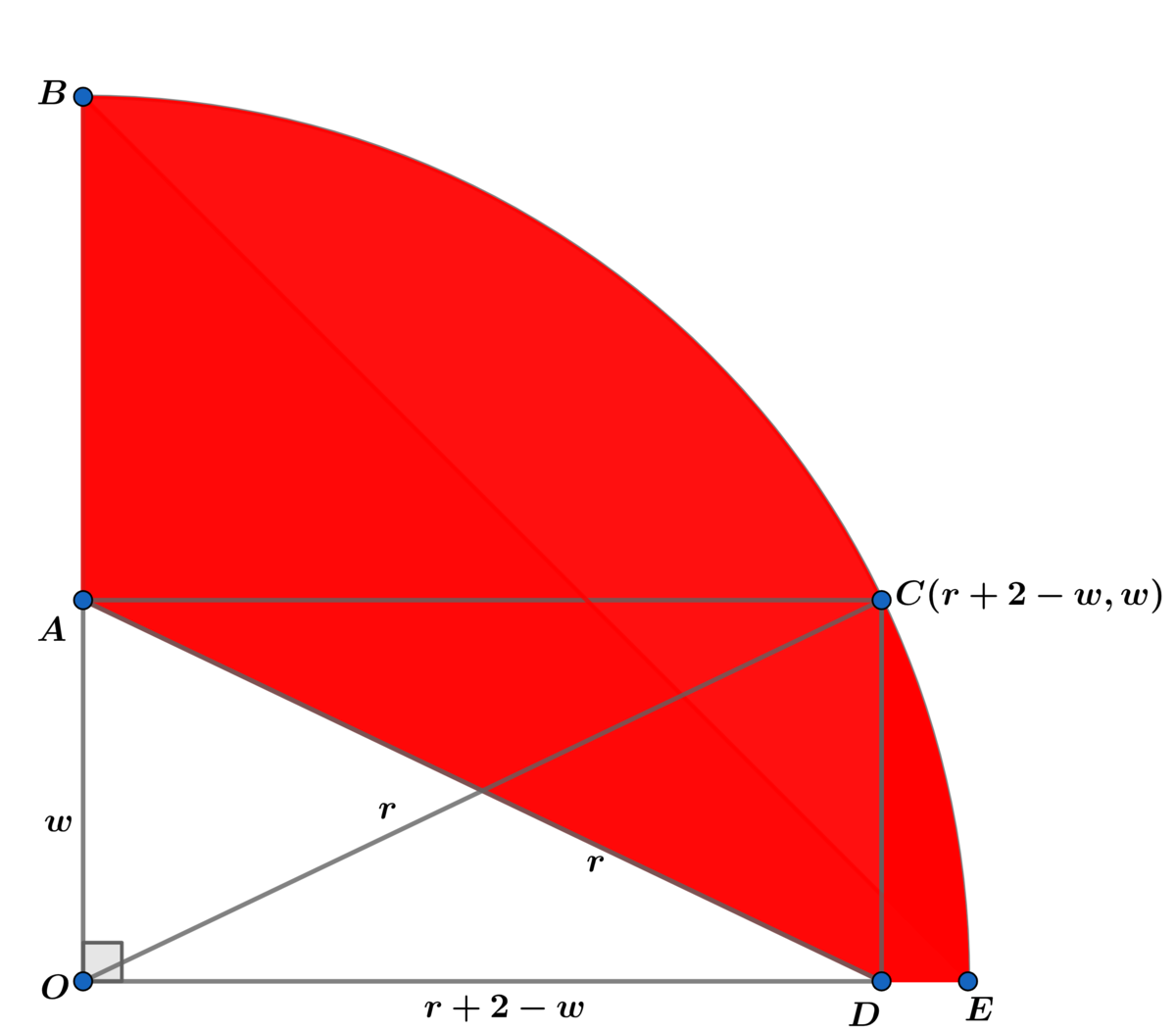
The diagonals of rectangle $OACD$ are congruent $\implies \overline{AD} \cong \overline{OC} = r \implies$

$P = r + (r - w) + r - (r + 2 - w) + \dfrac{\pi r}{2} = \dfrac{(4 + \pi)r - 4}{2}$

Using point $C$ above we have: $(r + 2 - w)^2 + w^2 = r^2 \implies$

$2w^2 - 2(r + 2)w + 4(r + 1) = 0 \implies w^2 - (r + 2)w + 2(r + 1) = 0 \implies$

$w = \dfrac{r + 2 \pm \sqrt{(r - 2)^2 - 8}}{2}$

Let $A_{R}$ be the red shaded region.

Using either value of $w \implies$

$A_{R} = \dfrac{1}{4}(\pi r^2 - \dfrac{1}{2}(r + 2 - \sqrt{(r - 2)^2 - 8})(r + 2 + \sqrt{(r - 2)^2 - 8})) =$

$\dfrac{1}{4}(\pi r^2 - \dfrac{1}{2}((r + 2)^2 - ((r - 2)^2 - 8))) = \dfrac{1}{4}(\pi r^2 - 4r - 4) = 9\pi - 7$

$\implies \pi r^2 - 4r + 12(2 - 3\pi) = 0 \implies r = \dfrac{2(1 \pm (3\pi - 1))}{\pi}$ dropping negative root

$\implies r = 6 \implies$ $P = \dfrac{20 + 6\pi}{2} = 10 + 3\pi =$ $\alpha + \beta\pi \implies \alpha + \beta = \boxed{13}$ .

Let $OD=AC=x$ and $OA=CD=y$ . First it is given that $x+y=r+2$ By Pythagoras’s theorem on right $\triangle OAC$ ,

$\begin{aligned} A{{C}^{2}}+O{{A}^{2}}=O{{C}^{2}} & \Rightarrow {{x}^{2}}+{{y}^{2}}={{r}^{2}} \\ & \Leftrightarrow {{\left( x+y \right)}^{2}}-2xy-{{r}^{2}}=0 \\ & \Leftrightarrow {{\left( r+2 \right)}^{2}}-2xy-{{r}^{2}}=0 \\ & \Leftrightarrow 4r+4-2xy=0 \ \ \ \ \ (1)\\ \end{aligned}$ Moreover, for the area of the red region we have

$\begin{aligned} \left[ ABCED \right]=\left[ OBCE \right]-\left[ OAD \right] & \Rightarrow 9\pi -7=\frac{1}{4}\pi {{r}^{2}}-\frac{1}{2}xy \\ & \Leftrightarrow 2xy=\pi {{r}^{2}}-36\pi +28 \ \ \ \ \ (2)\\ \end{aligned}$ Combining $(1)$ and $(2)$ , we have an equation for $r$ :

$4r+4-\pi {{r}^{2}}+36\pi -28=0\Leftrightarrow \pi {{r}^{2}}-4r+24-36\pi =0\overset{r>0}{\mathop{\Leftrightarrow }}\,r=6$ For the perimeter,

$\begin{aligned} P & =AB+BCD+ED+AD \\ & =\left( r-y \right)+\frac{1}{4}2\pi r+\left( r-x \right)+r \\ & =3r-\left( x+y \right)+\frac{\pi r}{2} \\ & =3r-\left( r+2 \right)+\frac{\pi r}{2} \\ & \overset{r=6}{\mathop{=}}\,10+3\pi \\ \end{aligned}$ For the answer, $\alpha=10$ , $\beta=3$ , thus, $\alpha+\beta=\boxed{13}$ .