Circling The Foot
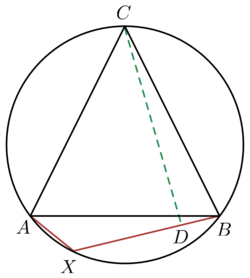 An isosceles triangle
has side lengths
.
is a point on the circumcircle of triangle
, lying on the arc between
and
not containing
, such that
and
. Let
be the foot of the perpendicular from
to
. What is the length of
?
An isosceles triangle
has side lengths
.
is a point on the circumcircle of triangle
, lying on the arc between
and
not containing
, such that
and
. Let
be the foot of the perpendicular from
to
. What is the length of
?
The answer is 200.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Construct the point Y on X B extended such that B Y = X A . Clearly C A X B is cyclic, so we have ∠ C A X = 1 8 0 ∘ − ∠ C B X = ∠ C B Y . Thus triangles C A X and C B Y are congruent by side-angle-side (with C A = C B , X A = B Y and ∠ C A X = ∠ C B Y ). Therefore C X = C Y and this implies that C X Y is an isosceles triangle. Since D is the foot of the perpendicular from C to X B , D is the midpoint of X Y . So X D = 2 X Y = 2 X B + B Y = 2 3 0 0 + 1 0 0 = 2 0 0 .