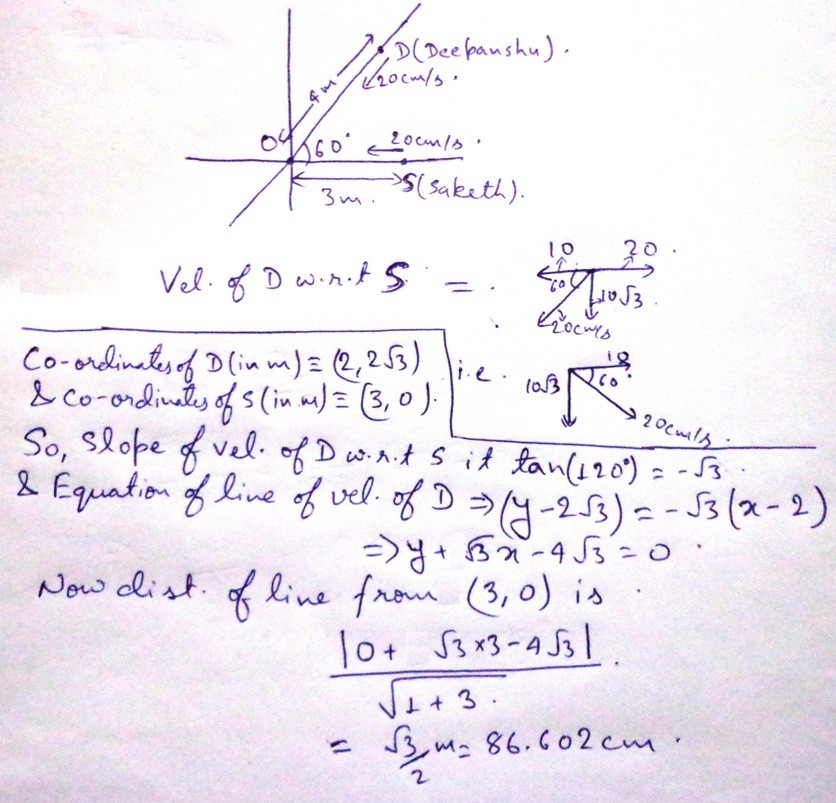
Perpendicular is the shortest distance

Classical Mechanics Level 5

Deepanshu and Mvs Saketh are moving along 2 long straight lines, in same plane , with same speed = 20 c m / s 20 ~ cm/s . The angle between the 2 lines is 6 0 ∘ 60^{\circ} , and their intersection point is O. At a certain moment, Deepanshu and Mvs Saketh are located at distances 3m and 4m from O , and are moving towards O . Find the shortest distance between them in centimetres .


The answer is 86.602.

View wiki
U Z by U Z , 24, Guyana

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

3 solutions

I used some physics and some mathematics to solve is easily.

4 Helpful 0 Interesting 0 Brilliant 0 Confused

Thought every unit to be in metre... even the speed!! Got wrong answer because of that. I used your same method...

Kishore S. Shenoy - 5 years, 11 months ago

Log in to reply

forgot to convert to metres :(

CH Nikhil - 5 years, 5 months ago

can someone tell. i didn't quite get it.

A Former Brilliant Member - 4 years, 1 month ago

Log in to reply

In the solution I am trying to see the motion of D as seen by by S. And then using the simple co-ordinate geometry to get the minimum distance.

Purushottam Abhisheikh - 4 years, 1 month ago
Jaikirat Sandhu
Jan 16, 2015

Let after time t they are closest. Then distance of Deepanshu from O is 300-20t cm. And that of Mvs Saketh is 400-20t cm. Then by using cosine law, the distance between them (Let it be x) x^2 = 400t^2 - 14000t + 130000. differentiating shows that at t = 17.5 sec. Then at t = 17.5 sec, x^2 = 7500. This means x = 50sqrt(3) cm = 0.86602 m = 86.602 cm.

1 Helpful 0 Interesting 0 Brilliant 0 Confused

I did it exactly in the same way. :)

satvik pandey - 6 years, 4 months ago

What is the Cosine law?? can you please elaborate??

Vikram Venkat - 6 years, 4 months ago

Log in to reply

In a triangle ABC, the side opp. to angle A is assumed of length 'a'. The side opposite B of length 'b' and that opposite to angle C as 'c'. Then Cos A = (b^2 + c^2 - a^2)/2bc Cos B = (a^2 + c^2 - b^2)/2ac Cos C = (a^2 + b^2 - c^2)/2ab

jaikirat sandhu - 6 years, 4 months ago

The symmetry of the situation requires that the shortest distance is reached when both have the same distance to O O . It is easy to see that this distance must 50 cm.

Their positions together with O O for an isosceles triangle with 12 0 ∘ 120^\circ top angle and legs of 50 cm. Calculate the length of the base: 50 ⋅ 2 ⋅ sin ⁡ 6 0 ∘ = 86.60 cm 50\cdot2\cdot\sin 60^\circ = 86.60\ \text{cm} .

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...