Planks across a ditch part 2

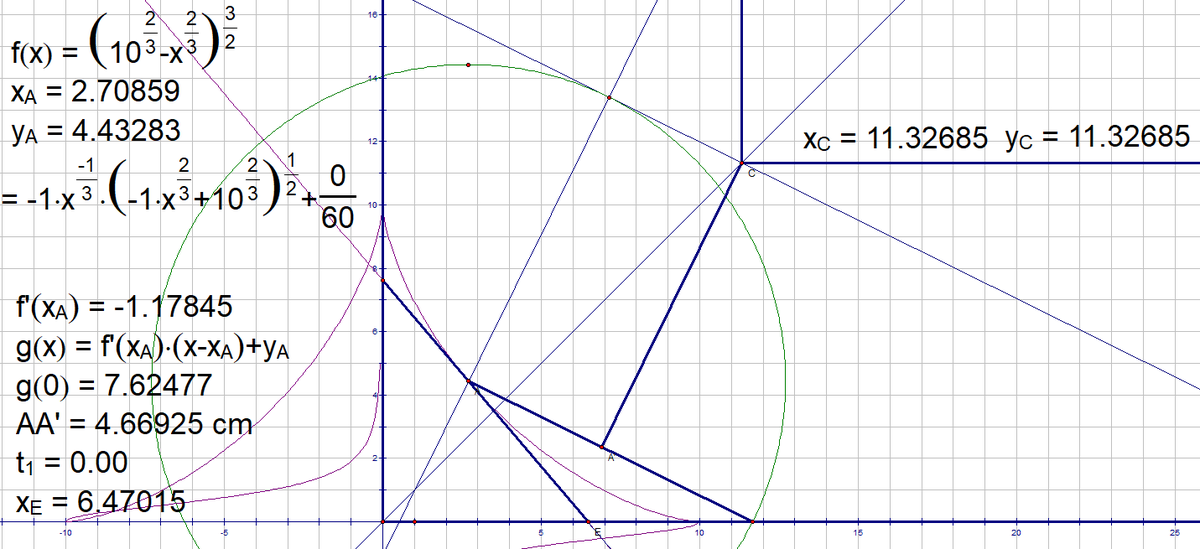
Let the planks be the lines $AB,CD,EF$ , each of length $10$ , and let $BC = x$ and $ED = y$ , and let $\angle OBA = \theta$ and $\angle ODC = \phi$ , while $\angle FED = 90^\circ$ .

Then $x\sin\theta = 10\sin\phi$ , and the coordinates of $F$ are $(X,X)$ , where $X \; = \; (10-x)\sin\theta + (10-y)\cos\phi + 10\sin\phi \; = \; y\sin\phi + 10\cos\phi$ and hence $\begin{aligned} y & = \; \frac{(10-x)\cos\theta + 10\sin\phi}{\sin\phi + \cos\phi} \\ X & = \; \frac{10 + 10\sin\phi\cos\phi + (10-x)\cos\theta\sin\phi}{\sin\phi + \cos\phi} \end{aligned}$ If we put $u = x\sin\theta$ and $v = (10-x)\cos\theta$ we deduce that $X \; = \; \frac{100 + u\sqrt{100-u^2} + uv}{u + \sqrt{100-u^2}}$ For the values of $x,\theta$ for which $X$ is maximized, we will have $\frac{\partial X}{\partial x} = \frac{\partial X}{\partial \theta} = 0$ , and hence $0 \; = \; x\cos\theta \tfrac{\partial X}{\partial x} - \sin\theta\tfrac{\partial X}{\partial \theta} \; = \; \big[(10-x)\sin^2\theta - x\cos^2\theta\big]\tfrac{\partial X}{\partial v}$ But it is clear that $\frac{\partial X}{\partial v} =\frac{u}{u + \sqrt{100-u^2}} > 0$ , and hence we deduce that $(10-x)\sin^2\theta - x\cos^2\theta =0$ , so that $x \; = \; 10\sin^2\theta$ This then implies that $\sin\phi = \sin^3\theta$ and that $X \; = \; 10\frac{1 + \sin^3\theta\sqrt{1 - \sin^6\theta} + \sin^3\theta\cos^3\theta}{\sin^3\theta + \sqrt{1-\sin^6\theta}}$ Maximizing this function of $\theta$ numerically over $(0,\tfrac12\pi)$ , we see that the maximum value of $X$ occurs when $\theta = 0.8665662$ , when $X = \boxed{11.326849}$ .

I created and solved this problem a few months ago. I will find my notes and exact solution and append this post soon.

11.32685 feet. The first plank on the outside edges 7.62254 and 6.47279 feet from the outside corner. The second plank has one end on the first 4.18969 from the smaller angle and the other on the bank. The third plank has one end 4.66891 from the end of the second and the other at the inside corner of the ditch.