Point inside a rectangle
Consider rectangle
A
B
C
D
with a point
P
inside it such that
A
P
=
7
,
B
P
=
1
5
, and
C
P
=
2
4
as shown in the figure.
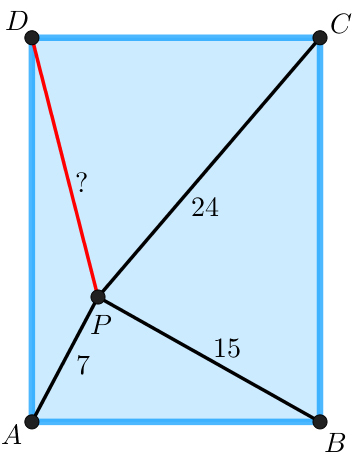 The figure is not drawn to scale.
The figure is not drawn to scale.
What is the length of segment D P ?
The answer is 20.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Let A B = C D = a and A D = B C = b , and the coordinates of A ( 0 , 0 ) and P ( x , y ) . Then the coordinates of B ( a , 0 ) , C ( a , b ) and D ( 0 , b ) . By Pythagorean theorem :
⎩ ⎪ ⎪ ⎪ ⎨ ⎪ ⎪ ⎪ ⎧ A P 2 = x 2 + y 2 = 4 9 B P 2 = ( a − x ) 2 + y 2 = 2 2 5 C P 2 = ( a − x ) 2 + ( b − y ) 2 = 5 7 6 D P 2 = x 2 + ( b − y ) 2 . . . ( 1 ) . . . ( 2 ) . . . ( 3 ) . . . ( 4 )
We note that ( 3 ) − ( 2 ) + ( 1 ) = ( 4 ) or C P 2 − B P 2 + A P 2 = D P 2 . ⟹ A P 2 + C P 2 = B P 2 + D P 2 or British flag theorem as mentioned by @Guillermo Templado .
Therefore, D P 2 = 5 7 6 − 2 2 5 + 4 0 = 4 0 0 , ⟹ D P = 2 0 .
Use British Flag Theorem D P = 7 2 + 4 9 2 − 1 5 2 = 4 0 0 = 2 0
Using the British Flag Theorem , we have
( P A ) 2 + ( P C ) 2 = ( P B ) 2 + ( P D ) 2
7 2 + 2 4 2 = 1 5 2 + ( P D ) 2
4 9 + 5 7 6 = 2 2 5 + ( P D ) 2
( P D ) 2 = 4 0 0
P D = 4 0 0 = 2 0