Points of Non-Differentiability

Calculus Level 5

f ( x ) = x 2 − ∣ x 2 − 1 ∣ + 2 ∣ ∣ x ∣ − 1 ∣ + 2 ∣ x ∣ − 7 f(x)=x^2-\mid x^2-1\mid+2\mid\mid x\mid-1\mid+2\mid x\mid-7

Number of points where the above function is non-differentiable is?

Try for some more interesting problems of Limits and Derivatives.


The answer is 0.

View wiki
Sandeep Bhardwaj by Sandeep Bhardwaj , 28, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Gagan Raj
Feb 15, 2015

The given function is differentiable at all points (Verified Graphically)

Hence f(x) has zero points of non - differentiability.

Hence the answer is 0.

Enjoy and Learn!!!!!

6 Helpful 0 Interesting 0 Brilliant 0 Confused

How do you know it is differentiable at all points?

Pi Han Goh - 6 years, 3 months ago

Yep I too have verified graphically. Can someone tell me how to do above question without using graph.

A Former Brilliant Member - 6 years, 3 months ago

Log in to reply

the proper way to solve this problem at those particular points, is as follows:
Say we take x = 1 x = 1 .
The Right hand derivative is 2 x − 2 x + 2 x + 2 x = 4 x 2x - 2x + 2x + 2x = 4x , which evaluates to 4.
The Left hand derivative is 2 x − ( − 2 x ) − 2 x + 2 x = 4 x 2x - (-2x) -2x + 2x = 4x , which evaluates to 4.
Hence the derivative at x = 1 x = 1 is 4.
Now, repeat this with x = 0 , − 1 x = 0, -1 .


Calvin Lin Staff - 6 years, 2 months ago

U can analyse for different cases and find it's gradient and you would see it cancels out

Julian Poon - 6 years, 3 months ago
Saharsh Rathi
Nov 4, 2016

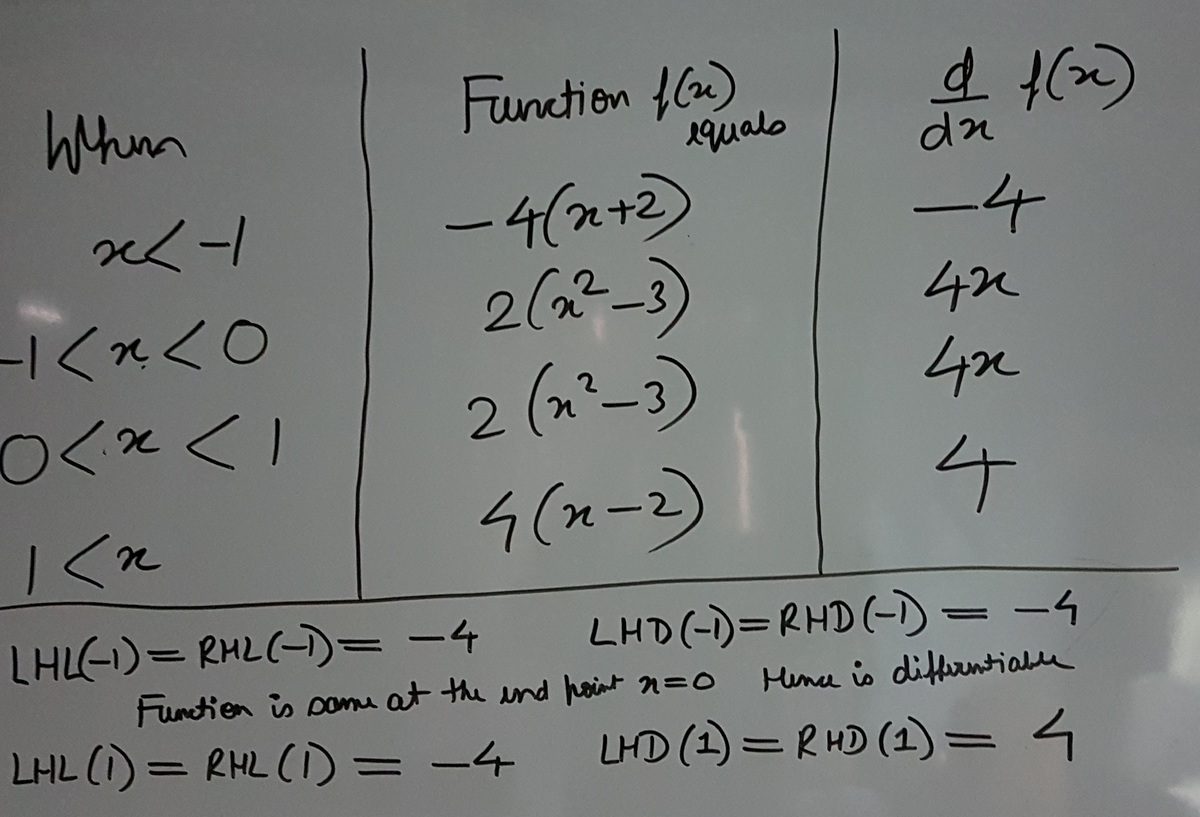
We only need to check differentiability at the end points .

5 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...