Pool Shark Shuffle
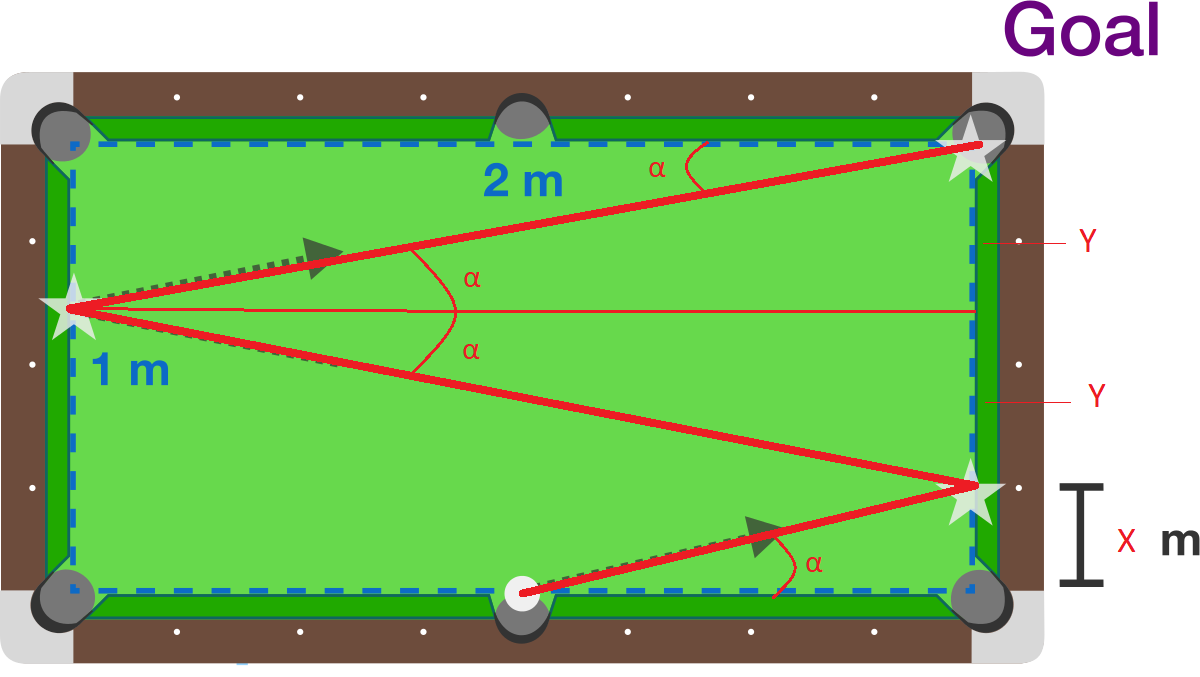
Shelley has a ball placed at the exact bottom middle of a 2 m by 1 m pool table as shown. She wants to hit the ball towards the right side of the table such that it bounces exactly 2 times and ends up in the upper right hole . How far up from the lower right corner should she shoot the ball?
This problem is part of the Brilliant.org Open Problems Group . The end goal for each open problem is to find a solution, and maybe publish it if it's a nice enough result! You can read about the unsolved billiards problem that this one is related to here .
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
13 solutions
Moderator note:
The "reflected pool table" perspective was optional for solving this problem, but if the shape is more complicated than a rectangle (like a triangle, in the first Intermediate and Advanced problems this week) reflections become much more useful; you can track many reflections on a complex figure just by drawing a straight line.
Nice job - reflecting the table was ‘brilliant’ !
Much more elegant than the solution I came up with (to get the same result)!
Such a good explanation! Well done!
sir could u pls explain why did u choose to visualize the ball travelling through reflected pool tables
Log in to reply
When the ball hits one of the side walls, it bounces off in a reflection, and although the actual path of the ball changes direction, its reflected path continues on in a straight line. Sometimes it is easier to view the path of a reflected object as one continuous segment rather than several connected segments (in this case it set up two proportional triangles), which is why I chose to visualize the ball travelling through reflected pool tables.
A beautiful solution!
it was seriously a brilliant approach
It's just a fabulous explanation........
My concern is regarding when reflection cannot be used. For example, what if the diagonal line drawn here had its needed 1m height (due to the height of the pool table) before it completes the two meters length of the pool table? Or vice versa? Is there a way that reflection is aways sucessful in these cases? If not, what similar approaches can one take? Thank you!
Log in to reply
If the diagonal line needed more height before it completed the two meters length, then it would bounce off the top bumper, in which case a vertical reflection could be used to model the problem. In this problem, however, the ball is only supposed to bounce once off the right wall and once off the left wall, so only a horizontal reflection (as pictured) is necessary.
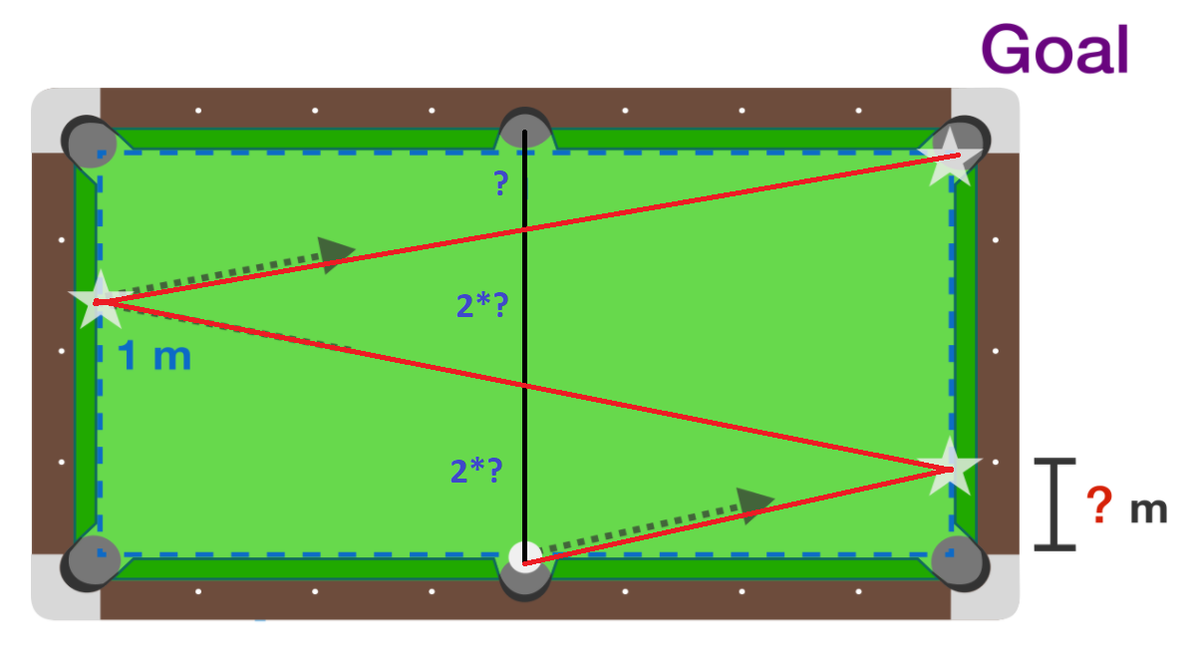
First, the ball goes towards the right side of the table, travelling ? m upwards. Because the ball is reflected back at the same angle, once it travels the same distance, the ball's vertical height will increase by the same amount. Therefore, when the ball returns back to the middle, it will have travelled an additional ? m upwards for a total of 2 ? m .
Once the ball goes to the left side of the table and returns to the middle again, it will increase by 2 ? m again. Finally, the hole will be on the right side of the table, so the ball's height will increase ? m . These heights total to 5 ? m , so ? = 5 1 m .
This made perfect sense to me. Thank you!
identical triangles! therefore the required distance ?m is equal to the distance from the top center pocket to the line-of-travel for a total of 5 ?m's,, and 1?m distance = 1/5m. I get it!
Log in to reply
The problem with this puzzle is that the solution only holds true if the ball is replaced by a beam of light and the cushions are mirrors. Anyone who has played on a surface such as a snooker table will know that on the first bounce the ball starts rotating clockwise and the effect of this is to reduce the reflection angle on the second cushion. A snooker player would have to put side on the cue ball to counter this effect. Over to you.....
If we consider the number of times the ball travels horizontally, it goes middle → right → middle → left → middle → right. This is a total of 5 movements, and the ball goes upwards by the same amount each time. Therefore, 5 times this upward amount = 1m, meaning 5 1 is the answer.
EDIT: For clarity, this pattern of movements only works for the middle of the table. If say, the ball was only a quarter of the width from the first bounce, it would be 1 + 4 + 4 = 9 movements
You have the right idea, but it needs to be better phrased/explained. For example, if we started "off-middle", we would still have the 5 movements of "offmiddle - right - offmiddle - left - offmiddle - right", but the answer would not be 1/5.
Log in to reply
I agree that this wouldn't work for "off-middle", yet the problem didn't state that, so I answered the problem where it was in the middle
Log in to reply
Right, so can you include the unwritten assumption of why this works for the middle? This will reduce the mystique of the solution.
I think there is some information missing because the way I thought it was the ball on the down right corner. I don't think this problem is explained clearly enough. I used guess and check to figure it out. I don't really understand what the instructions meant because here everyone has a different way of laying out different ways to solve the problem. I don't really know how I would rate this problem because it's confusing. I think the instructions weren't very clear.
Log in to reply
It says in the problem that the ball is in the bottom middle, not the bottom right. Even if there is confusion, the diagram is clear enough to deduce what the problem is asking for.
I worked it it rather simply thinking about gradients. for every X meter traveled how many Y meters need to be traveled?
1) we know that in the Y direction total distance traveled is 1
2) total X distance is is 5 meters (1 meter from start to right edge, 2 meters for left edge to right and 2 meters from right to left)
gradient = rise/run gradient = 1/5 ( for every 1 meter in Y, 5 must be traveled in X)
and so ans = 1/5*1m = 1/5m
Nice and elegant

We begin by assigning lengths x and ( 1 − x ) in the picture above. Note that we are asked to find length x .
Now, represent the path we would like the ball to travel with red lines (as shown in the picture below).
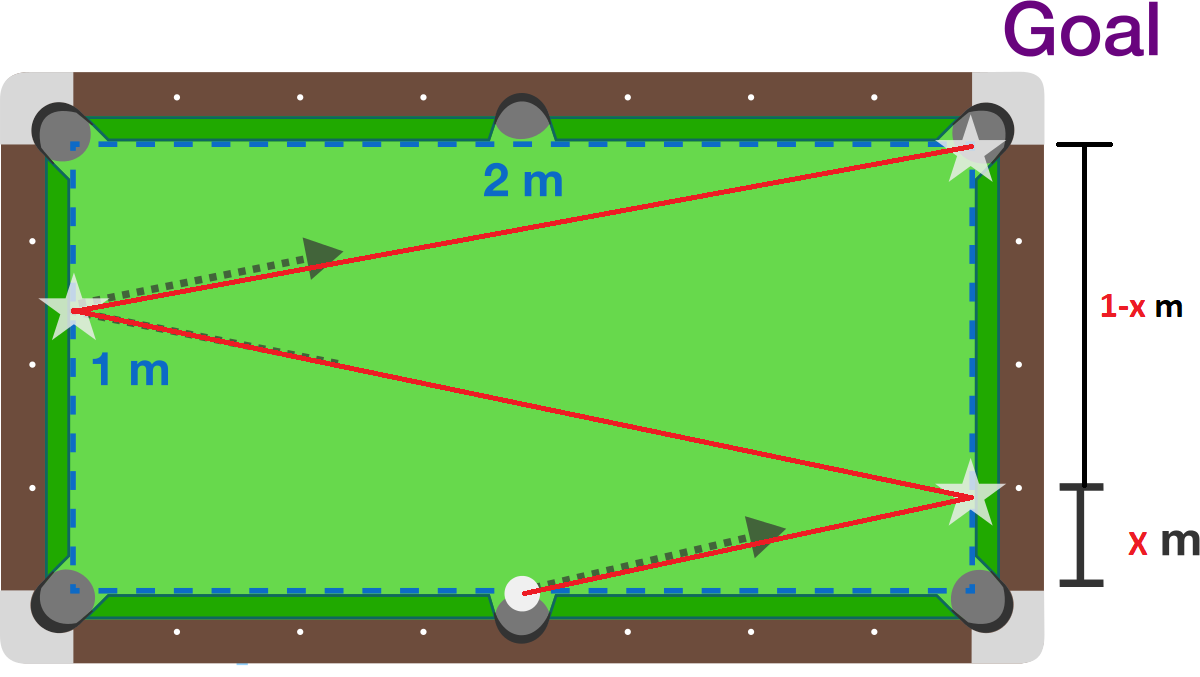
Notice how in the picture above, we have formed a right triangle and an isosceles triangle. We will split the isosceles triangle into two right triangles by inserting an angle bisector (see image below).
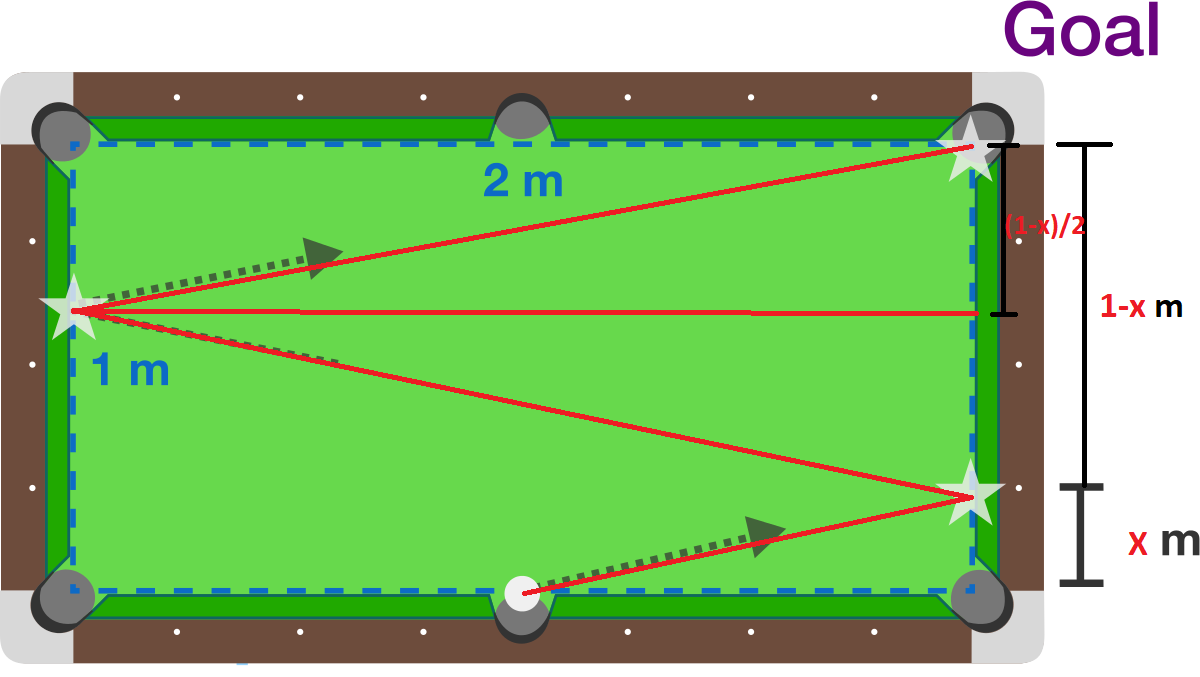
Note how the leg length of the top right triangle is now 2 ( 1 − x ) .
We will now assign letters to noteworthy points in the figure to make this solution easier to explain.
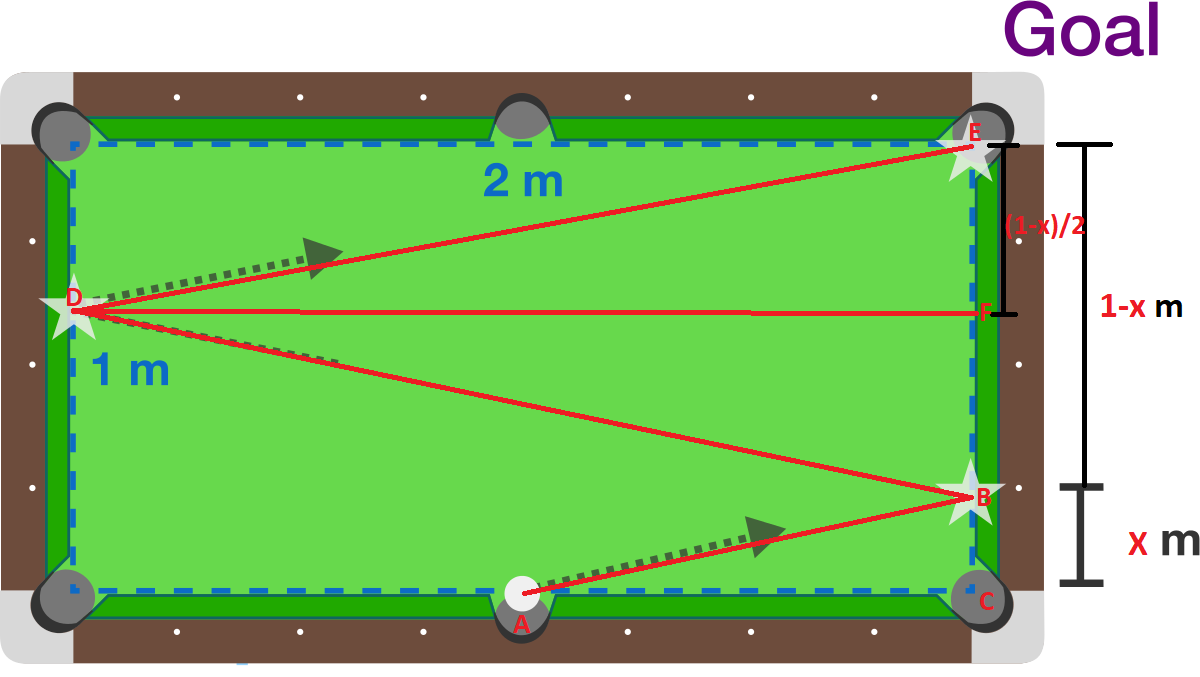
We now have everything we need to develop (and solve) a system of equations.
Triangle DEF has legs of length 2 m and length 2 ( 1 − x ) .. We will use the pythagorean theorem to show that ( 2 ) 2 + ( 2 1 − x ) 2 = y 2 where y is the length of the hypotenuse of Triangle DEF.
Now, let's look at Triangle ABC. Length AB is parallel to length DE; however, length AB is only half as long. Since length DE was assigned length
y
, we must assign length AB with a length of
2
y
The image below depicts the current situation.

Note that the legs of triangle ABC are length x and length 1m. We will use the pythagorean theorem to show that 1 + x 2 = 4 y 2 .
We now have two equations:
Equation 1 . from Triangle DEF: ( 2 ) 2 + ( 2 1 − x ) 2 = y 2
Equation 2 . from Triangle ABC: 1 + x 2 = 4 y 2
Equation 2 can be modified by multiplying each side of the equation by 4. The result is equation 3 : 4 + 4 x 2 = y 2
Since the left sides of equations 1 and 3 are both equal to y 2 , we will now set them equal to each other as follows:
( 2 ) 2 + ( 2 1 − x ) 2 = 4 + 4 x 2
A little bit of algebra and simplification yields the following quadratic equation: 1 5 x 2 + 2 x − 1 = 0
The roots of the equation are ( − 1 / 3 ) and ( 1 / 5 ) . In this context, a negative solution is nonsensical. Therefore, the correct solution is x = 1 / 5 meters.
Great job on the analytical solution
 In pool, the angle of incidence needs to equal the angle of reflection, or there'd be something wrong with the pool table itself. From the illustration, we see the first angle the ball takes when hitting the first bumper. Let's call that x. When the ball rebounds, we see it makes twice the original angle, or 2x, since it makes the first angle out the same angle it went in, which is equal to the angle the ball was shot at , or x. It then goes to the other side, and makes another double angle against the bumper over there, or 2x. Altogether, there are x+2x+2x, or 5 equal angles, requiring the ball to be shot at 1/5th the width of the table (1 meter), in order to fall in the upper right pocket. Thus, the answer as to where to aim one's shot to make the ball go the desired path would be at the 1/5th meter mark.
☺☺☺☺
In pool, the angle of incidence needs to equal the angle of reflection, or there'd be something wrong with the pool table itself. From the illustration, we see the first angle the ball takes when hitting the first bumper. Let's call that x. When the ball rebounds, we see it makes twice the original angle, or 2x, since it makes the first angle out the same angle it went in, which is equal to the angle the ball was shot at , or x. It then goes to the other side, and makes another double angle against the bumper over there, or 2x. Altogether, there are x+2x+2x, or 5 equal angles, requiring the ball to be shot at 1/5th the width of the table (1 meter), in order to fall in the upper right pocket. Thus, the answer as to where to aim one's shot to make the ball go the desired path would be at the 1/5th meter mark.
☺☺☺☺
Yes, identical triangles.
If we visualize the ball direction to the goal, we can realize that the angle (α) with which the ball bounces in the sides of the table is the same:
With this reasoning we can formulate 3 equations: Tan α = 1 x // Tan α = 2 y // 2y + x = 1 //. Equaling the 2 first we obtain the following wquation system: y = 2x // 2y + x =1 // And solving this we have: 4x + x =1 ➞ 5x = 1 ➞ x = 5 1
Simple, beautiful
Let α be the shot angle with respect to the horizontal where an angle of 0 degrees would be rightward.
Let x 0 be the value in meters of the first bounce (the answer we seek).
These are related by x 0 = t a n ( α ) .
Given a sensible law of reflection and some trig, we can write a formula for the vertical position of all future bounces x n = ( 2 n + 1 ) t a n ( α ) .
x 2 (n=2) is where the ball falls into the hole (instead of bouncing) and we know its value in meters is 1 = x 2 = 5 t a n ( α ) and we can solve for α = t a n − 1 ( 5 1 )
Therefore, x 0 = tan ( t a n − 1 ( 5 1 ) ) = 5 1 .
I solved it using basic trigonometry.

The tangent of angle a = x 1 The tangent of angle b = ( 1 − x ) / 2 2 Now angle a = angle b. So we can say that: x 1 = ( 1 − x ) / 2 2 ie x 1 = ( 1 − x ) 4 ie 1 - x = 4x so therefore x = 5 1
We know 1m is width and 2m is length. The ball hits the 1m side at an angle then spans the length of the pool table twice. 2 is twice as large as 1. So 1/5 of the 1m at first hit, then by the time u get to your second hit, u will have covered 3/5 total is the width. And lastly as the ball is going back the same distance toward the goal it will complete 5/5 or the whole width of the table. If it we're a square (having equal sides) then each of the 2 hits will only cover 1/3 of the table. 1/x + 1/2x + 1/2x = 1 can be used to find what fraction of the width u have to hit
I was really confused for a moment because this quiz was trying to pocket the cue ball...

We can visualize the ball travelling in a straight line through a series of reflected pool tables, as shown below.
To reach the goal, the ball would need to travel 1 meter in a vertical direction and 5 meters in a horizontal direction at a 1:5 ratio. Therefore, since the ball hits the right side of the table after it has traveled 1 meter in the horizontal direction, the missing vertical direction can be found by solving the proportion 5 1 = 1 x , which is x = 5 1 meters.