Present Mystery
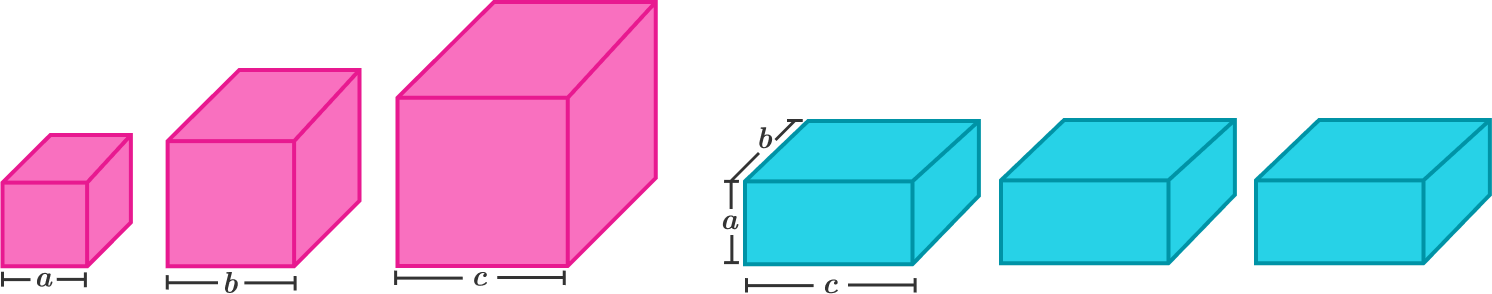
a , b , c ( a < b < c ) are coprime integers, and there are the following 2 sets of present boxes:
- 3 pink cubic boxes of respective side lengths a , b , c
- 3 identical blue cuboid boxes of dimensions a × b × c .
Which set has a larger total surface area?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
7 solutions
Whyy when a=1,b=2,and c=3 the blue is almost big
Log in to reply
No it isn't. I think you are calculating the volume. 😜
The problem can be written as comparing 6 a 2 + 6 b 2 + 6 c 2 (LHS) and 6 a b + 6 b c + 6 c a (RHS). Dividing 3 out and subtracting LHS-RHS, the remaining expression is 2 a 2 + 2 b 2 + 2 c 2 − 2 a b − 2 b c − 2 c a , which can be expressed as ( a − b ) 2 + ( b − c ) 2 + ( c − a ) 2 , obviously ≥0. Therefore, the LHS is greater, so pink has the bigger surface area. Equality occurs when a=b=c
According to AM -GM inequality, a^3 + b^3+ c^3 ≥ 3 ∛a^3b^3c^3 ⇒ a^3 + b^3+ c^3 ≥ 3 abc ⇒Sum of volumes of pink boxes ≥ Sum of volumes of blue boxes
I think you misunderstood the problem... It asks for the total surface, not the total volume
This is WRONG!!
Surface area of the three cubes= 6 a 2 + 6 b 2 + 6 c 2
surface area of the three cuboids= 3(2ab +2bc+ 2ac)= 6(ab+bc+ac)
Now according to cauchy shwarz inequality:
( a 2 + b 2 + c 2 ) ( 1 2 + 1 2 + 1 2 ) > = [ a ( 1 ) + b ( 1 ) + c ( 1 ) ] 2
3 ( a 2 + b 2 + c 2 ) > = ( a + b + c ) 2
3 a 2 + 3 b 2 + 3 c 2 > = a 2 + b 2 + c 2 + 2 ( a b + b c + a c )
2 ( a 2 + b 2 + c 2 ) > = 2 ( a b + b c + a c )
multiplying 3 on both sides:
6 ( a 2 + b 2 + c 2 ) > = 6 ( a b + b c + a c )
therefore total surface area of the three cubes is greater than the total surface area of the three cuboids.
As A M ≥ G M and a = b = c from the fig.,
a 2 + b 2 > 2 a b
b 2 + c 2 > 2 b c
a 2 + c 2 > 2 a c
Adding them up,
⟹ 2 ( a 2 + b 2 + c 2 ) > 2 a b + 2 b c + 2 a c
⟹ 6 a 2 + 6 b 2 + 6 c 2 > 3 ( 2 a b + 2 b c + 2 a c )
∴ a a a a : S . A . ( Cubes ) > S . A . ( Cuboids )
By the AM-GM inequality $a^{3}+b^{3}+c^{3}>3abc$
Taking the two sequences $(a,b,c)$
According to Cauchy-Schwarz inequality , ( a 2 + b 2 + c 2 ) ( b 2 + c 2 + a 2 ) ≥ ( a b + b c + c a ) 2 .
Hence, a 2 + b 2 + c 2 ≥ a b + b c + c a .
Then, 6 a 2 + 6 b 2 + 6 c 2 ≥ 3 ( 2 a b + 2 b c + 2 c a ) .
The total surface area of all pink cubes = 6 a 2 + 6 b 2 + 6 c 2 , and the total surface area of all blue cuboids = 3 ( 2 a b + 2 b c + 2 c a ) .
Since a , b , c are co-prime integers, the ratios a : b = b : c = c : a .
As a result, such inequality can not become equality, and the pink cubes will always have larger total surface area than the blue ones.