Prime Square
A red rectangle is reshaped into a blue square, as shown below, such that both quadrilaterals have the same perimeter . The area of the square is larger than that of the rectangle.
If all the sides of both quadrilaterals have
prime number
lengths
in
then what is the perimeter of the square?
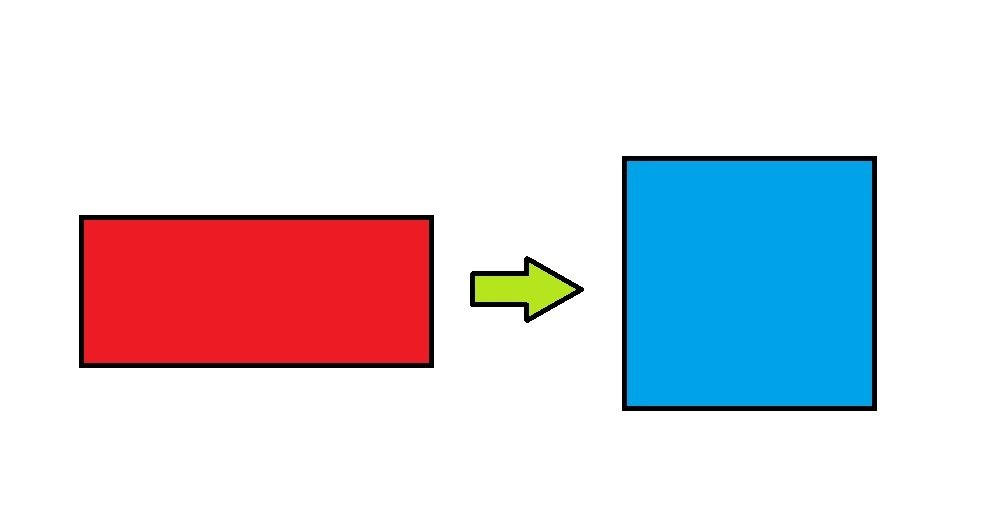
The answer is 20.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Relevant wiki: Perimeter
Let x and y be the side lengths of the rectangle. Hence, the square's side length = 2 x + y .
Then the rectangle's area = x y , and the square's area = 4 ( x + y ) 2 .
Thus, 4 ( x + y ) 2 − x y = 4 ( x 2 + 2 x y + y 2 ) − 4 x y = 4 ( x − y ) 2 = 4 .
Taking square roots both sides, we will get: 2 ∣ x − y ∣ = 2 . ∣ x − y ∣ = 4 .
In other words, supposedly if x is the shorter side length of the rectangle, then x + 2 is the side length of the square and x + 4 is the longer side length of the rectangle.
Since all these lengths are prime integers, only the triple 3 , 5 , 7 applies because in the higher triple range, one of the three numbers will fall upon the multiple of 3 .
As a result, the square's perimeter = 4 × 5 = 2 0 .
Alternatively, the rectangle's perimeter = 2 × ( 3 + 7 ) = 2 0 .