Two Random Numbers

Probability Level 3

Let A A and B B be two numbers randomly chosen between 0 0 and 1 1 .

Find the probability of having an absolute difference greater than 1 3 \frac{1}{3} between the two numbers.

5 9 \frac59 1 3 \frac13 4 9 \frac49 1 2 \frac12

View wiki
Jonathan Dou by Jonathan Dou , 20, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Jonathan Dou
Dec 27, 2016

Let A and B be x and y on a 2D graph respectively, if we plot out all the favorable points we get the graph below: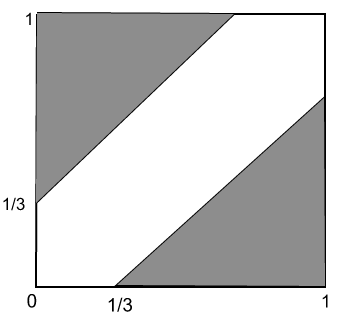 The favorable points lie in the shaded areas and we can find the probability of getting a favorable point by solving for the area which is 4 9 \frac{4}{9}

2 Helpful 0 Interesting 0 Brilliant 0 Confused

I love geometric probability approaches.

Calvin Lin Staff - 4 years, 5 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...