This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
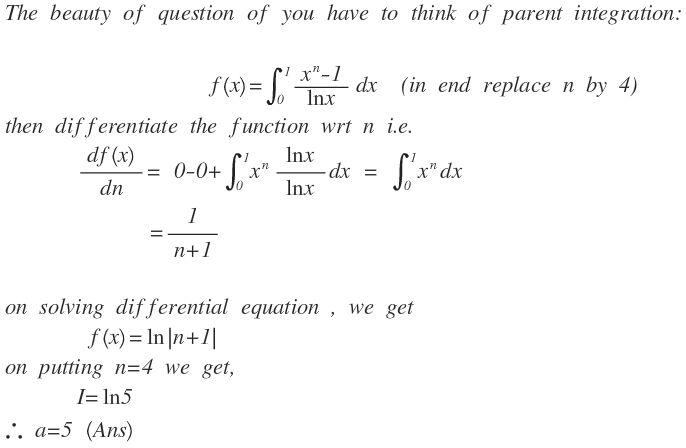
Why did you take n=4 ? @Yash Dev Lamba
Log in to reply
first I sustitute 4 as n then i back substitute n=4 see in question power of x is 4 which i take as n for differention
For your answer to be correct,you need to show that the value of the arbitrary constant obtained in the solution of the differential equation is 0.
If we define F ( a ) = ∫ 0 1 ln x x a ( x 4 − 1 ) d x , a > − 1 , then F ′ ( a ) = ∫ 0 1 x a ( x 4 − 1 ) d x = a + 5 1 − a + 1 1 , a > − 1 , and hence F ( a ) = c + ln ( a + 5 ) − ln ( a + 1 ) = c + ln ( a + 1 a + 5 ) , a > − 1 . The function ln x x 4 − 1 is integrable over ( 0 , 1 ) and so, using the Dominated Convergence Theorem, it is clear that a → ∞ lim F ( a ) = 0 , and hence c = 0 , so that F ( a ) = ln ( a + 1 a + 5 ) . We are asked to evaluate the integral F ( 0 ) = ln 5 , so the answer is 5 .
U can do this in ur head..so easy