Projectile Motion Comparison - 4

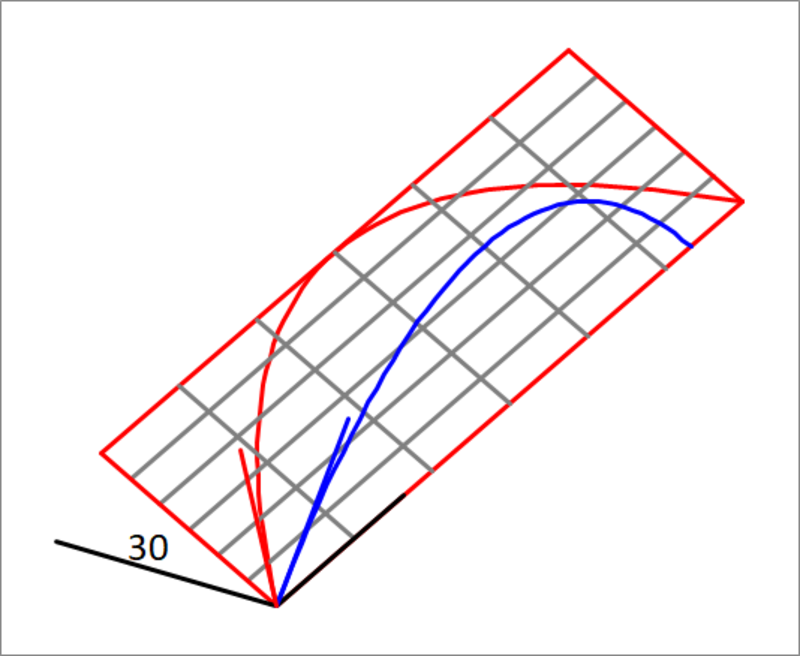
Classical Mechanics Level pending

Two solid balls are thrown from the same point with the same linear speed of their centers, the first freely at an angle of $45^{\circ}$ with the horizontal, and the second is sent on an inclined plane, with its initial velocity vector making an angle of $45^{\circ}$ with the horizontal edge of the plane. The second ball is purely rolling on the inclined plane. How do the maximum altitudes of the two balls compare ? The inclined plane makes an angle of $30^{\circ}$ with the horizontal ground. If $H_1$ is the maximum altitude of the first ball, and $H_2$ is the maximum altitude of the second ball, what is the relation between $H_1$ and $H_2$ ?

The attached figure depicts the two projectiles. The figure is not drawn to scale.

H_2 = 1.4 H_1

H_2 = H_1

H_2 = 2 H_1

H_2 = 2.5 H_1

1 solution

A Former Brilliant Member
May 19, 2020

First case :

$H_1=\dfrac{u^2}{4g}$ .

Second case :

Acceleration is $\dfrac{5g}{14}$ along the line of greatest slope of the inclined plane.

Component of initial velocity along this line is $\dfrac{u}{\sqrt 2 }$ and

along the horizontal line is also $\dfrac{u}{\sqrt 2 }$ .

So, $H_2=\dfrac{\frac{u^2}{2}}{2\times \frac{5g}{14}}\times \sin 30\degree=\dfrac{7u^2}{20g}$ .

Therefore $\boxed {H_2=1.4H_1}$

Solution to the fifth problem of this series :

$R_1=\dfrac{u^2}{g}$ .

Since the kinetic energies in the two cases are the same, $v^2=\frac{5}{7}u^2$ , where $v$ is the initial velocity in the second case.

So, $R_2=\dfrac{v^2}{\frac{5g}{14}}=\dfrac{5}{7}u^2\times \dfrac{14}{5g}=2\dfrac{u^2}{g}$ .

Therefore $\boxed {R_2=2R_1}$

Your problems are really good Hosam Hajjir

Projectile Motion Comparison - 4

1 solution

0 pending reports