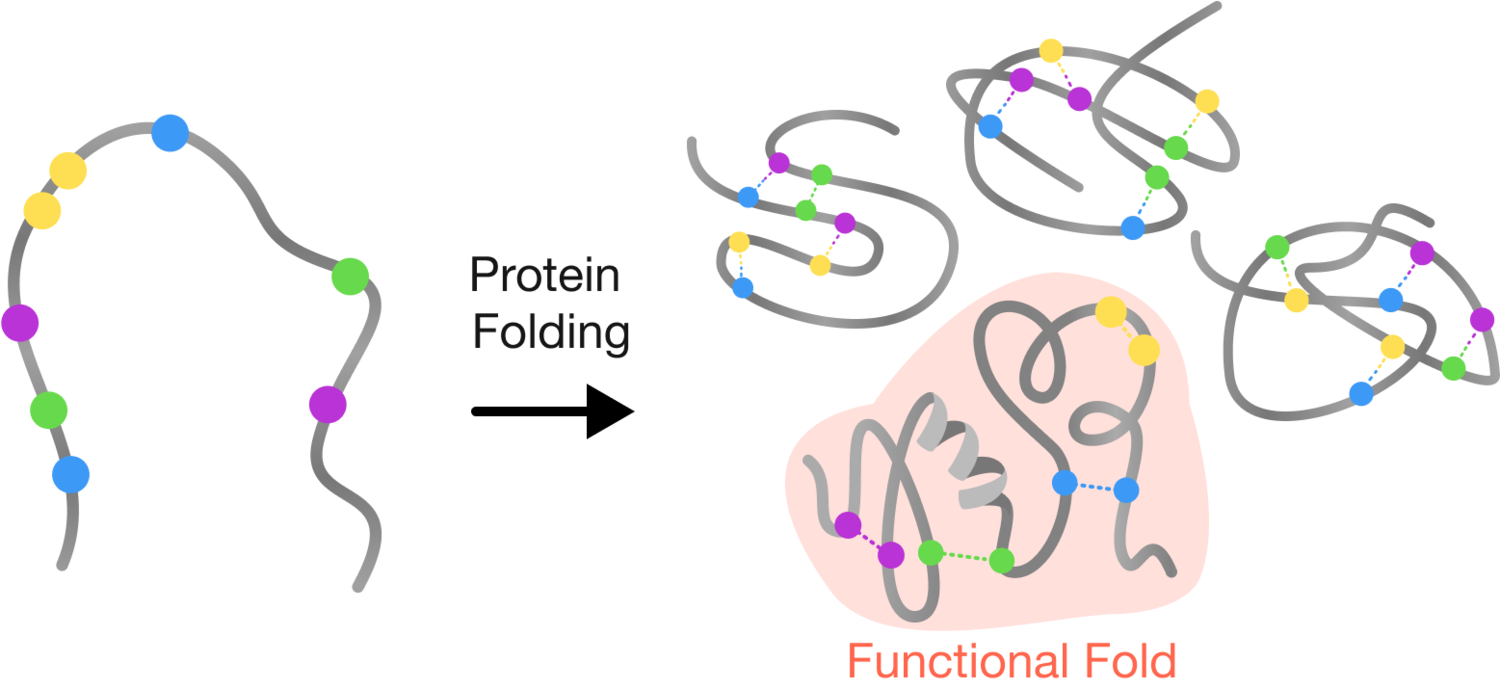
Protein Origami

Pick any particular cysteine. It has to be bonded with just one other cysteine, and it has to be the correct one. There is $\frac17$ chance that it is so since there are $7$ possibilities.

This takes care of two of the cysteines. Pick any of the remaining six. This cysteine too has to be bounded with the right one, and the chance of that is $\frac15$ .

Selecting one of the remaining $4$ to consider, it has $\frac13$ chance of being connected correctly.

Finally, the last two have to be correct, if all else is. So the chance of them being right is $1$ .

The probability of all the bonds being correct simultaneously is the product, that is $\frac17\cdot\frac15\cdot\frac13\cdot1=\boxed{\frac1{105}}$

There are $8!$ $($ where the $!$ symbol denotes the factorial $)$ ways to pair up the $8$ cysteine amino acids. This number of ways includes different orderings of the same two cysteines, so we must divide by 2 because the ordering of the $C$ amino acids in each pair is unimportant. We must divide by $4!$ because the ordering of the pairs as a whole is unimportant to the structure of the protein.

Thus, the number of arrangements is given by $\frac{8!}{2^4\times 4!} = 105.$

If each protein folds randomly, we'd expect only $\frac{1}{105} < 1\%$ of the proteins to find this functional fold. In fact, basically $100\%$ of ribonuclease enzymes fold into the correct arrangement. How proteins stumble onto their so-called "native" fold is still a field of active study.

The number of perfect matchings of a complete graph $K_n$ for $n$ even is given by $(n-1)!!$ where $!!$ stands for the double factorial .

So here, for eight nodes, we have $7!!=7*5*3*1=105$ possible matchings and only one of them yields a correct folding. Hence the fraction is $\boxed{\displaystyle\frac1{105}}$

Consider all the cysteines.

When there are 8 cysteines, we can choose whatever you want as a beginning and then there is a only one can fit it. For $\frac{1}{7}$ chance.

And there are 6 cysteines left, we still can choose one randomly, after that a only one fit it, the possibility is $\frac{1}{5}$ .

The same situation when 4-3 cysteines and 2-1 cysteines.

So we can list one expression as follow

1* $\frac{1}{7}$ * 1 * $\frac{1}{5}$ * 1 * $\frac{1}{3}$ * 1 * $\frac{1}{1}$ = $\frac{1}{105}$

Events:

• First. The probability of selecting the first cysteine is 1 because you can pick up any of them.
• Second. The probability of selecting its only partner is $\frac{1}{7}$ because you need to pick the exact one you need in seven options you have.
• Third. The probability of selecting the third cysteine is 1 because you can pick up any of the six remaining.
• Fourth. The probability of selecting the only partner of the third random cysteine is $\frac{1}{5}$ .because you need to pick the exact one you need in five options remaining.

Now we can see how the events to follow have to happen if we want only one configuration, and because each one of the events is independent we can multiply their probability to obtain the probability of the composite event which will mean a certain arrangement.

$1*\frac{1}{7}*1*\frac{1}{5}*1*\frac{1}{3}*1*\frac{1}{1}=\frac{1}{105}$

In general, we can calculate fractions of these kind as follows: $\frac{NumberOfWantedCombinations}{NumberOfPossibleCombinations}$

Now, lets imagine the bonds as a "chain". Two cysteine bond together if they are right next in the chain.

For the number of possible combinations we just have 8 factorial since there are 8 cysteines whose arrangement doesn't matter (yet).

To find the number of wanted combinations is a bit trickier:

For first one in the "chain" we can pick any of the cysteines.

For the second one we only have one choice: The other cysteine of the same kind.

For the third one we can pick any of the remaining ones and so on.

That leaves us with the following fraction: $\frac{8*1*6*1*4*1*2*1}{8!}$ = $\frac{1}{105}$