Pumpkin Volume 12
Dedicated to my grandson Fedor.
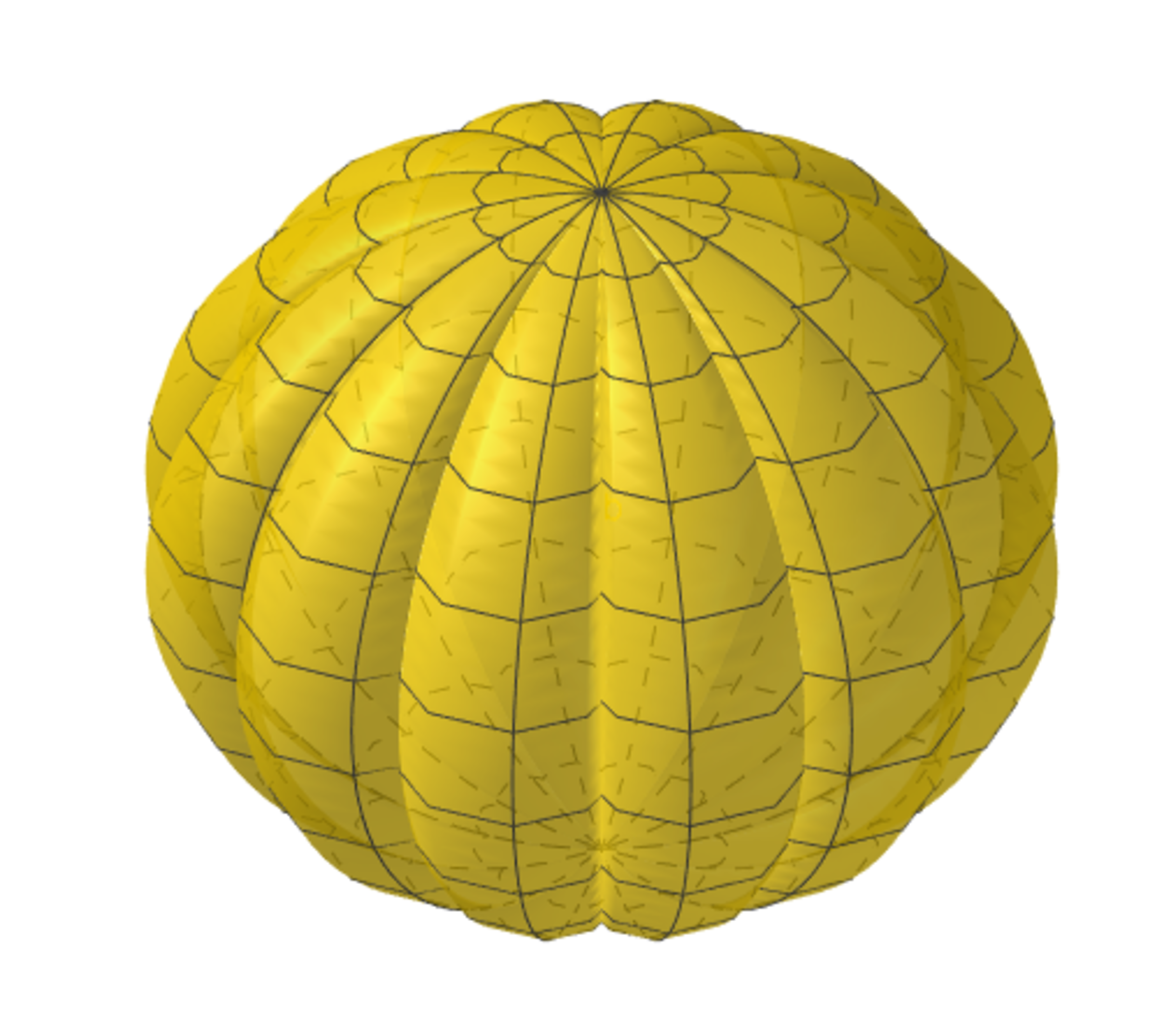
The parametric equations of a pumpkin surface with n ridges are given to be:
X ( p , t , n ) Y ( p , t , n ) Z ( p , t , n ) = = = R sin ( p ) n ( n + 1 ) cos ( t ) − cos ( t ( n + 1 ) ) R sin ( p ) n ( n + 1 ) sin ( t ) − sin ( t ( n + 1 ) ) h cos ( p ) , where 0 ≤ t ≤ 2 π and 0 ≤ p ≤ π .
Define V p ( R , h , n ) as the pumpkin's volume, and V s ( R ) = 3 4 π R 3 .
If the value of V s ( R ) V p ( R , R , 1 2 ) can be expressed as B A , where A and B are coprime positive integers, submit your answer as A + B .
The answer is 163.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
@Mark Hennings , we really liked your comment, and have converted it into a solution.
What does n represent here?
Where can I find more information about the elementary volume element ratio?
Log in to reply
A better name for it is the Jacobian ∂ ( r , p , t ) ∂ ( x , y , z )
I guess I need to review calculus.
With R = h we have the parametrization r = ( r sin p n ( n + 1 ) cos t − cos ( ( n + 1 ) t ) , r sin p n ( n + 1 ) sin t − sin ( ( n + 1 ) t ) , r cos p ) and hence we have the elementary volume element ratio X ( r , p , t ) = ∣ ∣ ∣ ∣ ∂ r ∂ r ⋅ ( ∂ p ∂ r × ∂ t ∂ r ) ∣ ∣ ∣ ∣ = n 2 2 ( n + 1 ) ( n + 2 ) r 2 sin p sin 2 2 1 n t so the pumpkin volume is V p ( R , R , n ) = ∫ 0 R d r ∫ 0 π d p ∫ 0 2 π d t X ( r , p , t ) = 3 n 2 4 ( n + 1 ) ( n + 2 ) π R 3 so that V s ( R ) V p ( R , R , n ) = n 2 ( n + 1 ) ( n + 2 ) This makes the correct answer 1 4 4 1 3 × 1 4 = 7 2 9 1 , so the desired answer is 1 6 3 .