Pure geometry topics application for JEE

surely not a level 5 problem, can be solved mentally with in 15 sec.

the graph of $sinx$ between $[0,\pi]$ is symmetrical about $x=\frac{\pi}{2}$

and $\frac{\pi}{2}=1.507$

also $\sqrt{2} =1.414$ and $\sqrt{3}=1.732$

we see that 1.414 is closer to 1.507 than 1.732, so $sin(\sqrt{2})>sin(\sqrt{3}$

similarly from graph $cos(\sqrt{3})=negative$ $value$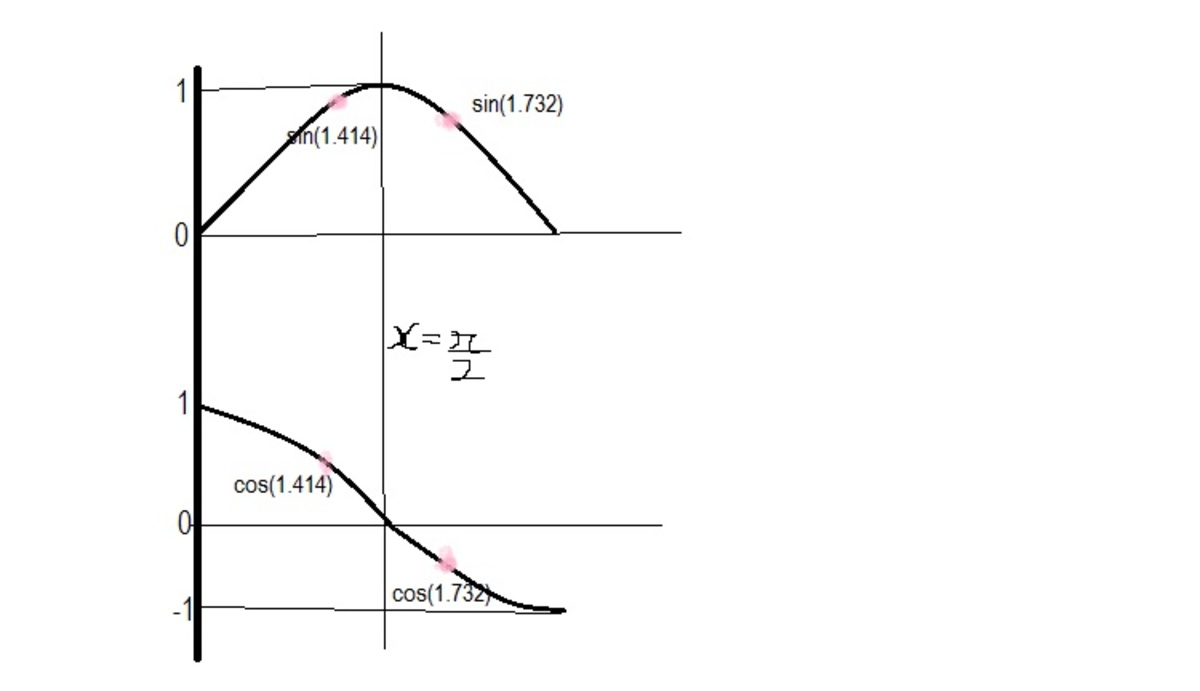

the denominator of $y^2$ is greater as the are added up ,so the answer follows an ellipse with focii on y axis

do upvote