Putnam - Q6 - Use your intuition

Probability Level 1

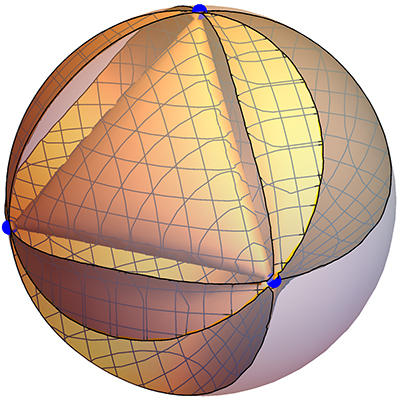
If four points are randomly selected on the surface of a sphere and then connected internally , then what is the probability percent that the center of the sphere eventuate in the tetrahedron formed by the connected points?

15 10 25 12.5

A Former Brilliant Member by A Former Brilliant Member

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Consider a 2-D representation of the the sphere, a circle with three points randomly selected on it.

  • If you fix any two points, then you've fixed the region where the third point have to eventuate for the condition to be satisfied.
  • You also have only half the perimeter of the circle where the two points can occur - [0-180°]
  • The average of all the possible points A(a, b) and B(c, d) fixed for the third point C(e, f) to occur in the arc of love is 1 4 \frac{1}{4} (Think about it a little bit).

The idea generalizes to other dimension too.

Now you're ready to solve it yourself. You may wanna use linear algebra for a rigorous interpretation.

2 Helpful 0 Interesting 0 Brilliant 0 Confused

See the solution in the 3Blue1Brown channel in YouTube.

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...