Pythagorean Cross Section
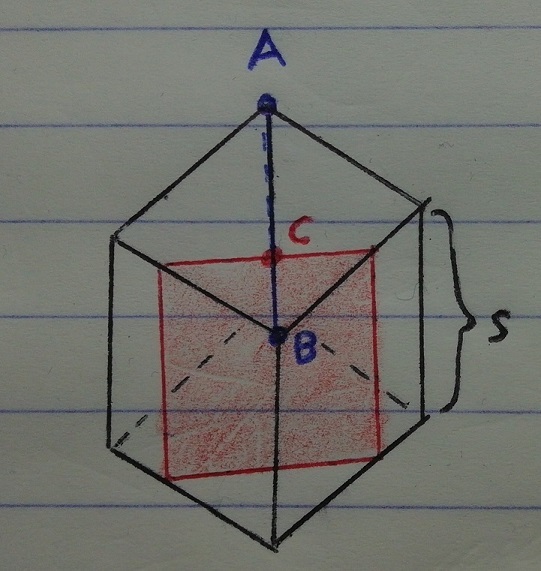
A cross section is made inside a cube such that its vertical sides are parallel to the vertical edges of the cube and its top side is perpendicular to the segment A B , at a distance from point B such that the cross section has an area equal to the face of the cube as shown below. Point C is put on the intersection of the top side of the cross section and segment A B .
What is the ratio of
A
B
B
C
?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Nice solution! Much simpler than mine!
We're trying to find
A
B
B
C
, so let's find
A
B
first. We can call the side of the cube
s
. Using the pythagorean theorem, we know that:
s
2
+
s
2
=
(
A
B
)
2
⟹
2
s
2
=
(
A
B
)
2
⟹
2
s
=
A
B
So we have our answer for
A
B
in terms of
s
. Now onto
B
C
.
Let's illustrate the top face of the cube and label a couple things to make notation easier.
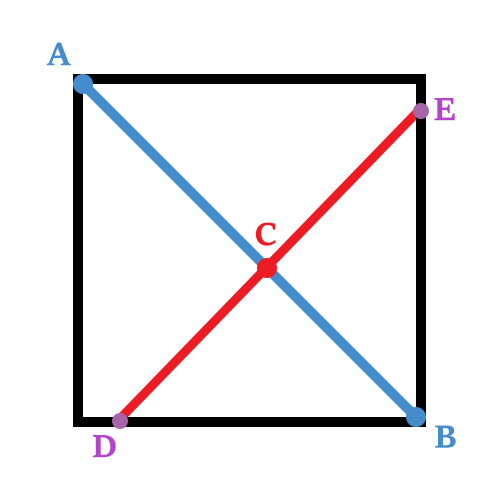 The distance from the top side of the cross section (that is,
D
E
) to
B
should be such that the whole cross section's area is equal to the face of the cube. Since the cross section is completely vertical, that can only happen when
D
E
=
s
. And since
D
E
is perpendicular to
A
B
,
D
B
and
B
E
must be equal. Let's call them both
t
for simplicity.
The distance from the top side of the cross section (that is,
D
E
) to
B
should be such that the whole cross section's area is equal to the face of the cube. Since the cross section is completely vertical, that can only happen when
D
E
=
s
. And since
D
E
is perpendicular to
A
B
,
D
B
and
B
E
must be equal. Let's call them both
t
for simplicity.
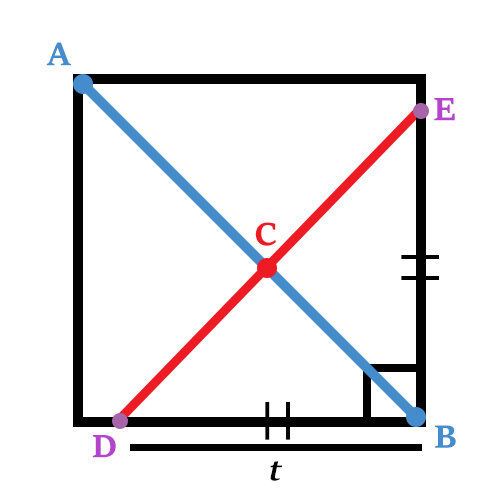 But what is
t
? Notice
t
is the side of a right triangle with hypotenuse
s
. We can use the pythagorean theorem again to find
t
in terms of
s
.
t
2
+
t
2
=
s
2
⟹
2
t
2
=
s
2
⟹
2
t
=
s
⟹
t
=
2
2
s
Notice that
B
C
is part of a
t
-sided right triangle, more specifically,
B
C
is the
height
of the right traingle. And since
B
C
is perpendicular to
D
E
we can use the
inverse
pythagorean theorem and substitute
t
to find
B
C
:
t
2
1
+
t
2
1
=
(
B
C
)
2
1
⟹
(
2
2
s
)
2
1
+
(
2
2
s
)
2
1
=
(
B
C
)
2
1
(
4
2
s
2
)
1
+
(
4
2
s
2
)
1
=
(
B
C
)
2
1
⟹
2
s
2
4
+
2
s
2
4
=
(
B
C
)
2
1
⟹
2
s
2
8
=
s
2
4
=
(
B
C
)
2
1
4
s
2
=
(
B
C
)
2
⟹
2
s
=
B
C
Now that we have both
A
B
=
2
s
and
B
C
=
2
s
it's time to find
A
B
B
C
.
A
B
B
C
=
2
s
2
s
=
2
s
×
2
s
1
=
2
2
s
s
=
4
s
2
s
=
4
2
Not the most elegant or efficient solution, I know. This problem came to mind and this is how I solved it. I'm excited to see your own solutions!
But what is
t
? Notice
t
is the side of a right triangle with hypotenuse
s
. We can use the pythagorean theorem again to find
t
in terms of
s
.
t
2
+
t
2
=
s
2
⟹
2
t
2
=
s
2
⟹
2
t
=
s
⟹
t
=
2
2
s
Notice that
B
C
is part of a
t
-sided right triangle, more specifically,
B
C
is the
height
of the right traingle. And since
B
C
is perpendicular to
D
E
we can use the
inverse
pythagorean theorem and substitute
t
to find
B
C
:
t
2
1
+
t
2
1
=
(
B
C
)
2
1
⟹
(
2
2
s
)
2
1
+
(
2
2
s
)
2
1
=
(
B
C
)
2
1
(
4
2
s
2
)
1
+
(
4
2
s
2
)
1
=
(
B
C
)
2
1
⟹
2
s
2
4
+
2
s
2
4
=
(
B
C
)
2
1
⟹
2
s
2
8
=
s
2
4
=
(
B
C
)
2
1
4
s
2
=
(
B
C
)
2
⟹
2
s
=
B
C
Now that we have both
A
B
=
2
s
and
B
C
=
2
s
it's time to find
A
B
B
C
.
A
B
B
C
=
2
s
2
s
=
2
s
×
2
s
1
=
2
2
s
s
=
4
s
2
s
=
4
2
Not the most elegant or efficient solution, I know. This problem came to mind and this is how I solved it. I'm excited to see your own solutions!
AB = s sqrt(2). (BC)^2 = (S/sqrt(2))^2 - (S/2)^2 = S^2/2 - S^2/4 = S^2/4, so BC =S/2. Then CB/AB = (S/2)/(S sqrt(2) = 1/(2*sqrt(2)) = sqrt(2)/4.
Let the side of the cube be s . Since B C is the same as half a side, B C = 2 1 s , and since A B is the diagonal of a square with side s , A B = 2 s . Therefore, the ratio A C B C = 2 s 2 1 s = 4 2 .