Pythagorean Theorem
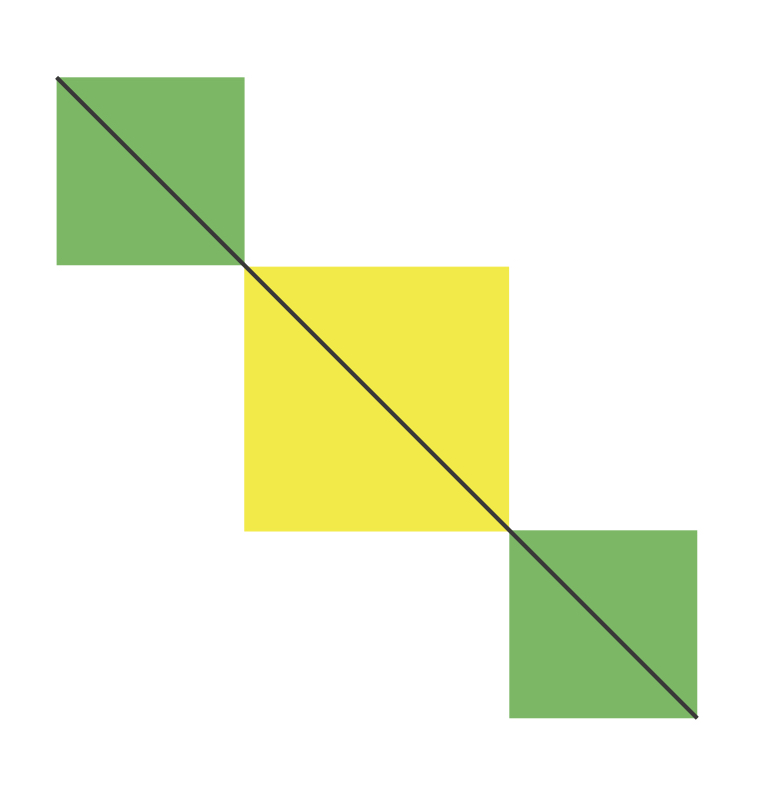
The following diagram shows two identical green squares and one larger yellow square. If the area of each green square is 9 and the area of the yellow square is 16, what is the length of the black diagonal line?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Using this I f a s q u a r e h a s a s i d e l e n g t h o f x , i t s d i a g o n a l h a s a l e n g t h o f x 2 So, the length of the line is 3 2 + 4 2 + 3 2 = 1 0 2 = 2 0 0
Use the areas to find the length of the sides of the squares. This would result in the green square having a side length of 3 and the yellow square having a side length of 4. Next add all three sides of the squares: 3 + 4 + 3 = 10. Now you know the length and height of the three squares stacked together. Next, plug in your value of 10 for the a and b variables in the pythagorean theorem equation: a 2 + b 2 = c 2 .  1 0 2 + 1 0 2 = c 2
1 0 0 + 1 0 0 = c 2
2 0 0 = c 2
2 0 0 =c