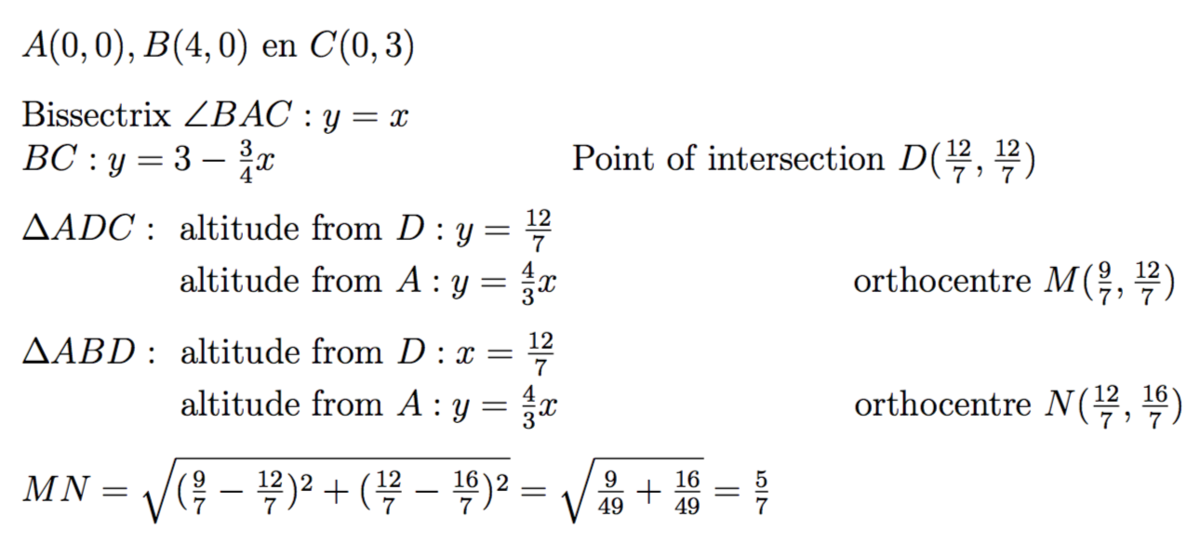
First off, consider the origin as the point having the right angle, B in triangle ABC.

Then, A = (4,0) , B = (0,0) and C = (0,3).

Use vertical angle bisector theorem and sections formula where the required point divides AC into 4 : 3 ratio.

Name this point as D. Now using sections formula, D = $(\frac { 0+4*3 }{ 4+3 } ,\frac { 4*3+0 }{ 4+3 } )$ = $(\frac { 12 }{ 7 } ,\frac { 12 }{ 7 } )$ .

Now to find orthocentre,O(x,y) of triangle ADB,

slope of AO x slope of BD = -1.

So, -1 = $\frac { y }{ x-4 }$ .

Also, as D and O lie on the same line( parallel to x-axis), y = 12/7.

Moreover, x = 4 - 12/7 = 16/7, which is $\frac { { AB }^{ 2 } }{ AB+BC }$ .

In the same way orthocentre of the other triangle is (12/7,9/7).

The distance between them using distance formula is 1/7 x $\sqrt { { 3 }^{ 2 }+{ 4 }^{ 2 } }$ . = 5/7 = $\boxed{0.714}$

Take the Vertices of the triangle as A(3,0),B(4,0) and origin

Now the angle bisector meets AB at D(12/7 , 12/7)

The perpendicular dropped from D meets OB at E(12/7,0)

Let another perpendicular from O on DB in triangle DOB meet DE at F(12/7 ,16/7)

therefore F is orthocenter of Triangle DOB.

Similarly do the same for triangle DOA.

The orthocenter thence found be G(9/7 , 12/7)

BY USING DISTANCE FORMULA

FG=5/7