Ratio Problem!
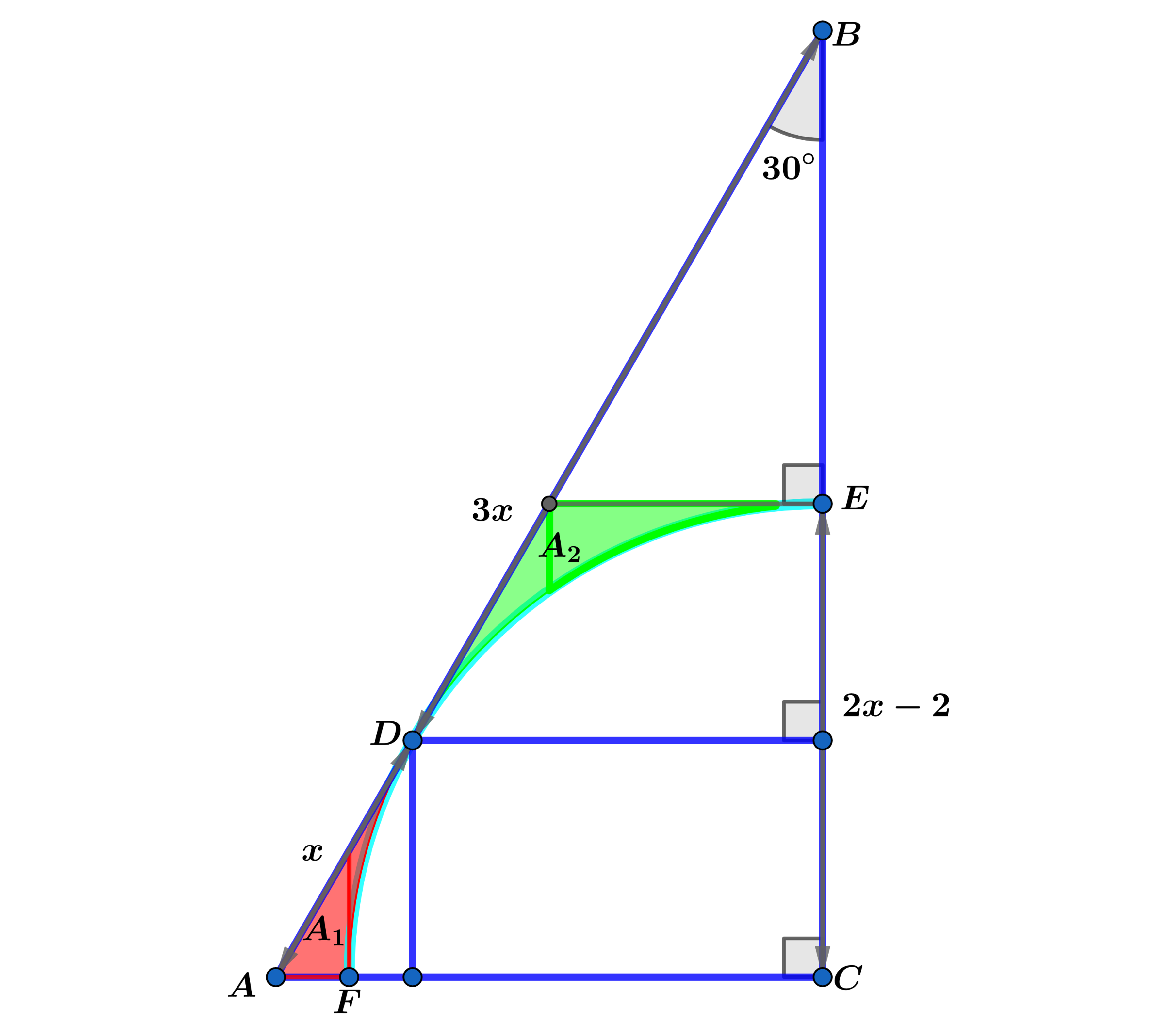
In right △ A B C , m ∠ A B C = 3 0 ∘ , A D = x , D B = 3 x and the quarter of the circle has radius C E = 2 x − 2 .
Find A 1 A 2 .
The answer is 2.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
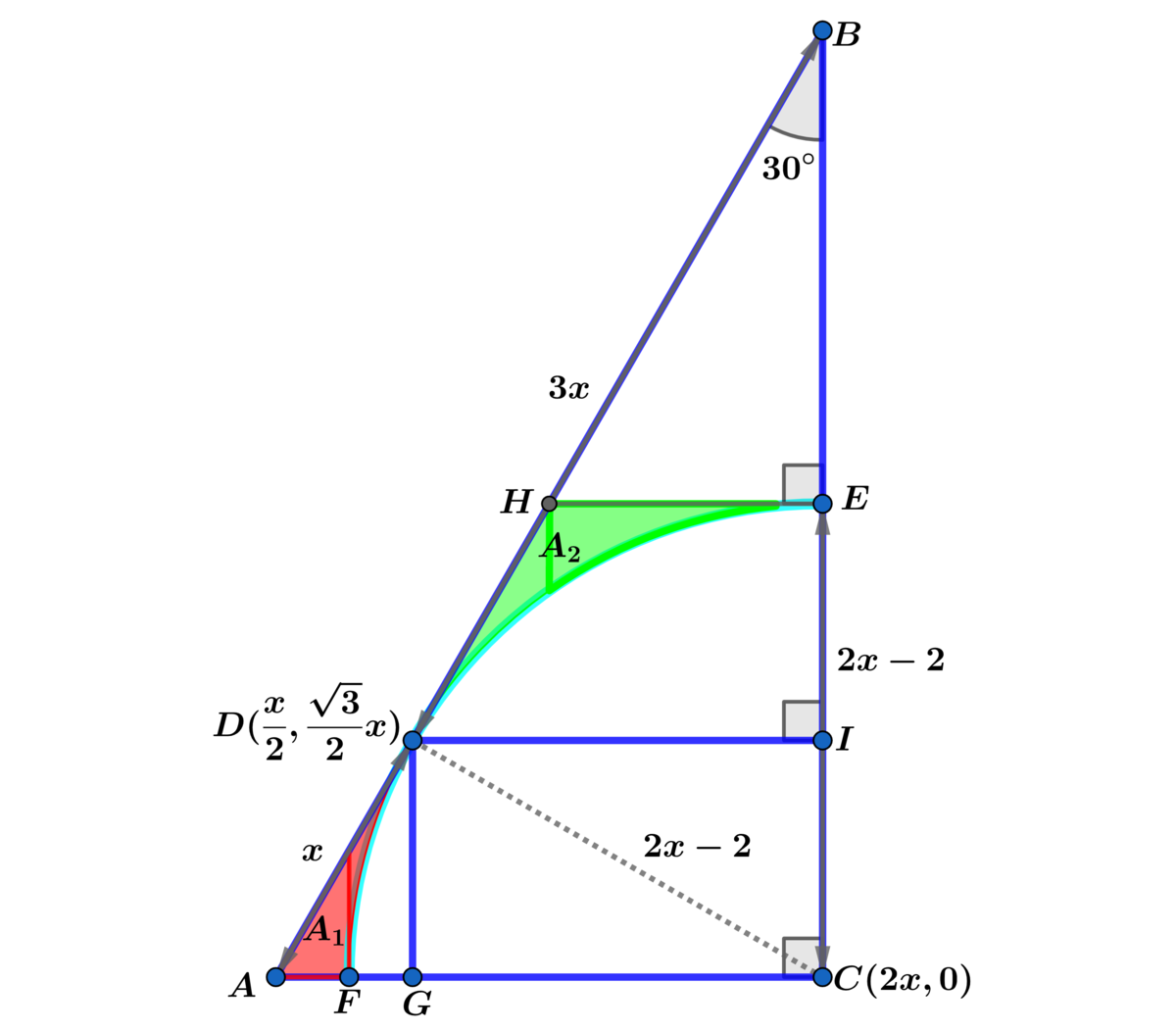
A F = 2 x − ( 2 x − 2 ) = 2 , G D = 2 3 x , A G = 2 x ⟹
( C D ) 2 = 4 ( x − 1 ) 2 = 1 6 x 2 − 3 2 x + 1 6 = ( 2 x − 2 x ) 2 + 4 3 x 2 = 1 2 x 2 ⟹ x 2 − 8 x + 4 = 0 ⟹ x = 4 ± 2 3
x = 4 − 2 3 ⟹ 2 ( x − 1 ) = 2 ( 3 − 2 3 ) < 0 ∴ choose x = 4 + 2 3
⟹ radius r = 2 x − 2 = 2 ( 3 + 2 3 )
For A 2 :
Let θ = m ∠ D C E
⟹ tan ( θ ) = 3 ⟹ θ = 3 π ⟹ A s e c t o r C D E = 2 1 θ r 2 = 2 1 ( 3 π ) ( 4 ) ( 3 + 2 3 ) 2 =
2 π ( 7 + 4 3 )
and A △ C D I = 2 1 ( 2 3 x ) ( 2 3 x ) = 8 3 3 x 2 = 8 3 3 ( 4 + 2 3 ) 2 = 2 3 3 ( 7 + 4 3 )
Let R 2 be region D E I ⟹ A R 2 = A s e c t o r C D E − A △ C D I = 2 ( 7 + 4 3 ) ( 4 π − 3 3 )
B C = 2 3 x ⟹ B E = 2 3 x − ( 2 x − 2 ) = 2 ( ( 3 − 1 ) x + 1 ) =
2 ( 2 3 + 3 ) and △ A D G ∼ △ H B E ⟹ 3 1 = 2 ( 2 3 + 3 ) H E ⟹ H E = 3 4 3 + 6 = 4 + 2 3 = x and D I = 2 3 x = 3 ( 2 + 3 ) and I E = C E − C I =
( 2 4 − 3 ) x − 2 = 3 + 2 3 ⟹ area of trapezoid
A D H E I = 2 1 ( 5 ) ( 2 + 3 ) ( 3 + 2 3 ) = 2 5 ( 1 2 + 7 3 )
⟹ A 2 = A D H E I − A R 2 = 4 8 + 2 8 3 − ( 1 4 + 8 3 ) π = 2 ( 2 4 + 1 4 3 − ( 7 + 4 3 ) π )
For A 1 :
m ∠ F C D = 6 π ⟹ A s e c t o r C D F = 2 1 ( 6 π ) ( 4 ) ( 3 + 2 3 ) 2 = π ( 7 + 4 3 )
and A △ D G C = 2 3 3 ( 7 + 4 3 )
Let R 1 be region F D G ⟹ A R 1 = A s e c t o r C D F − A △ D G C = 2 ( 7 + 4 3 ) ( 2 π − 3 3 )
and A △ A D G = 8 3 x 2 = 2 3 ( 7 + 4 3 )
⟹ A 1 = A △ A D G − A R 1 = ( 7 + 4 3 ) ( 2 3 − π ) = 2 4 + 1 4 3 − ( 7 + 4 3 ) π
⟹ A 1 A 2 = 2 .
E H ⊥ B C and intersects A B at H :
⟹ ∴ ⟹ ⟹ ∴ C D = r = C E R T △ C D H ≅ R T △ C E H ∠ E C H = ∠ D C H = 2 1 ∠ B C D = 3 0 ° R T △ C D H ≅ R T △ C D A ≅ R T △ C E H A 2 = 2 A 1 A 1 A 2 = 2
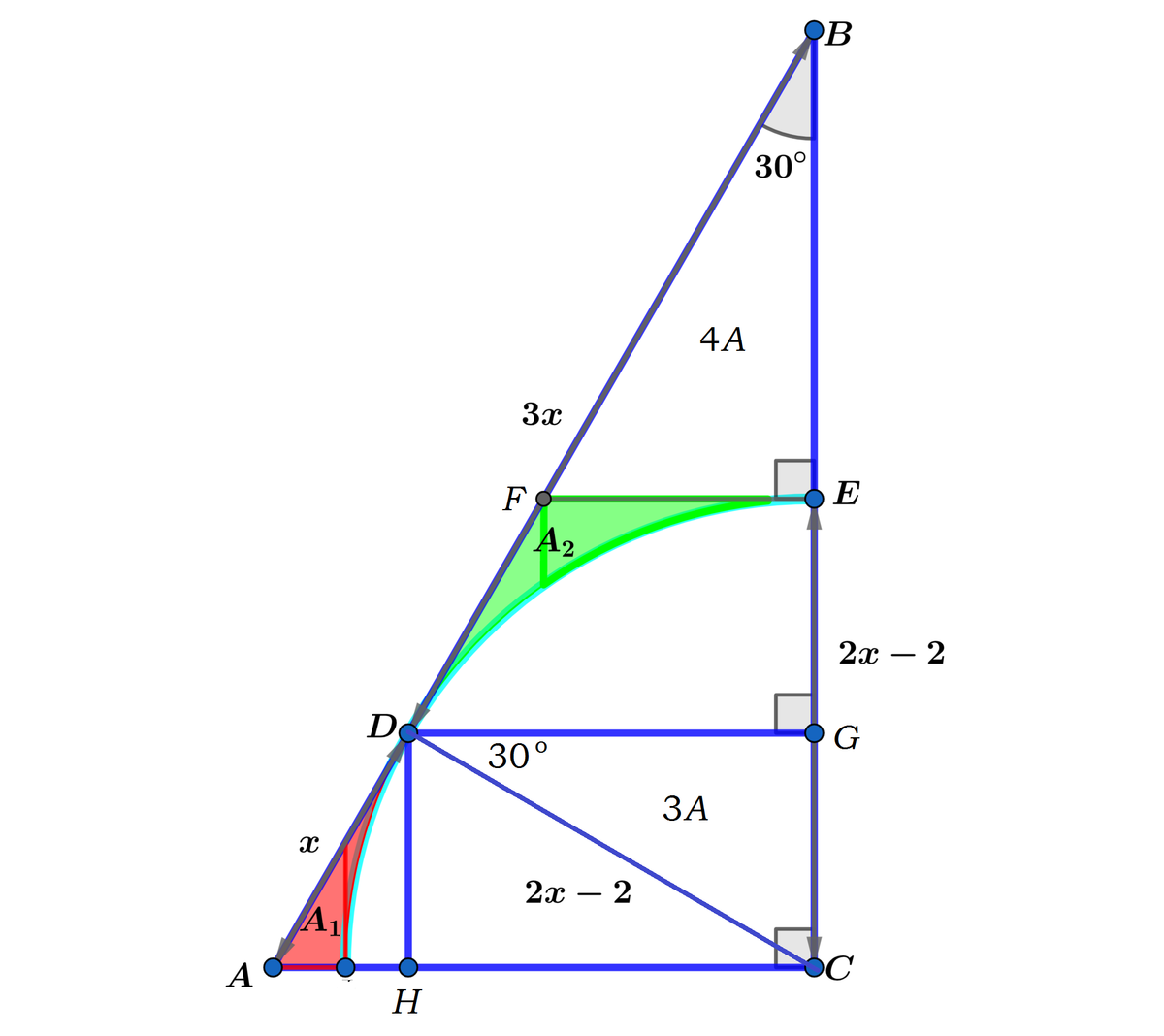
Label the two parallel lines to C A be E F and D G and D H the perpendicular line to C A .. By Pythagorean theorem ,
C G 2 + D G 2 ( A D ⋅ cos 3 0 ∘ ) 2 + ( D B ⋅ sin 3 0 ∘ ) 2 4 3 x 2 + 4 9 x 2 x 2 − 8 x + 4 ( x − 4 ) 2 ⟹ x = C D 2 = ( 2 x − 2 ) 2 = 4 x 2 − 8 x + 4 = 0 = 1 2 = 2 3 + 4 Note that C G = D H
Then C E = 2 x − 2 = 4 3 + 6 and B C = A B ⋅ cos 3 0 ∘ = 2 3 x = 8 3 + 1 2 = 2 ⋅ C E . Then the ratio of lengths B E : B G : B C = 2 : 3 : 4 . Let the area of △ A D H , [ADH] = A). then [ B E F ] = 4 A , [ A D G C ] = 1 2 A , A D G C = 7 A , [ D G C H ] = 6 A , and [ C D H ] = 3 A . Let r = 2 x − 2 . Then we have:
⎩ ⎪ ⎨ ⎪ ⎧ A 1 + A 2 = [ A F E C ] − 4 π r 2 = 1 2 a − 4 π r 2 A 2 = [ A D G C ] − [ C D H ] − 1 2 π r 2 = 4 A − 1 2 π r 2 . . . ( 1 ) . . . ( 2 )
( 1 ) − ( 2 ) : A 1 = 8 A − 6 π r 2 ⟹ A 2 A 1 = 2
Let the point to the left of E be H , and let B E = y .
Since ∠ A B C = 3 0 ° and A B = 4 x , from △ A B C we have A C = 2 x and B C = 2 3 x = 2 x − 2 + y .
By the tangent-secant theorem , ( 3 x ) 2 = y ( 4 x − 4 + y ) .
These two equations solve to x = 4 + 2 3 and y = 6 + 4 3 .
That means A C = 2 x = 2 ( 4 + 2 3 ) = 8 + 4 3 and E C = 2 x − 2 = 2 ( 4 + 2 3 ) − 2 = 6 + 4 3 .
Since ∠ H B E = 3 0 ° and B E = y = 6 + 4 3 , from △ H B E we have H E = 3 3 ( 6 + 4 3 ) = 4 + 2 3 = x = A D .
By the Pythagorean Theorem on △ A D C , D C = A C 2 − A D 2 = ( 8 + 4 3 ) 2 − ( 4 + 2 3 ) 2 = 6 + 4 3 = E C .
By the Pythagorean Theorem on △ H E C , H C = H E 2 + E C 2 = ( 4 + 2 3 ) 2 + ( 6 + 4 3 ) 2 = 8 + 4 3 = A C .
By the Pythagorean Theorem on △ H D C , H D = H C 2 − D C 2 = ( 8 + 4 3 ) 2 − ( 6 + 4 3 ) 2 = 4 + 2 3 = A D = H E .
Since H D = A D = H E , A C = H C , and D C = E C , triangles △ A D C , △ H D C and △ H E C are all congruent to each other by SSS congruency.
By corresponding parts of congruent triangles, ∠ A C D = ∠ H C D = ∠ H C E .
Since A 2 spans exactly twice the triangles and angles of A 1 , A 1 A 2 = 2 .