Reach for the Summit - P-S1-A4
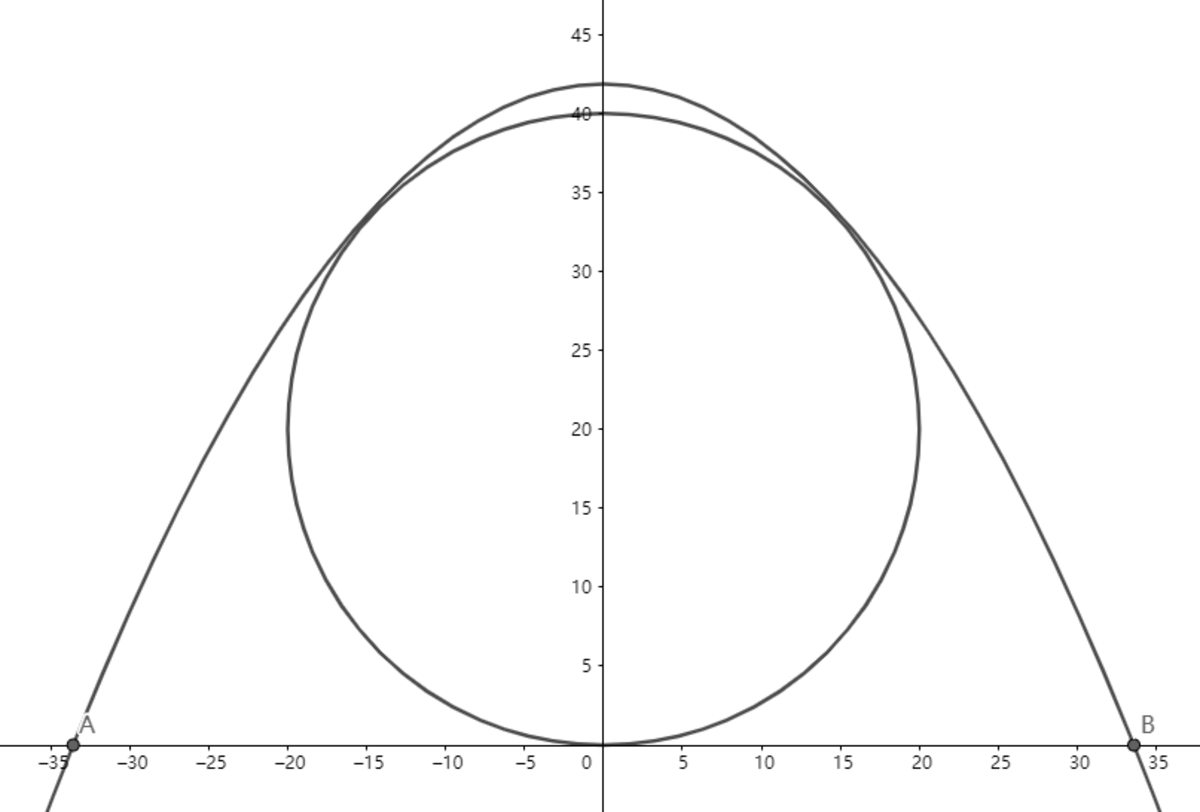
A pipe with diameter is put on the horizontal ground, and Ant-Man wants to jump over it to practice his strength. If Ant-Man is initially on the ground, and he can start to jump anywhere, what is the minimum initial velocity he should have to jump over the pipe? Let be the minimum velocity. Submit .
Assumptions:
- Ignore air resistance.
- He can be treated as a mass point.
- Take gravitational acceleration .
The answer is 2197.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.

Maximum height attained by a projectile projected at an angle α above horizontal with speed u is
H = 2 g u 2 sin 2 α
Horizontal range of the projectile is
R = = g u 2 sin 2 α .
Equation of path of the projectile is
x 2 = 4 H R 2 ( H − y )
Equation of the circle is
x 2 + y 2 − 5 y = 0
So the point of intersection of these two curves is obtained from
y 2 − ( 5 1 + 4 H R 2 ) y + 4 R 2 = 0
Since the two curves touch each other at this point, we have to have
( 5 1 + 4 H R 2 ) 2 = 4 × 1 × 4 R 2
⟹ g 2 u 2 cos 2 α − g 2 u 2 sin α cos α + 5 1 = 0
⟹ u 2 = cos α ( sin α − cos α ) 1
This will attain a maximum at α = 8 3 π
At this value of angle, the value of u is 2 . 1 9 7 3 6 8 . . . , and the answer is 2 1 9 7 .