Reaching with all of his three hands

Geometry Level pending

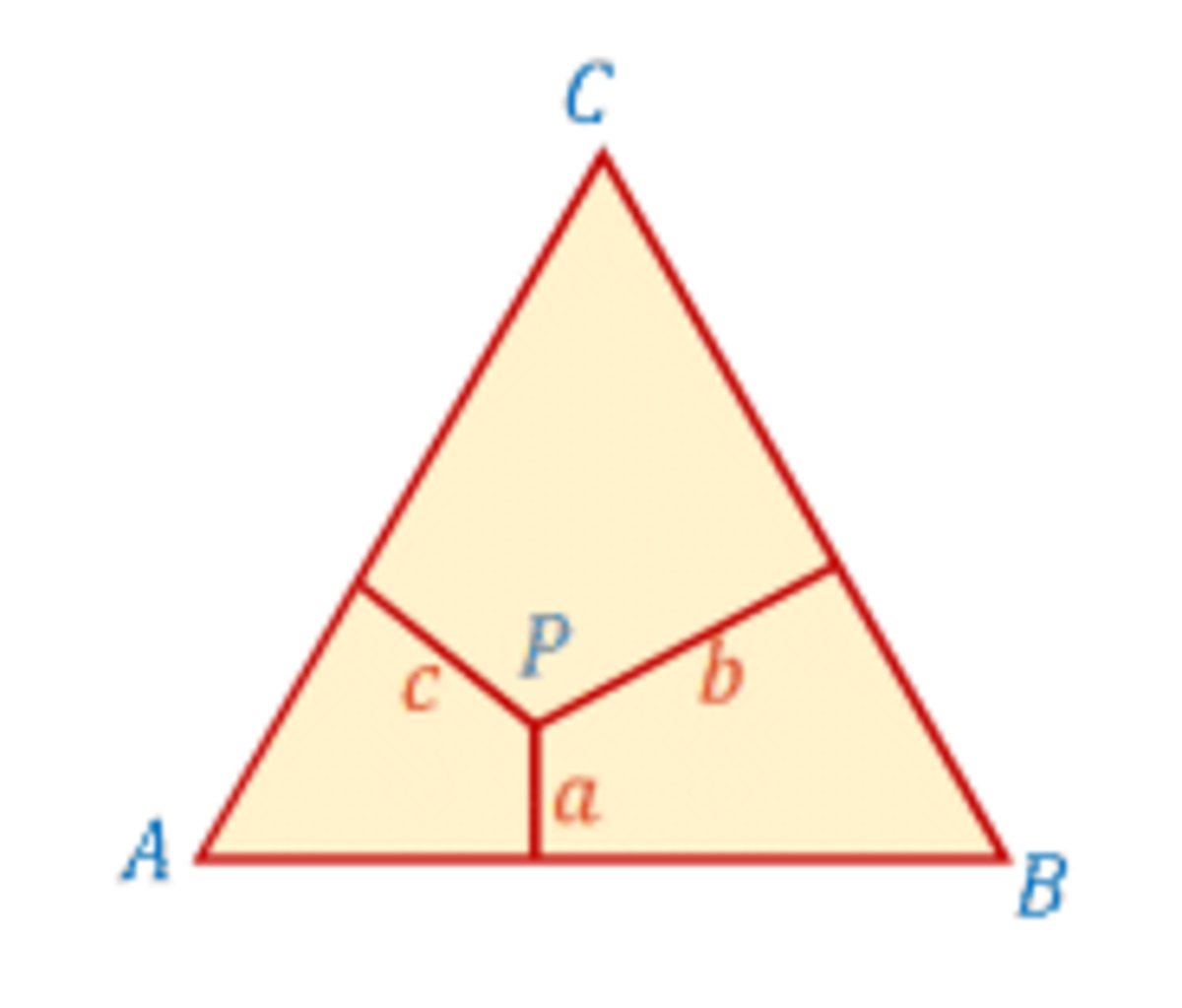Point P P is located inside a unit equilateral △ A B C \triangle ABC . Find the sum of its distances from the sides of the triangle, a + b + c a+b+c , to three decimal places.


The answer is 0.866.

Marta Reece by Marta Reece , 69, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Marta Reece
Apr 24, 2017

Area of △ A B C \triangle ABC is A = 1 2 3 2 A=\frac{1}{2}\frac{\sqrt{3}}{2}

It is also the sum of the areas of the three triangles A P B , B P C APB, BPC , and C P A CPA . A = 1 2 a + 1 2 b + 1 2 c A=\frac{1}{2}a+\frac{1}{2}b+\frac{1}{2}c

1 2 ( a + b + c ) = 1 2 3 2 \frac{1}{2}(a+b+c)= \frac{1}{2}\frac{\sqrt{3}}{2}

a + b + c = 3 2 ≈ 0.866 a+b+c=\frac{\sqrt{3}}{2}\approx0.866

5 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...