Real numbers
Number Theory
Level
1
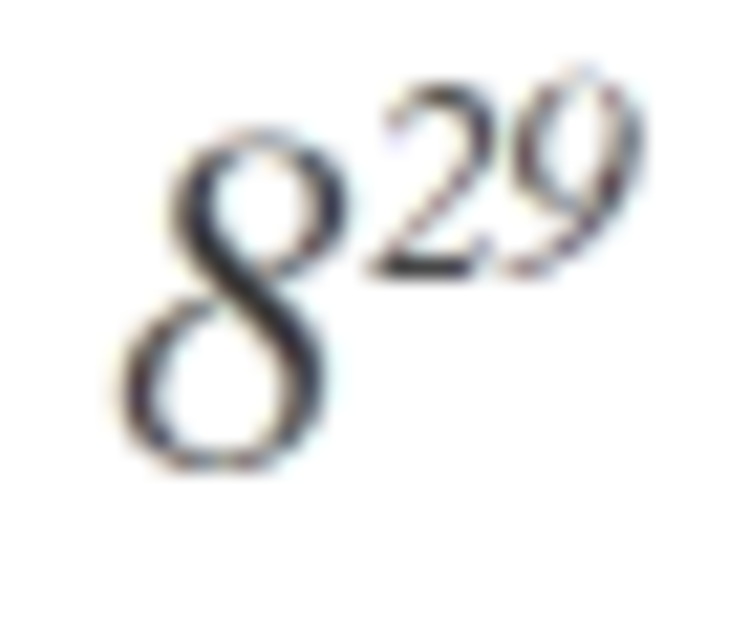 Find the unit digit of the number given
Find the unit digit of the number given
NOTE- just enter the single digit
The answer is 8.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The unit digit of the power of a number repeats every 4 powers. e.g. 2 1 → 2 , 2 2 → 4 , 2 3 → 8 , 2 4 → 6 , 2 5 → 2 . . . . . . Since 29 divided by 4 has a remainder of 1, it means that 8 2 9 has the same unit digit as 8 1 which is 8.