Really E-Z

Although this is not much of an explanation, I graphed as follows and got the radius to be $\boxed{29}$ .

Why do you all exclude the case when radius is 5. It is valid as well as the radius 29. But the circle will be "inside" the rectangle 8,9.

By Pythagorean Theorem

$R^2=(R-8)^2 + (R-9)^2$

$R^2 = (R^2 - 16R + 64) + (R^2 - 18R + 81)$

$R^2 = R^2 - 16R + 64 + R^2 - 18R + 81$

$0 = R^2 - 34R + 145$

By Quadratic Formula

$R=\dfrac{-b \pm \sqrt{b^2-4ac}}{2a}=\dfrac{-(-34) \pm \sqrt{(-34)^2-4(1)(145)}}{2(1)}=\dfrac{34\pm 24}{2}$

$R=\dfrac{34+24}{2}=29$ or $R=\dfrac{34-24}{2}=5$

$\text{Answer: R} = \boxed{\text{29 units}}$

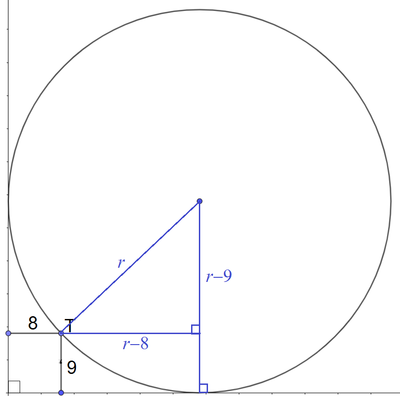
Let the radius of the circle be $r$ . We can see from the figure that by Pythagorean theorem :

$\begin{aligned} (r-8)^2 + (r-9)^2 & = r^2 \\ r^2 - 16r + 64 + r^2 - 18r + 81 & = r^2 \\ r^2 - 34r + 145 & = 0 \\ (r-5)(r-29) & = 0 & \small \blue{\text{Since }r > 5} \\ \implies r & = \boxed{29} \end{aligned}$

Let the radius of the circle be $r$ . Then

$(8-r)^2+(9-r)^2=r^2\implies r^2-34r+145=0\implies r=\boxed {29}$ (since $r>9$ ).