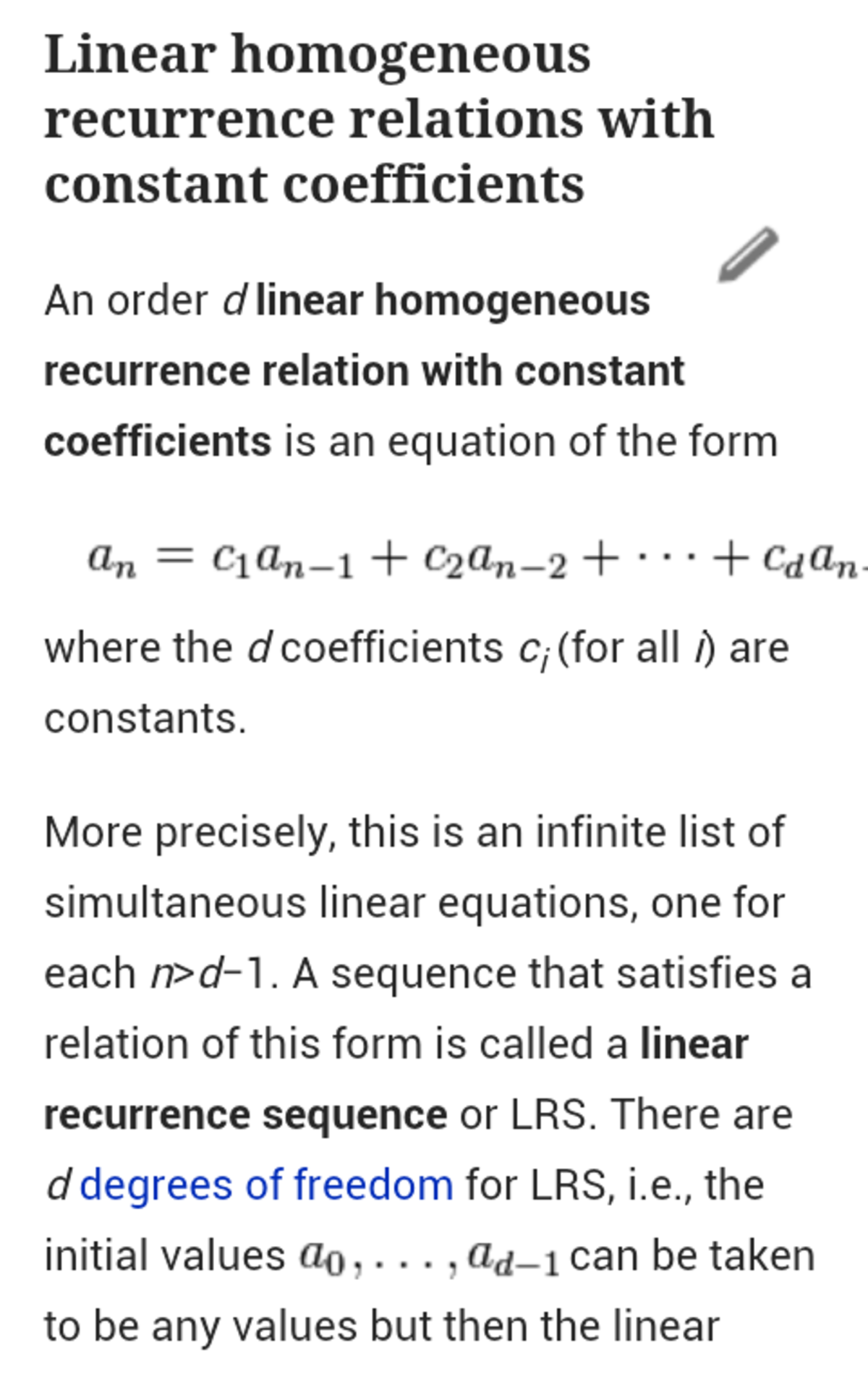
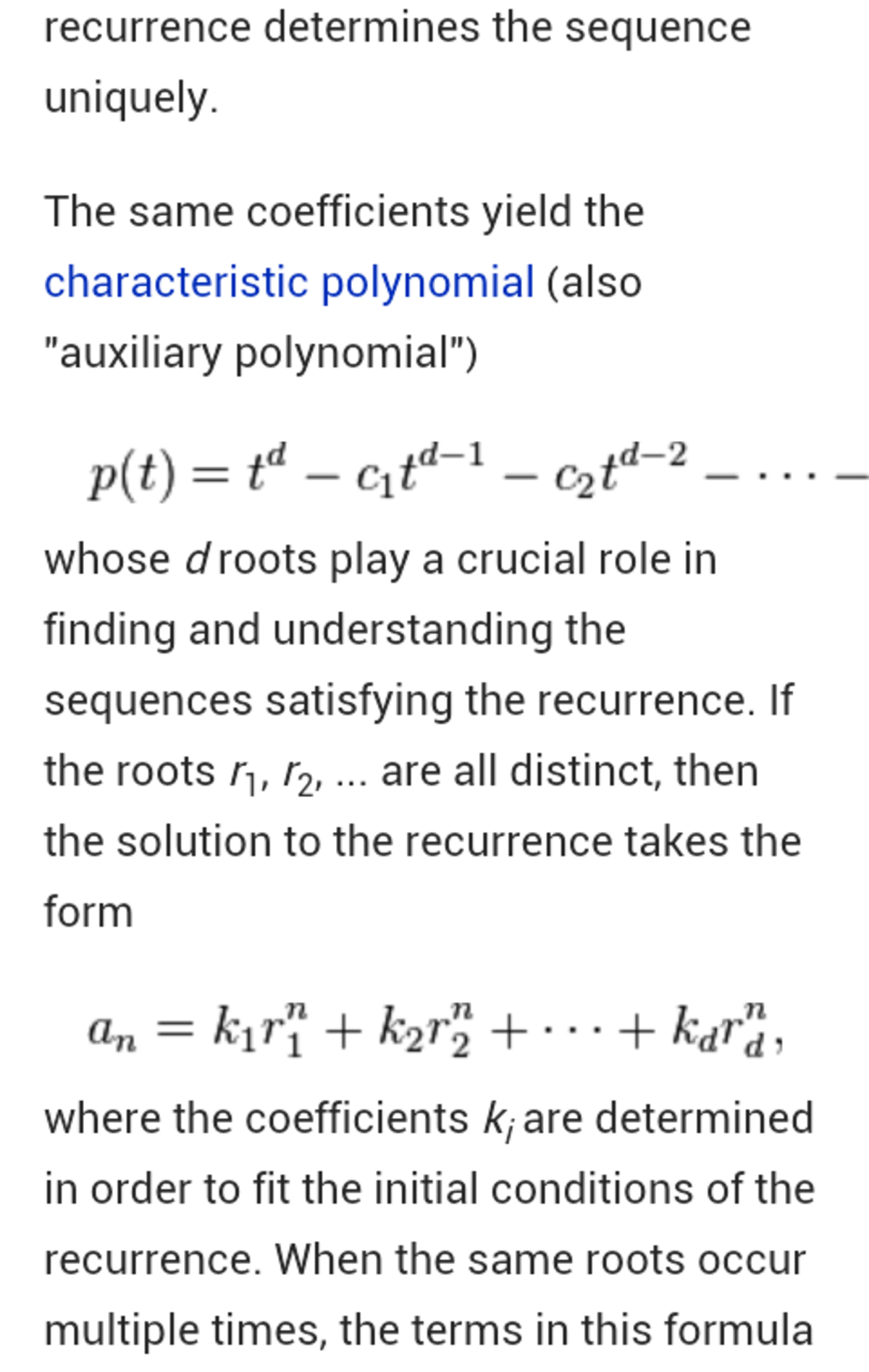
Recurring Styles #2 - Recurrence on Higher Degrees!

Calculus Level 5

$\Large{\frac{a_n}{a_{n-1}} = \sqrt[6]{\frac{a_{n-1}}{a^2_{n-2}}}}$

Define a sequence $a_n$ that satisfies the recurrence relation as described above where $n \geq 2 , a_0 = e , a_1 = e^2$ .

Find the value of $\displaystyle\large{ \lim_{n \to \infty} a_n}$ .

Bonus : Generalize for $a_n$ .

The answer is 1.

1 solution

Satyajit Mohanty
Jul 10, 2015

To understand the first step of second line, you need to learn this:

Can u explain the second line first step

Kyle Finch - 5 years, 11 months ago

Hi, @Kyle Finch , I've updated the solution. Hope you learn what is required to be learned to solve a linear homogeneous recurrence relation. :)

Satyajit Mohanty - 5 years, 11 months ago

I did the same way.

Ronak Agarwal - 5 years, 11 months ago

Thanks a lot dude

Kyle Finch - 5 years, 11 months ago

You need to check your problem. You are asking to find $\lim_{n\to\infty}\frac{a_{n}}{n}$ . In your solution here, you found $\lim_ {n\to\infty} a_{n}.$

Arturo Presa - 5 years, 10 months ago

I have learnt this in engineering .You guys already know it.Great man.Keep continuing .

Tejas Suresh - 5 years, 11 months ago

Recurring Styles #2 - Recurrence on Higher Degrees!

The answer is 1.

1 solution

0 pending reports