Red and Blue Areas

Geometry Level 3

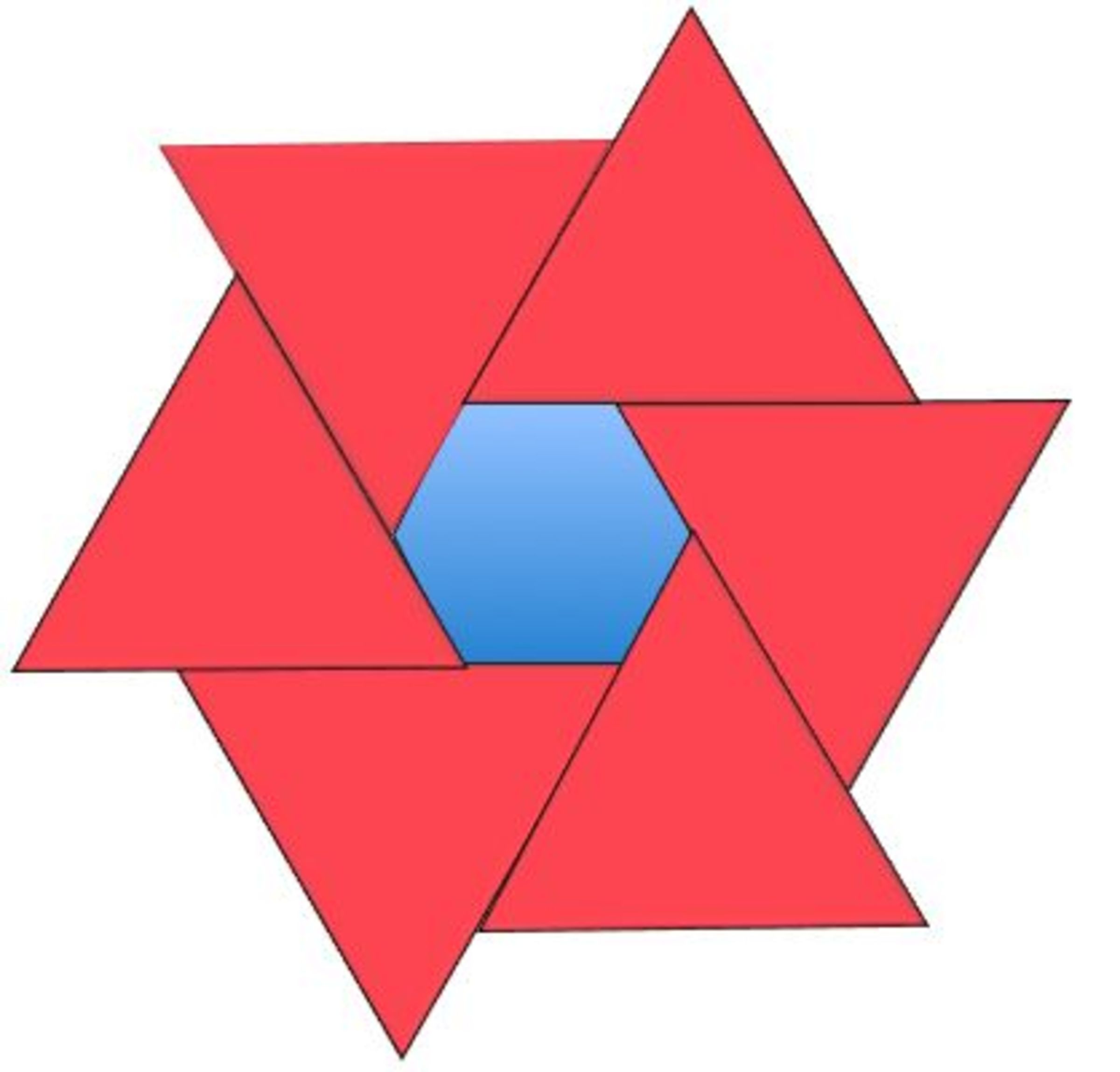Six red congruent equilateral triangles are attached to a blue regular hexagon. The side of the equilateral triangle is three times the side of the hexagon. What is the ratio of the Red area to the Blue Area?


The answer is 9.

Hana Wehbi by Hana Wehbi , 51, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Jeremy Galvagni
Aug 9, 2018

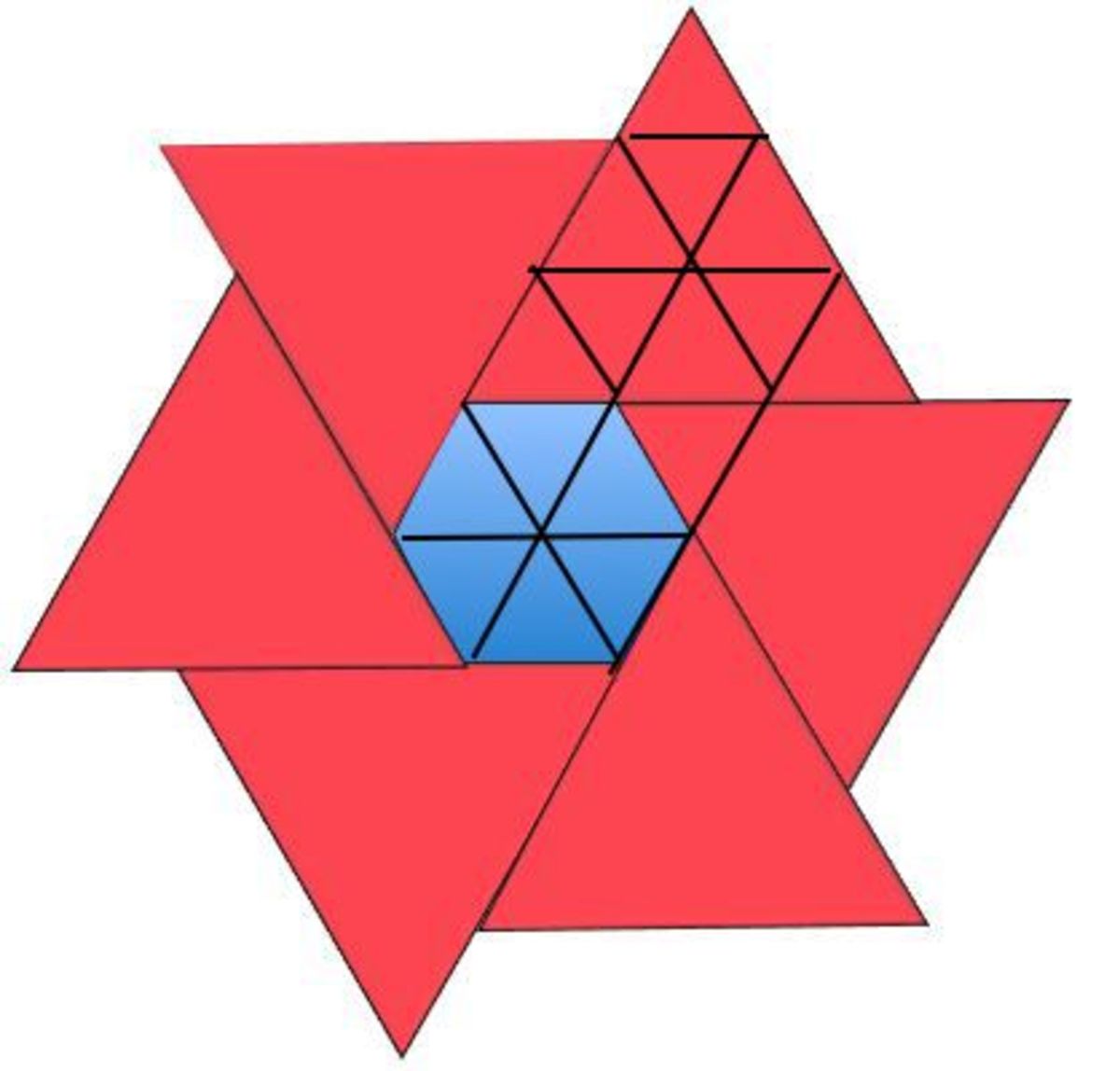
Make the hexagon into six congruent equilateral triangles. Each corresponds to one of the big red triangles. Each of these big triangles is 3 2 = 9 3^{2}=\boxed{9} of the smaller ones.

3 Helpful 0 Interesting 0 Brilliant 0 Confused
Hana Wehbi
Aug 12, 2018

A similar solution to @Jeremy Galvagni :

Each red triangle consists of 9 congruent small triangles, since we have 6 big red triangles with same area, their area will be 9 × 6 = 45 9\times 6=45

The hexagon is regular and consists of 6 congruent small triangles, thus, the ratio of both areas Red to Blue is : 45 6 = 9 \frac{45}{6}=9

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...