Reflecting Points
P
=
(
p
x
,
p
y
)
is a point in the plane.
X
is the reflection of
P
across the
x
-axis.
Y
is the reflection of
P
across the
y
-axis.
What point must the line X Y pass through?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Ah! So this is what you are trying to say! :)
Log in to reply
Is the question clear? Not many people answered this question.
Without having to work out the equation of the line, we can say that the gradient of − P x P y means that for every P x across, the line moves P y down. Since it starts at Y = ( − P x , P y ) , moving P x across and P y down brings it to the origin.
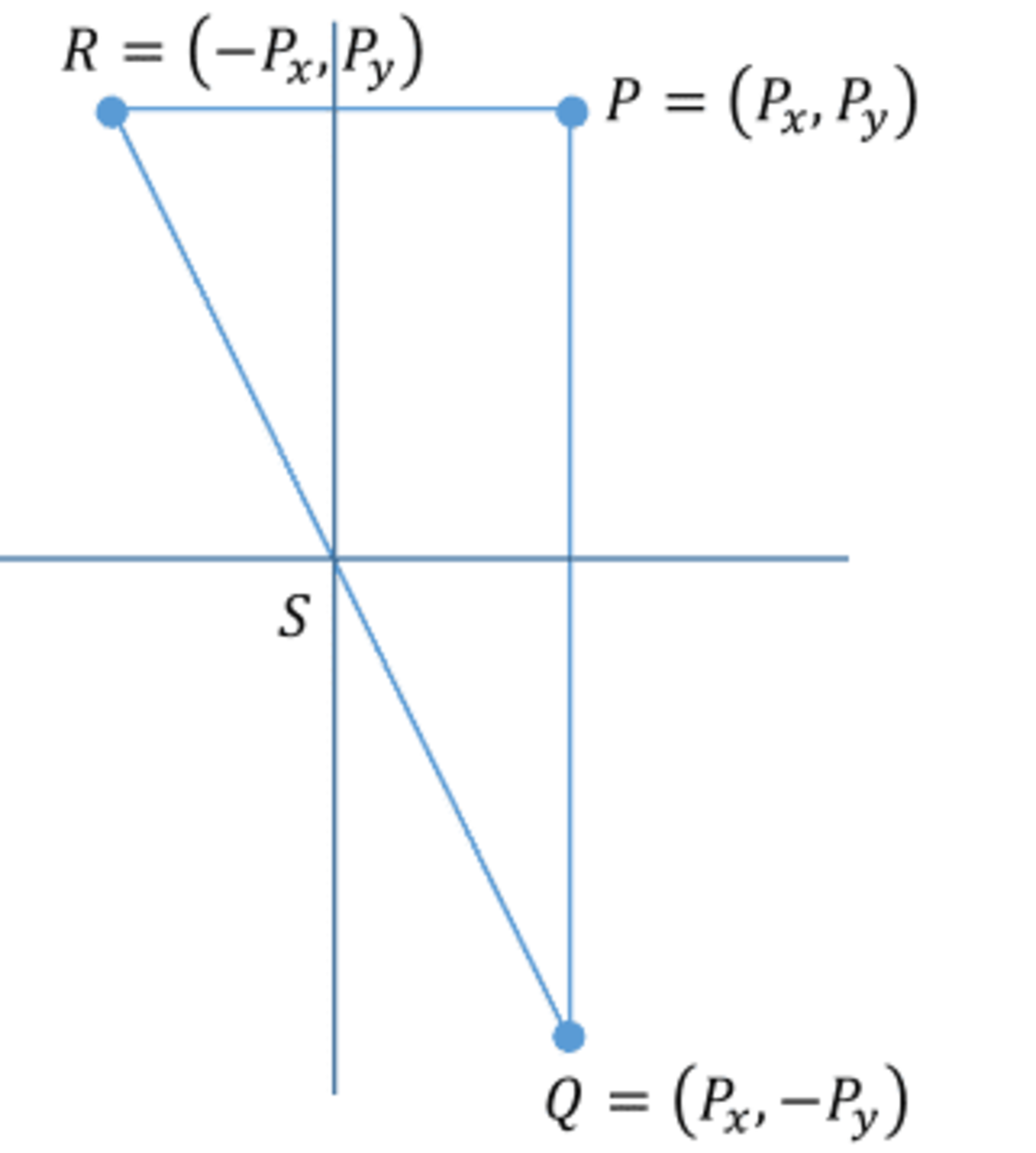Point
S
, half way between points
Q
and
R
, has coordinates which are the average of the coordinates of those two points.
S
=
(
2
P
x
−
P
x
,
2
−
P
y
+
P
y
)
=
(
0
,
0
)
X = ( p x , − p y ) and Y = ( − p x , p y )
The slope of the line is p x − ( − p x ) − p y − p y = − p x p y .
From the slope, we get that the equation of the line is y = − p x p y x .
Thus, this line passes through the origin ( 0 , 0 ) .