Related to the Folium of Decartes

Let $F(x, y)=x^3+y^3-9xy-c.$ We are going to prove that the answer to this problem is $-27.$ So we have to prove that when $c< -27$ there is a unique function $f(x)$ such that $F(x, f(x))= 0$ for any $x,$ and when $c=-27,$ there can be two different functions $f_1(x)$ and $f_2(x)$ such that $F(x, f_1(x))= 0$ and $F(x, f_2(x))= 0$ for any value of $x.$ The existence of the solution $f(x)$ for the implicit equation $F(x,y)=0$ can be proved easily using the fact that any third-degree polynomial has at least a real solution.

Then we are going to focus on the uniqueness of the solution when $c<-27$ and the existence of more than one solution when $c=-27.$

Let us define the region $R=\{(x, y)| x \:\text{and} \: y \: \text{ real numbers,} \: 3x\leq y^2\}.$ It is easy to see that the partial derivative $F_y$ is positive at any point of this region.

Using division and a little bit of algebra, we can prove that $F(x, y)=(x+y+3)((x-\frac{y+3}{2})^2+\frac{3}{4} (y-3)^2)-(c+27).\quad\quad (*)$ Then if $c=-27,$ we can obtain from the equation $F(x, y)=0$ that $x+y+3=0$ or $x=y=3.$ Then one of the solution of the given implicit equation can be $f_1(x)=-x -3,$ and another solution $f_2(x)$ can be obtained by changing the value of the function $f_1(x)$ to $3$ at $x=3.$

If $c<-27$ then from (*), we get that $(x+y+3)((x-\frac{y+3}{2})^2+\frac{3}{4} (y-3)^2)<0.$ Then $x+y+3<0,$ and, therefore, $x+f(x)+3<0.$ So the whole graph of the solution $f(x)$ below the line $x+y+3=0,$ and, therefore, in the region $R.$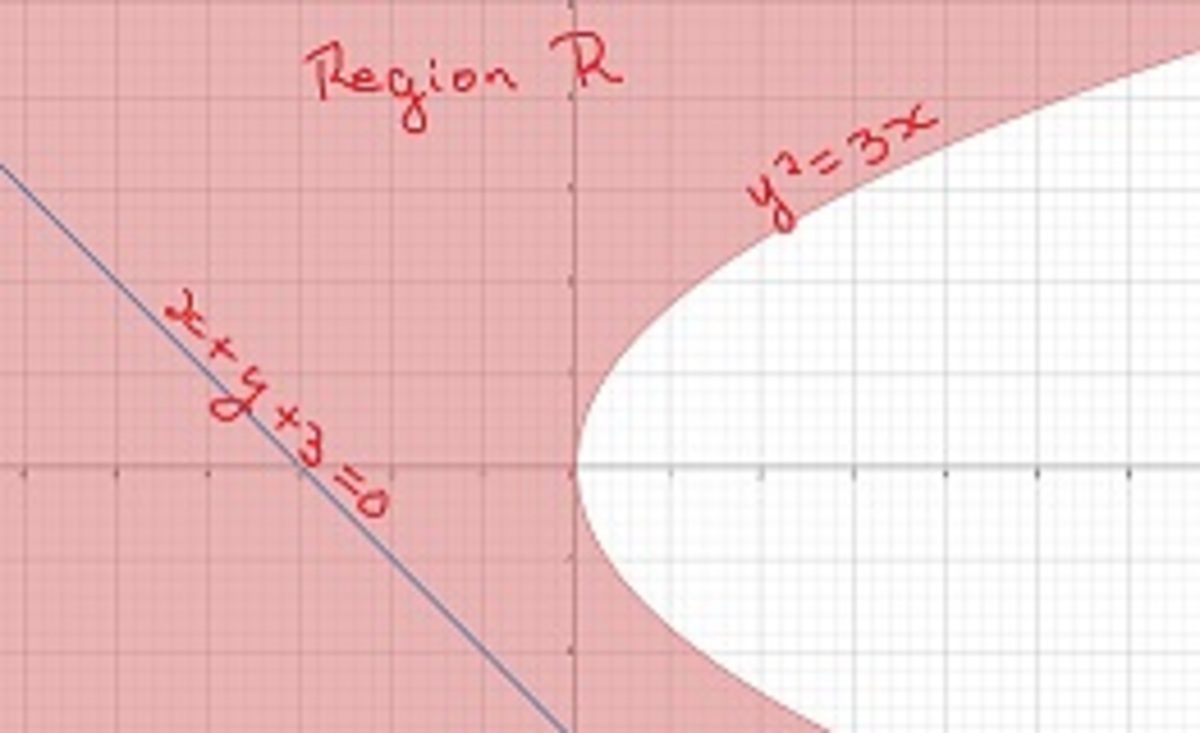

We know that $F_y>0$ at any point of $R.$ Now, let us use the contradiction method to prove that the solution of our implicit equation is unique in this case. Assume that there are two different solutions of $F(x, y)=0,$ that can be denoted by $f_1(x)$ and $f_2(x).$ Since they are different functions there must be a number $a$ such that $f_1(a)\neq f_2(a).$ Then $F(a, f_1(a))=F(a, f_2(a))=0$ and the points $(a, f_1(a))$ and $(a, f_2(a))$ are both in the region $R$ and this contradicts the fact that $F_y>0$ at any point of $R.$ Notice that the regions defined by $x+y+3<0$ is convex and it is contained in $R,$ so the segment determined by the points $(a, f_1(a))$ and $(a, f_2(a))$ is completely contain in that region. Then the contradition is derived from the Mean Value Theorem. This proves the uniqueness of the solution of the implicit equation, when $c<-27.$ So, the answer to this problem is $\boxed{-27}.$

The given equation is a cubic in $f(x)$ , with discriminant $\Delta=-27\left(x^6-2(c+54)x^3+c^2\right)$

When this discriminant is positive, the cubic equation has three real roots - ie, there are three possible values for $f(x)$ , and the function is not well defined.

Completing the square, $\Delta=-27\left[\left(x^3-(c+54)\right)^2-108c-2916\right]$

so the maximum value of $\Delta$ occurs at $x=\sqrt[3]{c+54}$ , when $\Delta=27\left[108c+2916\right]=2916[c+27]$

This is positive if and only if $c>-27$ ; so the required value is $b=\boxed{-27}$ .