Relativistic Rotating Disk
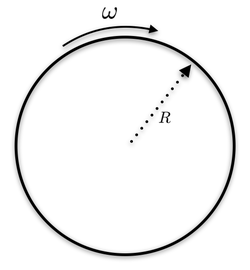 A disk with radius
is rotating with a large angular velocity of
. According to relativistic kinematics, what is the ratio of the disk's circumference to its radius, as observed in an inertial frame where the disk does not translate?
A disk with radius
is rotating with a large angular velocity of
. According to relativistic kinematics, what is the ratio of the disk's circumference to its radius, as observed in an inertial frame where the disk does not translate?
Bonus Thought: According to elementary geometry, the answer is . Is it? If not, what's going on here?
The answer is 3.47.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The relative velocity at any point is given by ω R Hence the circumference will have a lorentz expansion given by
1 − c 2 ( ω R ) 2
But the radius won't undergo any expansion because it is perpendicular to the direction of motion Thus the ratio becomes
R 2 π R 1 − c 2 ( ω R ) 2 = 2 π 1 − c 2 ( ω R ) 2 = 3 . 4 7