Right triangle perimeter
A right triangle has a hypotenuse of 75. If its area is 756, what is its perimeter?
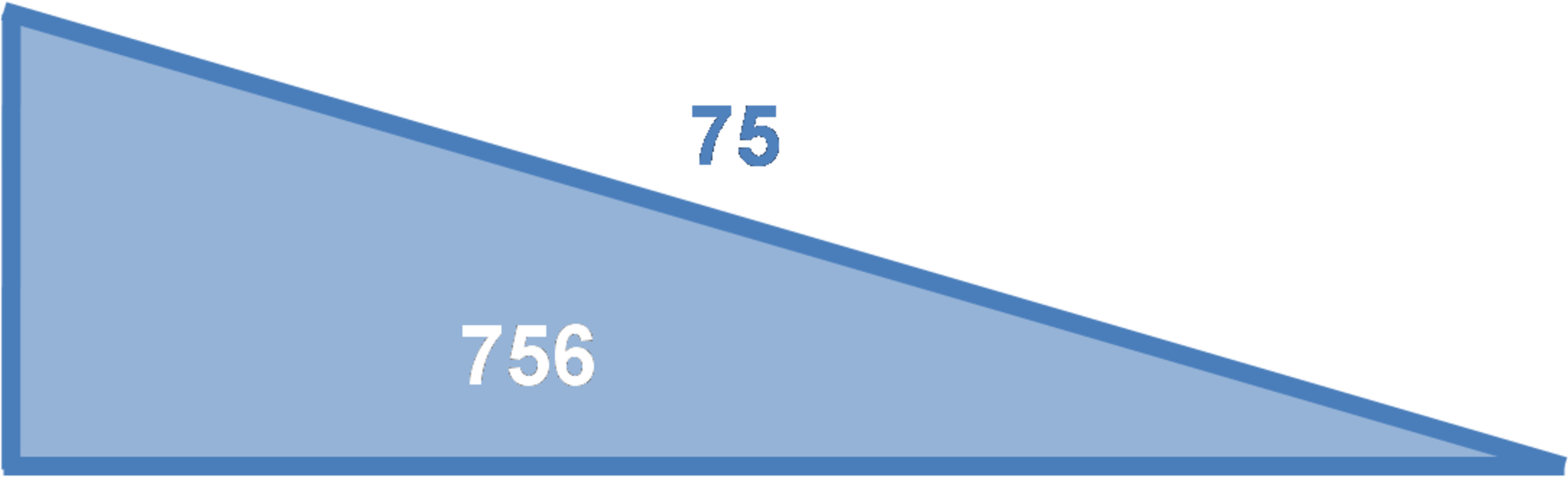
The answer is 168.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Let a and b be the legs of the right triangle. Then
A = 2 1 a b
7 5 6 = 2 1 a b
1 5 1 2 = a b
a = b 1 5 1 2 ( 1 )
By pythagorean theorem, we have
a 2 + b 2 = 7 5 2
a 2 + b 2 = 5 6 2 5 ( 2 )
Substitute ( 1 ) in ( 2 ) .
( b 1 5 1 2 ) 2 + b 2 = 5 6 2 5
b 2 2 2 8 6 1 4 4 + b 2 = 5 6 2 5
Multiplying both sides by b 2 , we get
2 2 8 6 1 4 4 + b 4 = 5 6 2 5 b 2
b 4 − 5 6 2 5 b 2 + 2 2 8 6 1 4 4 = 0
Let x 2 = b 4 and x = b 2 , then
x 2 − 5 6 2 5 x + 2 2 8 6 1 4 4 = 0
By using the quadratic formula,
x = 2 5 6 2 5 ± 2 ( − 5 6 2 5 ) 2 − 4 ( 2 2 8 6 1 4 4 ) = 2 5 6 2 5 ± 2 4 7 4 3
x = 5 1 8 4 and x = 4 4 1
The value of b can be 5 1 8 4 = 7 2 or 4 4 1 = 2 1 . The value of a follows, it can be 7 2 or 2 1 . (depending on the label on the figure or assumption: example: let a be the longer leg and b be the shorter leg or let a be the shorter leg and b be the longer leg ......etc)
The desired perimeter is 7 2 + 2 1 + 7 5 = 1 6 8
The Pythagorean theorem states that a 2 + b 2 = c 2 . Adding 2 a b to both sides turns the left hand side equal to the square of a + b . Thus, a + b = c 2 + 2 a b . Since 756 is 2 a b , 2 a b must then be 7 5 6 × 4 = 3 0 2 4 , and since c = 7 5 , c 2 = 5 6 2 5 . Adding 2 a b and c 2 and taking the square root, we get c 2 + 2 a b = 9 3 . Since c 2 = a 2 + b 2 , we can obtain the equation a 2 + 2 a b + b 2 = 9 3 . Since a 2 + 2 a b + b 2 = ( a + b ) 2 , a + b = 9 3 . Now that we know the sum of the lengths of the two legs along with the length of the hypotenuse, we can simply add them up to find that p = a + b + c = 9 3 + 7 5 = 1 6 8 .