Rising bubble
A small spherical gas bubble of diameter d = 4 μ m forms at the bottom of a pond. When the bubble rises to the surface its diameter is n = 1 . 1 times bigger. What is the depth in meters of the pond?
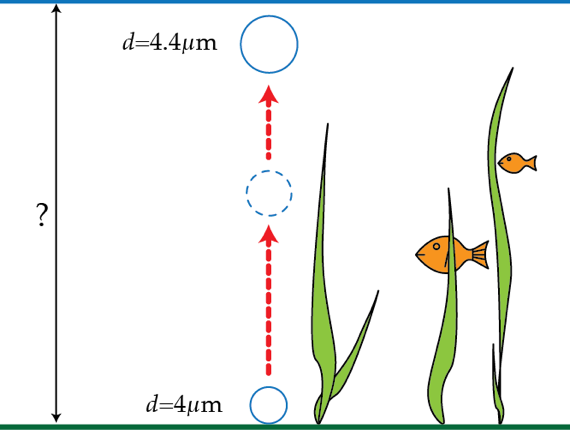
Details and assumptions
The atmospheric pressure is p A = 1 0 5 Pa , the acceleration of gravity is g = 9 . 8 m / s 2 and water's surface tension and density are σ = 7 3 × 1 0 − 3 N / m and ρ = 1 × 1 0 3 kg / m 3 , respectively. The gas expansion is assumed to be isothermal.
The answer is 4.94.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
nice
Wont surtace tension due to water reduce the pressure inside the bubble as it tend contract at surface of the bubble?🙋😊
why is this 2 σ /r and not 4 σ /r...since there are 4 surfaces
Log in to reply
This is not a soap bubble that has two surfaces. This is a bubble in the water, with one surface.
Good one!
Why is it + 2sigma/r I thought it was 4sigma/r?
Log in to reply
Thanks for your comment. It would be r 4 σ if the bubble is on the air since it will have 2 layers. But in the water, it only has 1 layer between the air inside the bubble and the water, so it would be r 2 σ .
The pressure and volume of the bubble when it is at the bottom of the pond are:
p 1 = p A + ρ g h + r 2 σ = p A + ρ g h + d 4 σ
V 1 = 3 4 π r 3 .
The pressure and volume of the bubble when it rises to the surface are:
p 2 = p A + r ′ 2 σ = p A + n d 4 σ
V 2 = 3 4 π n 3 r 3 .
Since the gas expansion is isothermal, we have: p 1 V 1 = p 2 V 2
Therefore, p A + ρ g h + d 4 σ = n 3 ( p A + n d 4 σ ) .
So, h = ρ g n 3 ( p A + n d 4 σ ) − ( p A + d 4 σ ) = 4 . 9 4 ( m ) .