RMO 2001; Part - 1

Geometry Level 3

In $\Delta ABC$ , $D$ is a point on $BC$ such that $AD$ is the internal angle bisector of $\angle A$ . Suppose $\angle B = 2 \angle C$ & $CD = AB$ , then find the measure of $\angle A$ in degrees .

The answer is 72.

2 solutions

Hemang Sarkar
Jul 14, 2014

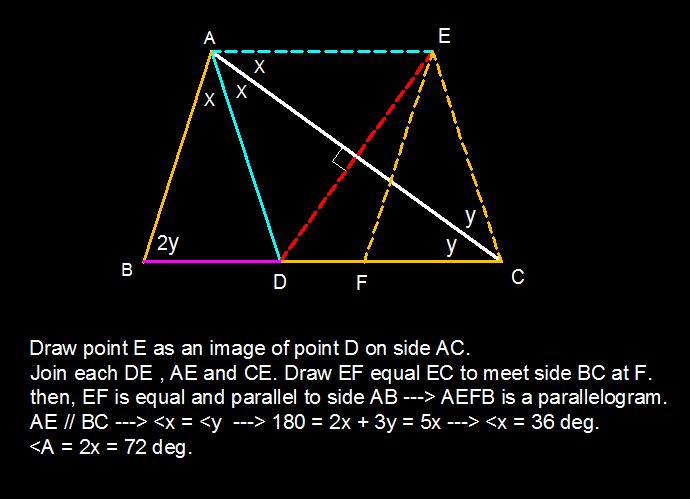
let angle DAC = angle DAB = y. let angle ACD = x and angle ABD equal 2x. we have 2y+3x = 180. applying sine law on both triangles. DC/siny = AD/sinx. and AD/sin2x = AB/sin(x+y). we are given that AB=DC. we can now get sinx/siny = sin2x/sin(x+y). on solving, sin(x-y) = 0.. combine this with 2y+3x = 180.

PURE GEOMETRY SOLUTION- CONST- DRAW ANGLE BIC. OF ANGLE B. JOIN DE.

1) ANGLE EBC = ANGLE ECB = ANGLE C. THAT IS EB=EC .

2)AB=AC. ANGLE ABE= ANGLE DCE. BE=EC. TRIANGLE ABE IS CONGRUENT TO TRIANGLE DCE GIVING US AE=DE.

3) THEREFORE ANGLE EAD = ANGLE EDA .

4) EXTEND RAY 'DE' TILL SOME pT. F . ANGLE EAD= ANGLE EDA= A/2. BY EXTERIOR ANGLE THEOREM, ANGLE AEF=A.

5) ANGLE BEA = ANGLE EBC + ANGLE ECB = C+C =2C. AS TRIANGLE BEA IS CONGRUENT TO TRIANGLE CED, ANGLE BEA= ANGLE CED= 2C.

6)BUT ANGLE CED = ANGLE AEF (VERTICALLY OPPOSITE) THEREFORE 2C=A.

AT LAST, IN TRIANGLE ABC, ANGLES A+B+C=180 THAT IS 2C+2C+C=180

HENCE, C=36 HENCE ANGLE A=2C=2(36)=72

PLZZ DRAW DIAG. WITH CONSTRUCTION AND REFER TO THIS

Nihar Mahajan - 6 years, 5 months ago

Ahmad Saad
Mar 3, 2017

RMO 2001; Part - 1

The answer is 72.

2 solutions

0 pending reports