RMO ( #1)

Geometry Level 4

Let ABCD be a unit square. Draw a quadrant of circle with A as centre and B,D as end points of the arc. Similarly , draw a quadrant of a circle with B as centre A and C as end points of the arc. Inscribe a circle S touching arcs AC and BD both externally and also touching the side CD. Find the radius of the circle S

0.125 0.625 6.25E-2 0.25

Ratul Pan by Ratul Pan , 21, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Ratul Pan
Sep 16, 2015

we can easily prove that AOE congruent to BOE(R.H.S.)
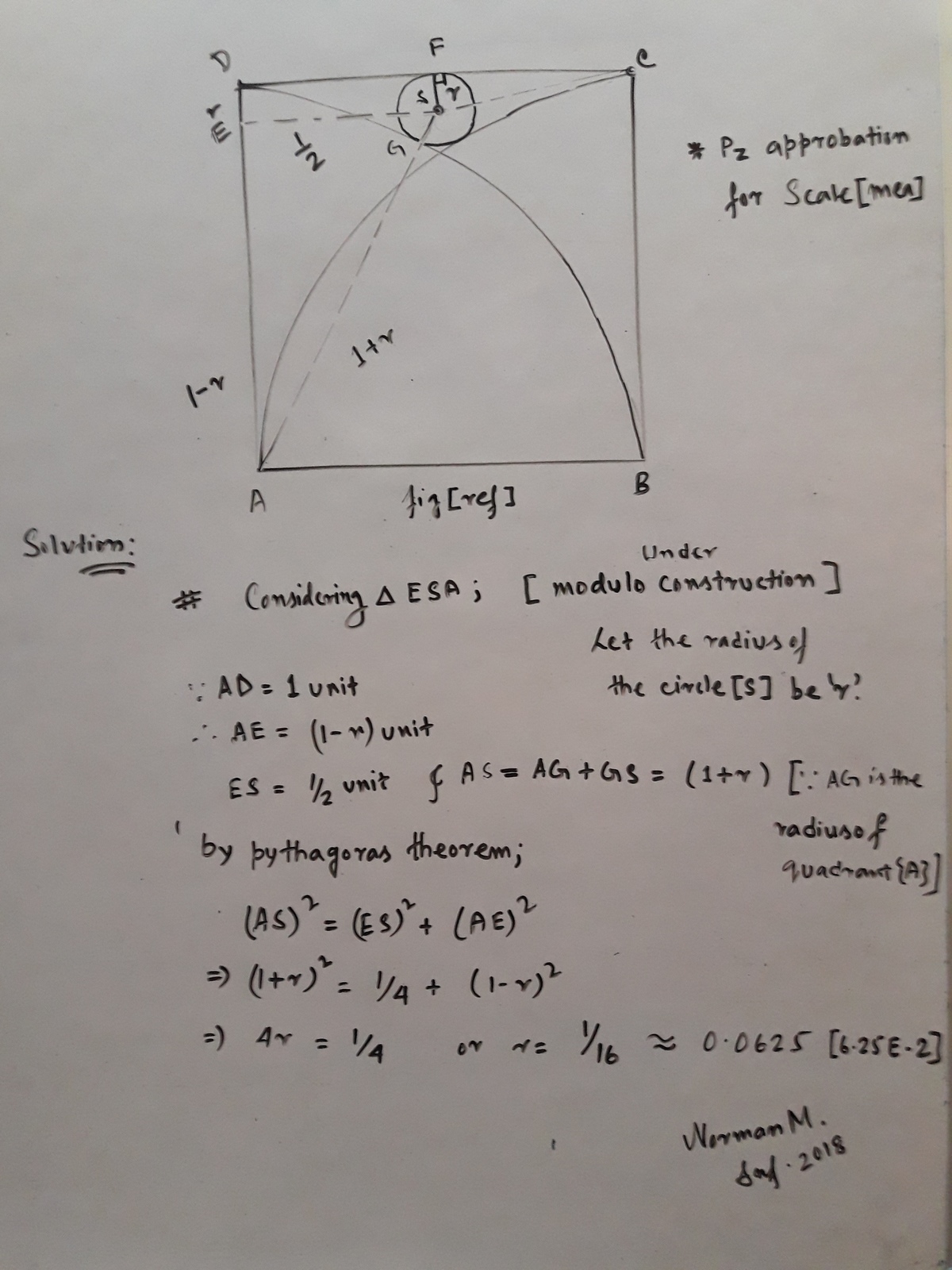
therefore, AE=EB=1/2
By pythagoras theorem A O 2 AO^2 = A E 2 AE^2 + E O 2 EO^2
or, ( R + r ) 2 (R+r)^2 = ( R − r ) 2 (R-r)^2 +1/4
or, ( 1 + r ) 2 (1+r)^2 = ( 1 − r ) 2 (1-r)^2 +1/4 [as R=1]
or, r = 1/16
or, r=0.0625

1 Helpful 0 Interesting 0 Brilliant 0 Confused

Good solution. I always lack in Geom :3

Nice one. :)

Mehul Arora - 5 years, 8 months ago

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...