Rod, Disk and Pulley

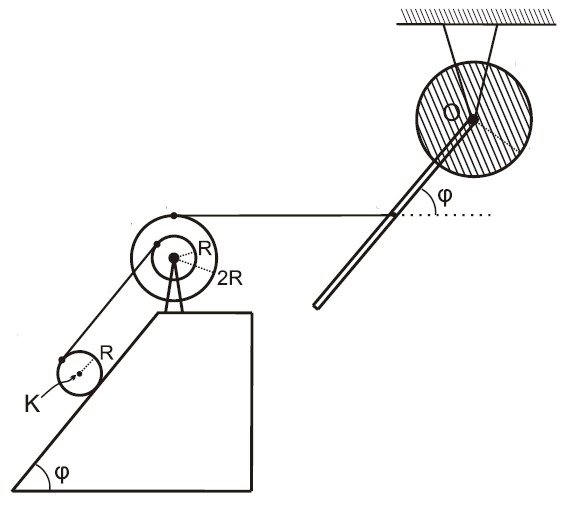The below system is in balanced. Thin homogeneous rod of length 3m and mass 8kg is welded at one end of the center of homogeneous disk of mass 4kg and radius $\frac{\sqrt 2}{2}$ m. The system of these (rod-disc) can rotate without friction as one, around fixed horizontal axis passing through the center of the disk and perpendicular to level of the disc. The middle of the rod is tied with a thin horizontal weightless and non-stretching rope with a double pulley and the rod forms an angle φ with the extension of the horizontal rope. The double pulley consists of two homogeneous welded coaxial discs with R and 2R radiuses, where R = 0,2 m and its angular mass towards the axis that passes through its center and is perpendicular to its level is equal to I = 1.95kgm^2.

A second thin weightless and non-stretching rope, which is parallel to angled inclination angle φ, is wrapped many times inside of the radius disk R of the pulley and its other end is wrapped around the circumference of a homogeneous cylinder with mass 30 kg and radius R as shown in the figure. At t=0 the rope 1 is cutted. Calculate the velocity of center K when it has moved a 2m distance on the ramp.