Rolling Ellipse

The locus of the focus will be a circle having radius $2a$ and centre at $(-\sqrt{a^2-b^2},0)$ .

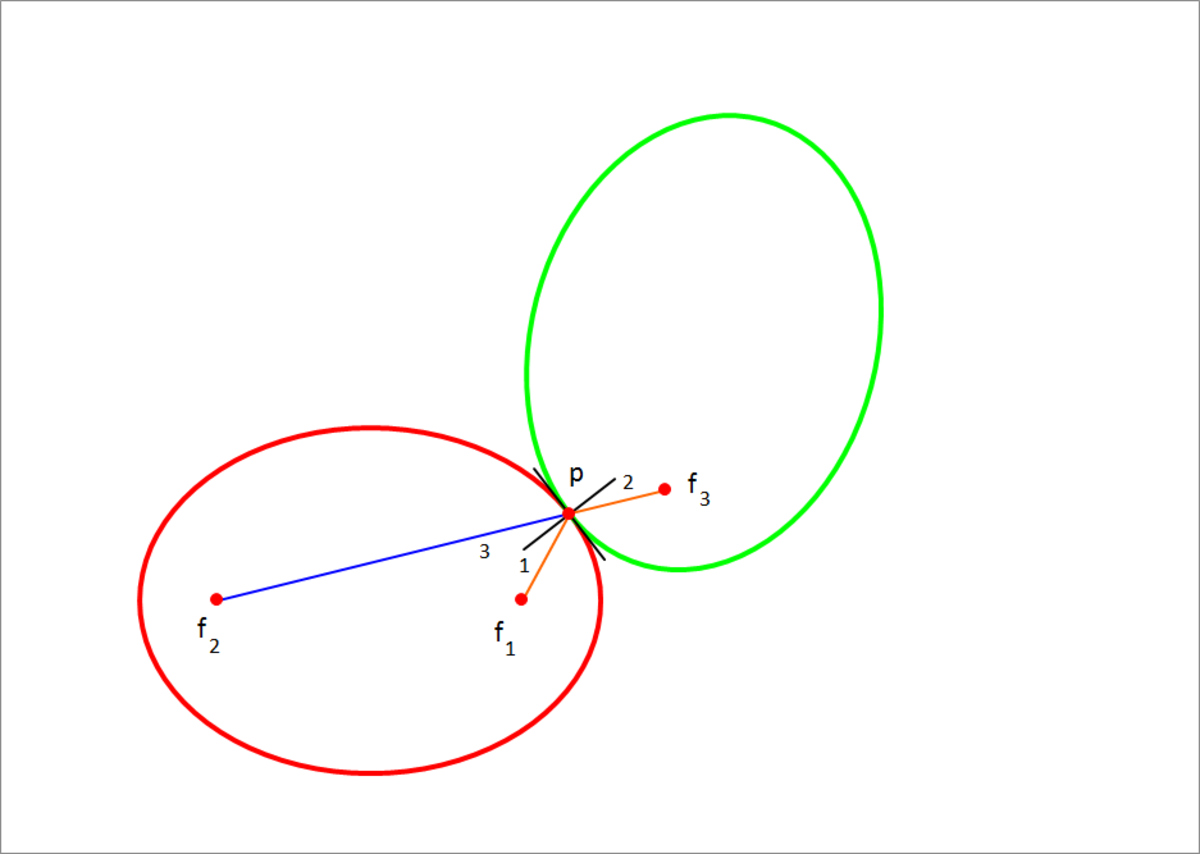
As the two ellipses are congruent, it follows that the rolled ellipse and the fixed ellipse are mirror images about the common tangent. Hence $\angle 1 = \angle 2$ . Also we know from the properties of ellipses that the normal at any point on the ellipse bisects the angle between the line segments connecting the point to the two foci. Hence $\angle 1 = \angle 3$ . From this, it follows that the points $p$ , $f_2$ and $f_3$ are collinear. Further, we know that the sum of $\overline{p f_3} + \overline{p f_2} = \overline{p f_1} + \overline{ p f_2 } = 2 a$ , where $a$ is the semi-major axis length. Hence the focus of the rolling ellipse is at a constant distance equal to $8$ from the left focus of the fixed ellipse. Therefore, the locus is a circle with radius equal to $8$ , from the which the length is the circumference of this circle which is equal to $16 \pi = 50.265$ .

The following GIF image shows the trajectory of the rolling ellipse focus.