Rotate the Vessel

For a particle of mass $m$ at the surface, $mgy - \tfrac12mv^2 = \text{const.}.$ Thus, comparing a particle at the edge and one in the center, $mg\Delta h - \tfrac12m(2\pi f r)^2 = 0.$ This simplifies to $\Delta h = \frac{2 \pi^2 f^2 r^2}g;$ in the given units, $\pi^2$ and $g$ cancel, and we have $\Delta h = 2\cdot 2^2\cdot (0.05)^2 = \SI{0.02}{m} = \boxed{\SI{2}{cm}}.$

here is my solution to this question:-

Consider the rotating frame. Acting on a particle of liquid in this frame we have two forces:

• gravity (potential $U_g = mgh$ )
• the centrifugal force $F = -m \omega ^2 r$ , where $\omega$ is the angular speed and $r$ is the radius of rotation

The centrifugal force has an effective potential of $U_c = \int F dr = -m \omega ^2 \frac{r^2}{2}$

The surface is an equipotential curve, so it can be described by the equation:

$U_g+U_c=\mathrm {const.}$

$mgh - m \omega ^2 \frac{r^2}{2}=\mathrm{const.}$

$h= \frac {\omega ^2 r^2}{2g}+\mathrm{const.}$

The angular speed is $\omega=2×2\pi =4\pi \ \mathrm{rad/s}$

Therefore the height difference between the centre and the edge is: $\Delta h = \frac{(4\pi )^2 0.05^2}{2 \pi ^2}-\frac{(4\pi ) ^2 0^2}{2 \pi ^2}=0.02 \ \mathrm{m}$

Bernoulli's Equation . d=density of liquid , Then $1/2*dv^2=dgh$ where, v = angular velocity * radius solve to get 'h'.

The surface of the liquid will be perpendicular to the total acceleration. (If it weren't, it would flow until it was.)

Therefore the slope of the liquid at any given point is

$f'(r) = \frac{r {\omega} ^ 2}{g}$ where $\omega=2 \pi f$ is the angular frequency.

Integrating the slope to get the curve,

$f(r) = \frac{(r \omega)^2}{2 g}$

Substituting,

$f(0.05)=\frac{(.05*2*2 \pi)^2}{2 \pi ^2} = 0.02$ , or 2 cm.

Wow. This exact problem (2rps & .05m) is online, only instead of just saying g=π^2, it sets g=10 & π^2=10 (which accomplishes the same thing).

http://physics.qandaexchange.com/?qa=1565/rotating-cylindrical-vessel-with-liquid

Let $f(x)$ be the height at radius $x$ with $f(0) = 0$ . Water surface will always be orthogonal to total acceleration:

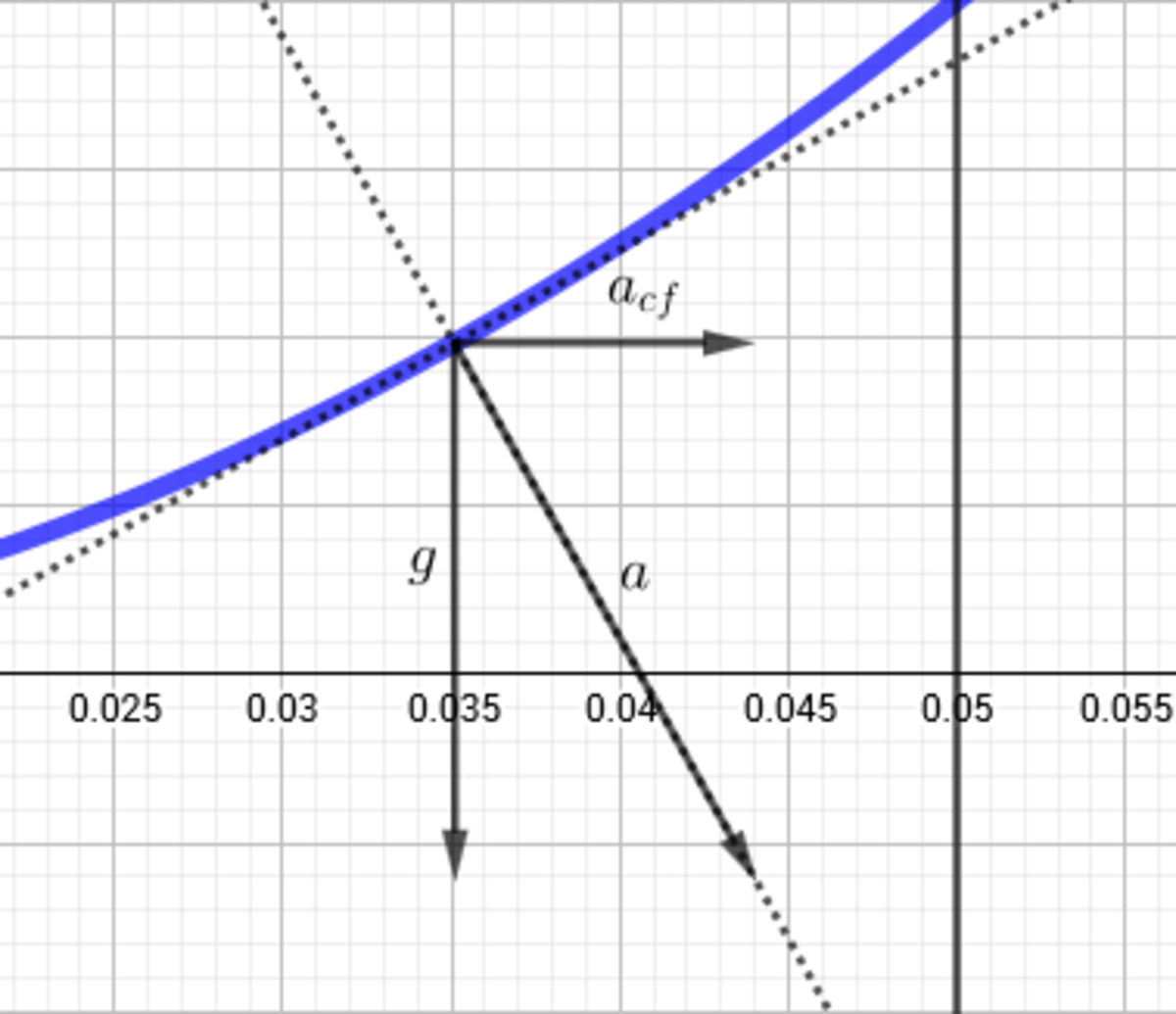
Describing the graph of $f$ as curve $(x,f(x))$ with velocity vector $(1,f'(x))$ , and given that centrifugal acceleration at radius $x$ is $a_{cf}=\omega^2x$ , we get that $\langle (1,f'(x)),(\omega^2x,-g)\rangle = 0$ which gives us differential equation $f'(x) = \frac{\omega^2}{g}x$ and with inital condition $f(0) = 0$ this gives us $f(x) = \frac{\omega^2}{2g}x^2$ .

Finally, $\omega = 2\pi f = 4\pi\,\mathrm{rad}/s$ , and $f(x) = 8x^2$ . This gives us $f(0.05) = 0.02$ , i.e. $\Delta h = 2\,\mathrm{cm}$ .