Rotation and Elongation of a SPRING - 3

Classical Mechanics Level 3

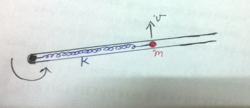A very long thin tube is hinged at a point such that its free to rotate in the horizontal plane.Inside the tube there is spring fixed at its end.the spring is connected to a mass 'm'...which precisely fits into the tube. At the instant t=0 when the spring is in its natural length the mass is given velocity 'V' in a direction perpendicular to the length of the tube.

WILL THERE EVER BE ANY COMPRESSION IN THE SPRING?

YES NO DEPENDS ON m DEPENDS ON K DEPENDS ON V DEPENDS ON THE NATURAL LENGTH OF THE SPRING

Yash Sharma by Yash Sharma , 24, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Since the centrifugal pseudo force is acting outward, there will be no compression but rather there will be elongation.

1 Helpful 0 Interesting 0 Brilliant 0 Confused

please highlight the word horizontal plane i mistook it as vertical and wrongly marked it as a) ie depends on v.

aryan goyat - 5 years, 7 months ago

Log in to reply

Can you please report it? I am not the author... It's @Yash Sharma

Kishore S. Shenoy - 5 years, 7 months ago
Apoorva Singal
Jan 20, 2021

The spring will elongate due to centrifugal force. Since it's elongation force is equal to centrifugal it's contraction force will also be equal to centrifugal force which will cancel and it will not contract.

0 Helpful 0 Interesting 0 Brilliant 1 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...