Rubber Physics (Part 7)

Classical Mechanics Level 3

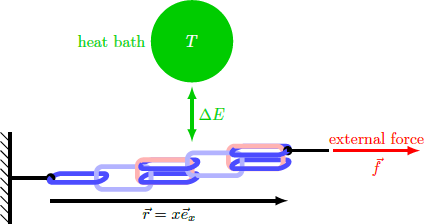
Now consider the case where a one-dimensional linear chain of N N chain links of length l l is connected to a heat bath at room temperature T = 293 K T = 293 \,\text{K} , so that heat exchange with the environment takes place. In addition, a external force f ⃗ = f e ⃗ x \vec f = f \vec e_x acts on the chain. As already shown in part 4 , the alignments l ⃗ i = ± l e ⃗ x \vec l_i = \pm l \vec e_x of the chain links are binomially distributed. However, the probabilities p + p_+ and p − p_- for the alignment in positive and negative x-direction, respectively, are now different and obey a Boltzmann distribution: p + = A exp ⁡ ( + l f k B T ) p − = A exp ⁡ ( − l f k B T ) A = 1 exp ⁡ ( + l f k B T ) + exp ⁡ ( − l f k B T ) = 1 2 cosh ⁡ l f k B T \begin{aligned} p_+ &= A \exp \left(+ \frac{l f}{k_B T} \right)\\ p_- &= A \exp \left(- \frac{l f}{k_B T} \right)\\ A &= \frac{1}{\exp \left(+ \frac{l f}{k_B T} \right) + \exp \left(- \frac{l f}{k_B T} \right)} = \frac{1}{2 \cosh \frac{lf}{k_B T}} \end{aligned} with the Boltzmann constant k B ≈ 1.38 ⋅ 1 0 − 23 J / K k_B \approx 1.38 \cdot 10^{-23}\,\text{J}/\text{K} . The pre-factor A A is chosen so that the normalization condition p + + p − = 1 p_+ + p_- = 1 is met. Calculate the mean value ⟨ x ⟩ \langle x \rangle of the displacement for the vector r ⃗ = x e ⃗ x \vec r = x \vec e_x that connects the beginning and the end of the chain. Derive an equation for the linear force constant k k of the chain with k = ( ∂ f ∂ ⟨ x ⟩ ) ⟨ x ⟩ = 0 = ( ∂ ⟨ x ⟩ ∂ f ) f = 0 − 1 k = \left( \frac{\partial f}{\partial \langle x \rangle} \right)_{\langle x \rangle = 0} = \left( \frac{\partial \langle x \rangle}{\partial f} \right)_{f = 0}^{-1} Give as result the numerical value for the force constant k k with two significant digits in units of newtons per meter. Assume a unit length l = 0.35 nm l = 0.35 \,\text{nm} and a number N = 1 0 4 N = 10^{4} of chain links.


The answer is 0.0000033.

Markus Michelmann by Markus Michelmann , 35, Germany

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Markus Michelmann
Jan 21, 2018

We want to find out the following mean value ⟨ x ⟩ = ⟨ r ⃗ ⟩ ⋅ e ⃗ x = ⟨ n ⟩ l = ( 2 ⟨ N + ⟩ − N ) l \langle x \rangle = \langle \vec r \rangle \cdot \vec e_x = \langle n \rangle l = (2 \langle N_+ \rangle - N) l with the number N + = 0 , 1 , … , N N_+ = 0,1,\dots, N chain links aligned in positive x-directions, that is bionomially distributed. We have already shown in part 4, that this mean value results ⟨ N + ⟩ = N p + \langle N_+ \rangle = N p_+ Therefore, we get a displacement ⟨ x ⟩ = ( 2 N p + − N ) l = N l ( 2 e β l f e β l f + e − β l f − 1 ) = N l 2 e β l f − ( e β l f + e − β l f ) e β l f + e − β l f = N l e β l f − e − β l f e β l f + e − β l f = N l sinh ⁡ ( β l f ) cosh ⁡ ( β l f ) = N l tanh ⁡ ( β l f ) ⇒ ∂ ⟨ x ⟩ ∂ f = β N l 2 sinh ⁡ ′ ( β l f ) cosh ⁡ ( β l f ) − sinh ⁡ ( β l f ) cosh ⁡ ′ ( β l f ) cosh ⁡ 2 ( β l f ) = β N l 2 cosh ⁡ 2 ( β l f ) − sinh ⁡ 2 ( β l f ) cosh ⁡ 2 ( β l f ) = β N l 2 1 cosh ⁡ 2 ( β l f ) ⇒ k = ( ∂ ⟨ x ⟩ ∂ f ) f = 0 − 1 = 1 β N l 2 = k B T N l 2 \begin{aligned} & & \langle x \rangle &= (2 N p_+ - N) l = N l \left(\frac{2 e^{\beta l f}}{e^{\beta l f} + e^{-\beta l f} } - 1\right) \\ & & &= N l \frac{2 e^{\beta l f} - (e^{\beta l f} + e^{-\beta l f})}{e^{\beta l f} + e^{-\beta l f} } \\ & & &= N l \frac{e^{\beta l f} - e^{-\beta l f}}{e^{\beta l f} + e^{-\beta l f} } \\ & & &= N l \frac{\sinh(\beta l f)}{\cosh(\beta l f)} = N l \tanh(\beta l f) \\ \Rightarrow & & \frac{\partial \langle x \rangle}{\partial f} &= \beta N l^2 \frac{\sinh'(\beta l f) \cosh(\beta l f) - \sinh(\beta lf )\cosh'(\beta l f) }{\cosh^2(\beta l f)}\\ & & &= \beta N l^2 \frac{\cosh^2(\beta l f) - \sinh^2(\beta l f) }{\cosh^2(\beta l f)} = \beta N l^2 \frac{1}{\cosh^2(\beta lf)} \\ \Rightarrow & & k &= \left(\frac{\partial \langle x \rangle}{\partial f}\right)_{f=0}^{-1} = \frac{1}{\beta N l^2} = \boxed{\dfrac{k_B T}{N l^2}} \end{aligned} with the reciprocal value β = 1 k B T \beta = \frac{1}{k_B T} of the thermal energy. Numerical evaluation yields k = 1.38 ⋅ 1 0 − 23 J / K ⋅ 293 K 1 0 4 ( 0.35 ⋅ 1 0 − 9 m ) 2 ≈ 3.3 ⋅ 1 0 − 6 N m k = \frac{1.38 \cdot 10^{-23} \,\text{J}/\text{K} \cdot 293 \,\text{K}}{10^4 (0.35 \cdot 10^{-9} \,\text{m})^2} \approx 3.3 \cdot 10^{-6} \,\frac{\text{N}}{\text{m}}

1 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...