Rubik's Cube Destruction
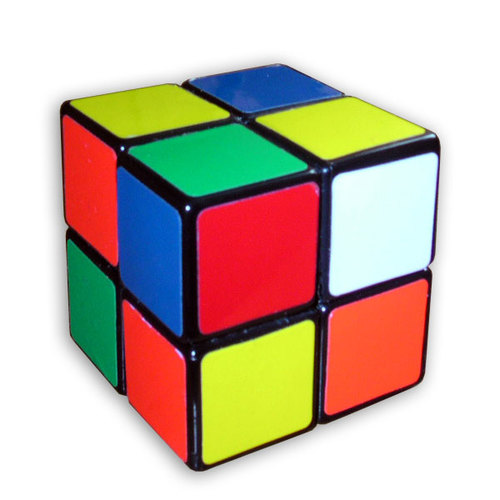 Tony has become frustrated with his
2
×
2
×
2
pocket cube and he's decided to destroy it by taking off all of the stickers.
Tony has become frustrated with his
2
×
2
×
2
pocket cube and he's decided to destroy it by taking off all of the stickers.
How many possible orders are there in which Tony could take off the stickers?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
16 solutions
oh but there will be 4 of six types so ans must be 24!/4!^6.......cause in rubics cube we have each face with four squres of same color.......must reply...............
Log in to reply
Actually, since here it is asked that in how many ways the stickers can be removed from the cube and there are 24 stickers in total, so removing any colour sticker from one side and then another of the same colour from the other side will not be considered the same as the reverse case. Thus, this is an example of permutation without repetitions.
Log in to reply
salam g ap ko Prasun Biswas Prasun Biswas,
Thank you for your explanation
we can also note that every individual sticker is unique as each of them have different adjacent stickers. Thus, it will make a difference, even if you are considering the stickers of the same faces.
Yes, I was stuck for a moment because I took out the repetitions...these kind of problems require clarification
Suyash you are absolutely right
No. Four squares of same color will then have combinations of which corner first up-left, up-right, down-left and down-right....24! is the only answer.
i am with suyash gupta 24!/(4!)^6
good one!
Uurgh..my bad..i am confused of the question in first place
The question should specify that all the stickers are distinct, so that others would not mistake that removing stickers of the same colour of different order does not count as a new way
Thanks for giving such nice solution
I'd like to note that it's spelled "Rubik's". But anyways, there are 6 sides to a cube, and 4 stickers on each side. Therefore, there are 24 stickers to take off. That's 24 times 23 times 22... etc., or 24!. I also posted a problem about 2x2x2 Rubik's Cubes.
What about the elements of symmetry this cube has ? That might reduce the answer to much greater extent. Plz Reply.
Log in to reply
Actually, no two cubes (of the 4 cubes that make up the one larger cube), are the same, unless you peel of stickers. Because they are all corners, they will have 3 colors touching them, and no 3 are the same 3 for any of the 8 vertices, so there are no lines of symmetry. Hope this helps!
we have six sides and 24 stickers so possible is we can remove 24 stickes so answer is 24!
I knew it
There are 4 stickers on each face. So there are 24 stickers in all.
At first you chose any of the 24 stickers, then any one of the rest 23, then any one of the rest 22 , and so on......
So the total number of orders is 24 x 23 x 22 x ..... x 1 = 24!
So here how you do it, there 6 sides of the cube, and each side has 4 colors in it, so you multiply it. So it becomes 24 then add the "!" sign that shows permutation. Permutation is the arrangement of a group in a definite order. so if you were to use this method, your formula would be 24P24 (24x23x22x21.......x1), or you use your calculator to calculate 24P24
I did not think of that Thank u for the help
each side contains 4 stickers and all 6 sides contains 4*6=24 Hence 24! different ways to take out the stickers.
On every side of the cube there are four colored stripes. Therefore, in total there are 8 X 4=32 coloured plates to be removed. These can be removed in 24! because the permutations of these 24 plates are 24!.
There are 6×4=24 stickers in a 2×2×2 pocket cube.
So, the answer is 24!
Each sticker is on a specific side and on a specific corner. If you think of each of these positions as unique then you have: 6 x 4 = 24 positions.
You have 24 positions to choose from at the beginning. Once you take off one sticker, then you have 1 less position to choose from.
Each time you take off a sticker you multiply the possible positions to the previous possible positions to get the number possible orders you could have taken the stickers in. (e.g. If you want to know the possible orders to take off 2 stickers, then 24 x 23 = 552 possible orders).
In this case we want the possible orders to take off all the stickers:
2 4 × 2 3 × 2 2 × ⋯ × 1 = 2 4 !
This was a great solution, thanks for writing it up.
As, there are total 6 sides and 4 blocks on each .Therefore, 6*4=24 . That's answer :)
There is 4 sticker on 1 side and there is 6 side in a cube so, 6 x 4 is equals to 24. As you can see there is 6x 4 in the choices, that is just to confuse you.
was tony's aim to destroy the rubik's cube or just remove all of the stickers . . . Because if tony wanted to destroy the cube then he could have done it by only striking off any two colours of sticker in the cube and then he would have 8! possible orders
Since it is a 2 x 2 x 2 cube it will have 24 distinct stickers. So to destroy it he can select any of the 24 stickers. So the total number of possibilities are 24 C 1 X 23 C 1 X .... X 1 C 1 = 24!
24 stickers so 24! ways to rip them off
they is a boy playing with a100 coins .if he took 10 coin from total coin randomly ,so what is probability at 11th coin
2 2 2 cube has 24 faces. therefore, first we can take out any of the 24 stickers, after that any of the remaining 23 stickers, after that any of the remaining 22 stickers, after that any of the remaining 21 stickers, after that any of the remaining 20 stickers, so on till we have to choose the last sticker. therefore, now we will multiply all the steps as they are occurring in the same time! i.e., 24 23 22 21 20 19... 2*1=24!
i am sorry, the last line should be 24 23 22 21 20 19... 2*1=24!
Since, there are 6 faces of the cube and each face has 4 stickers, so there are a total of ( 6 × 4 ) = 2 4 stickers in the cube.
Now, Tony can take off a sticker from any side in 2 4 ways and corresponding to the first sticker taken off, he can take off any one of the remaining stickers in 2 3 ways and corresponding to the second sticker taken off, he can take off any one of the remaining stickers in 2 2 ways and so on.
Thus, the total no. of ways to take off the stickers = ( 2 4 × 2 3 × 2 2 × . . . . . . . × 1 ) = 2 4 !
Also, we can say that the stickers can be taken off in 2 4 P 2 4 = 2 4 ! ways.