Same Height, Same Area, Different Volumes
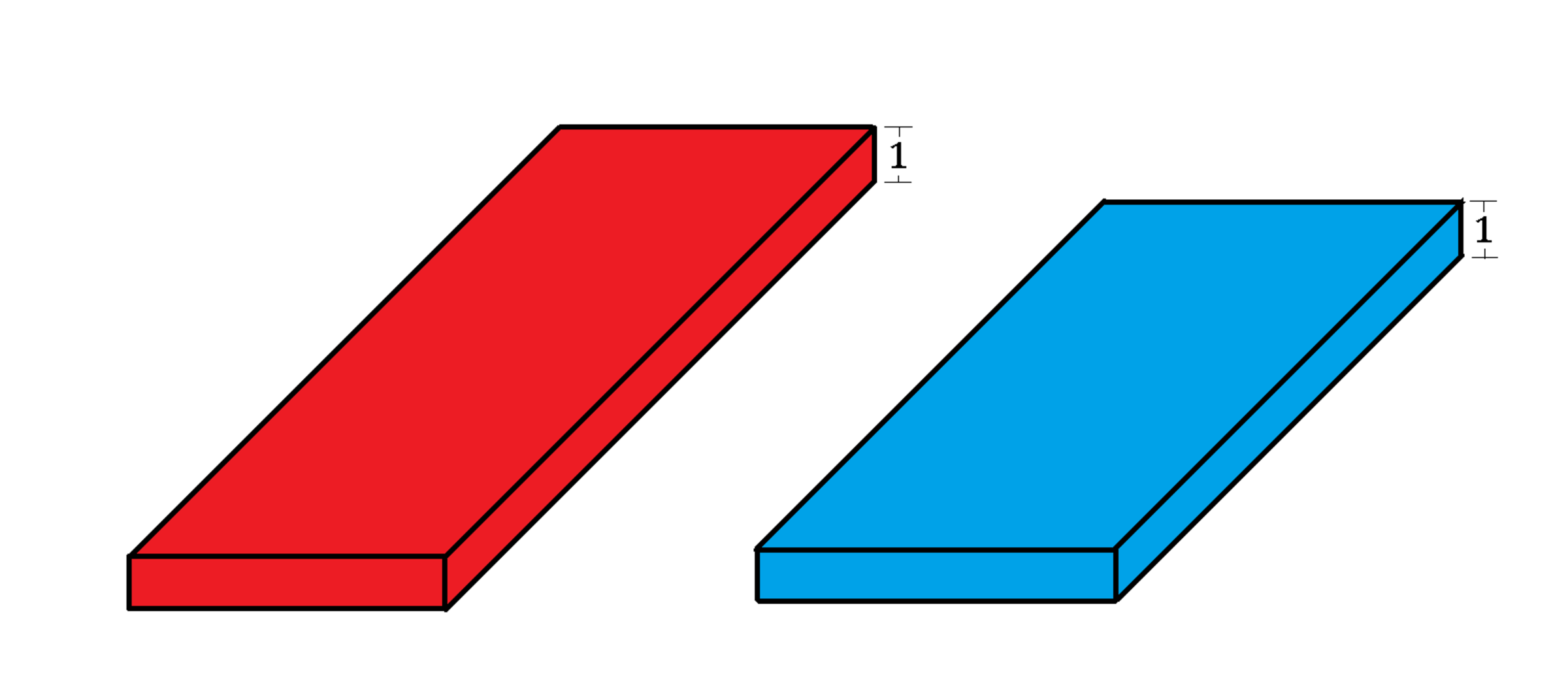
Two cuboid boxes of integer dimensions both have the same height of cm. and same total surface area of , but the volume difference between these boxes is .
What is the volume of the bigger box in ?
Inspired by Number Pyramids
The answer is 66.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let a , b be the width and length of the bigger box and c , d be that of the other box respectively.
The total surface area = 1 6 6 = 2 ( a b + a + b ) = 2 ( c d + c + d ) .
8 3 = a b + a + b = c d + c + d .
The volume difference = 1 = a b − c d . a b = c d + 1
Hence, a + b + 1 = c + d . 1 = ( c + d ) − ( a + b ) .
Now let us rewrite the equation in a more composite form:
8 3 = a b + a + b = ( a + 1 ) ( b + 1 ) − 1 . 8 4 = ( a + 1 ) ( b + 1 )
8 3 = c d + c + d = ( c + 1 ) ( d + 1 ) − 1 . 8 4 = ( c + 1 ) ( d + 1 )
Thereby, we are trying to find the factors of 8 4 , whose pair sums are different by 1 , and we will obtain 8 4 = 7 × 1 2 = 6 × 1 4 since ( 6 + 1 4 ) − ( 7 + 1 2 ) = 1 .
As a result, a = 7 − 1 = 6 ; b = 1 2 − 1 = 1 1 . c = 6 − 1 = 5 ; d = 1 4 − 1 = 1 3 .
Checking the answers: 2 ( 6 ⋅ 1 1 + 6 + 1 1 ) = 1 6 6 = 2 ( 5 ⋅ 1 3 + 5 + 1 3 ) .
Then the volume of the bigger box = 6 ⋅ 1 1 = 6 6 and the volume of the smaller box = 5 × 1 3 = 6 5 .