SAT Absolute Value

Correct Answer: B

Solution 1:

Tip: $|x| = \begin{cases} -x &\mbox{if } x < 0 \\ x & \mbox{if } x \geq 0. \\ \end{cases}$
We use the definition of absolute value to conclude that

for $x < 0$ , we have $f( |x| ) = f ( - x )$ and
for $x > 0$ , we have $f( |x| ) = f(x)$ .

Hence, we want to keep the positive x-axis, and also flip it across the y-axis to obtain the graph of the negative x-axis.

This gives us the graph of (B).

Solution 2:

Tip: $|x| = \begin{cases} -x &\mbox{if } x < 0 \\ x & \mbox{if } x \geq 0. \\ \end{cases}$
Tip: When transforming graphs, trace what happens to each point.

$f(|4| ) = f(4)$
$f( |3| ) = f(3)$
$f( |2| ) = f(2)$
$f( |1| ) = f(1)$
$f( |0| ) = f(0)$

These 5 points are represented in red below. They are retained from the original graph, which is in black.

$f( |-1| ) = f(1)$
$f( |-2| ) = f(2)$
$f( |-3| ) = f(3)$
$f( |-4| ) = f(4)$

These 4 points are represented in blue above. We connect the red and the blue points to form the shape of the new graph, which is in a dotted line. This is the graph of (B).

---

---

Incorrect Choices:

(A)
This is the graph of $y = |f(x) |$ .

(C)
This is the graph of $y = | f( |x| ) |$ .

(D)
This is the graph of $y = f(x)$ .

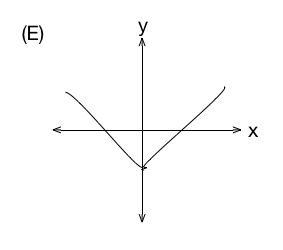
(E)
This is the graph of $y = f( -|x| )$ .

Replica of +be x on - be x...