SAT1000 - P648

Geometry Level 4

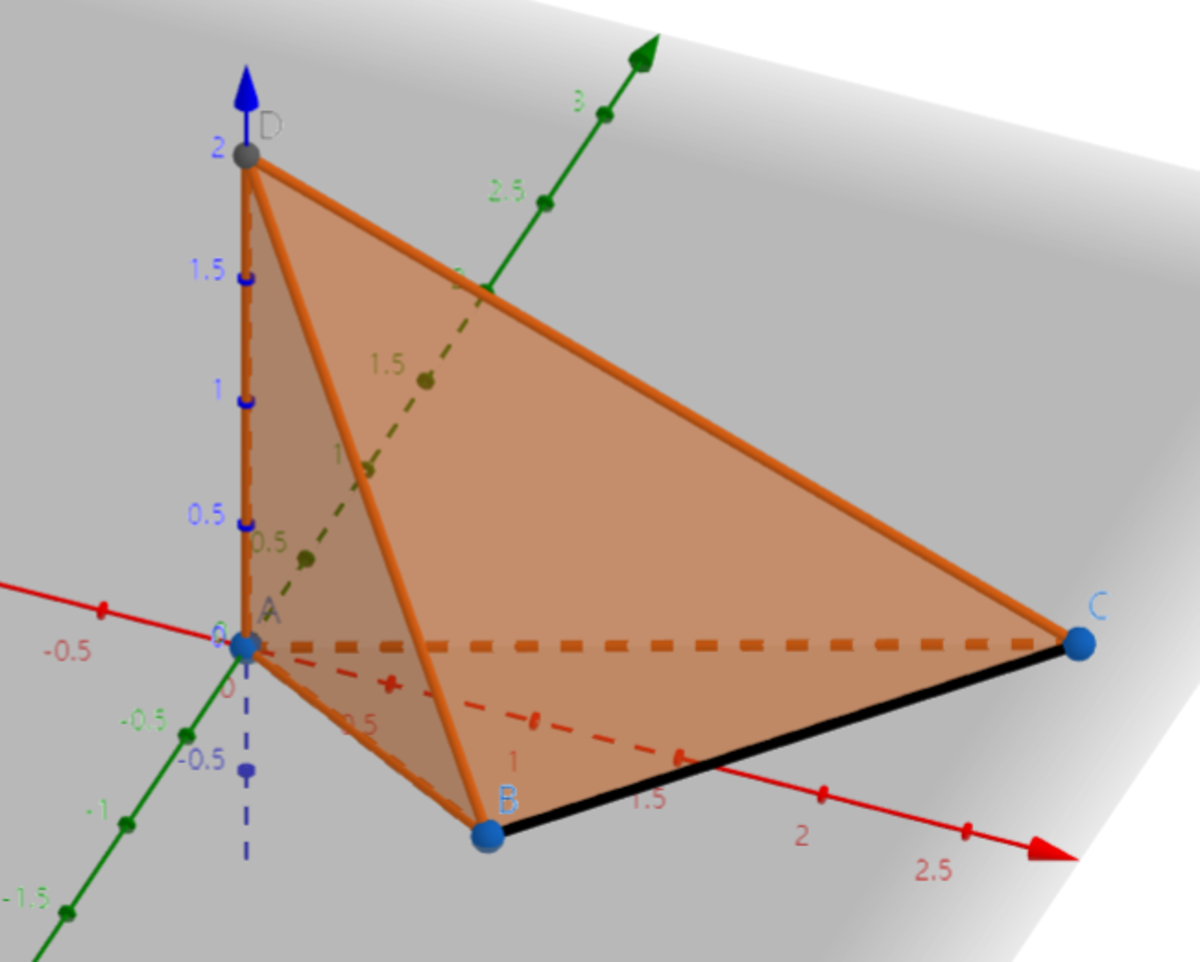
As shown above, in pyramid A B C D ABCD , A D ⊥ B C AD \perp BC , if A D = B C = 2 AD=BC=2 , A B + B D = A C + C D = 4 AB+BD=AC+CD=4 , then find the maximum volume for pyramid A B C D ABCD .

Let V V denote the maximum volume. Submit ⌊ 1000 V ⌋ \lfloor 1000V \rfloor .

Have a look at my problem set: SAT 1000 problems


The answer is 942.

Alice Smith by Alice Smith , 20, China

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Volume will be maximum when the tetrahedron is regular. Then the length of each side of the tetrahedron will be 2 2 , and the volume will be 2 2 3 ≈ 0.9428 \dfrac{2\sqrt 2}{3}\approx 0.9428 .

So the required answer is 942 \boxed {942} .

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...