SAT1000 - P810
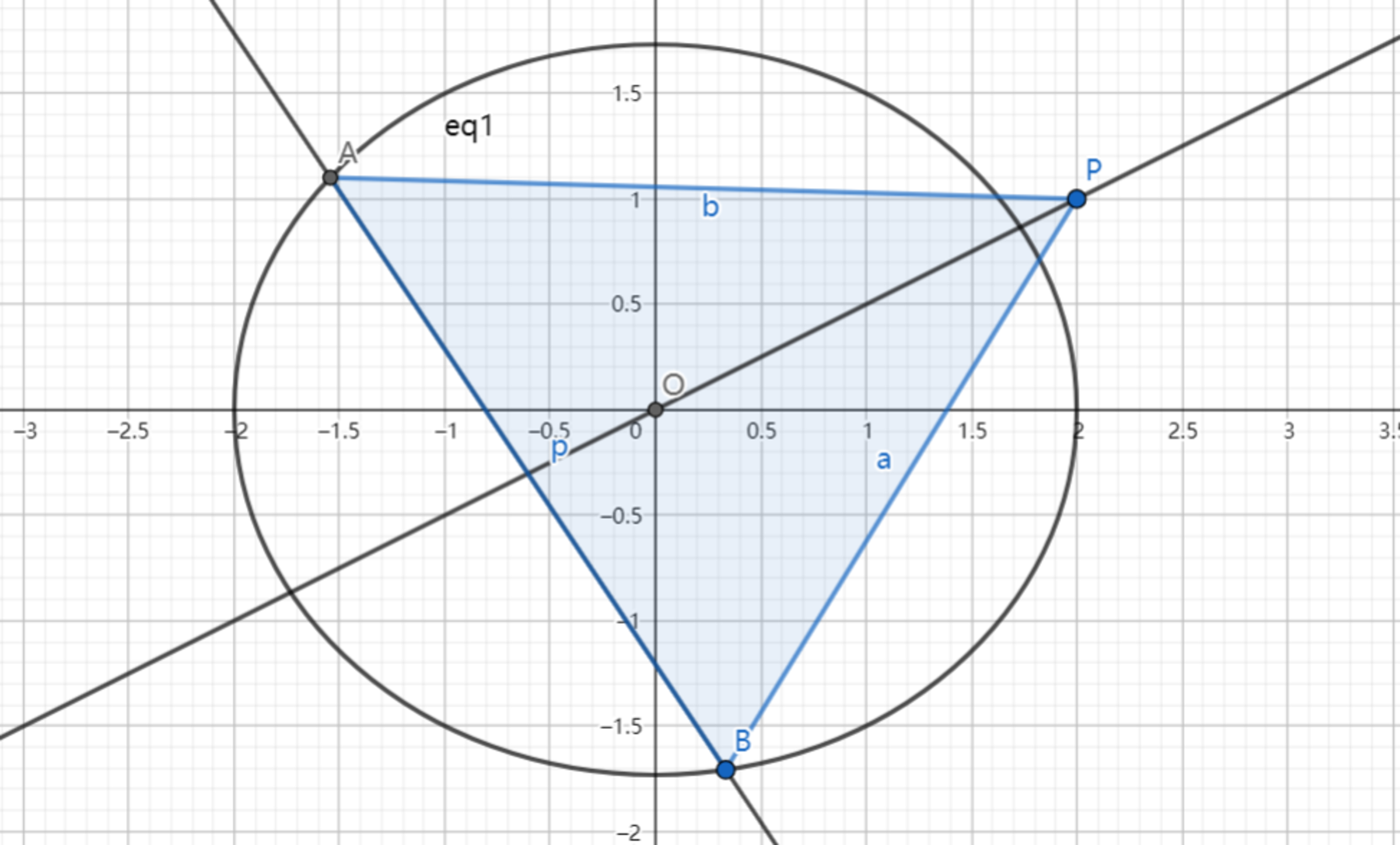
As shown above, the ellipse , , line intersects with at point , and line bisects segment .
If the area of reaches the maximum value, then find the equation of line .
The equation of the line can be expressed as , submit .
Have a look at my problem set: SAT 1000 problems
The answer is -3146.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let D be the intersection of O P and A B , and since O P has an equation of y = 2 1 , let its coordinates be D ( p , 2 1 p ) .
Let the slope of A B be m . Then the equation of the line A B through D ( p , 2 1 p ) is y = m x − m p + 2 1 p , and x -coordinates of A and B at the intersection of y = m x − m p + 2 1 p and 4 x 2 + 3 y 2 = 1 are A x = 4 m 2 + 3 4 m 2 p − 2 m p − ( − 1 2 m 2 + 1 2 m − 3 ) p 2 + 4 8 m 2 + 3 6 and B x = 4 m 2 + 3 4 m 2 p − 2 m p + ( − 1 2 m 2 + 1 2 m − 3 ) p 2 + 4 8 m 2 + 3 6 .
Since D is the midpoint of A and B , 2 1 ( A x + B x ) = D x , or 2 1 ( 4 m 2 + 3 4 m 2 p − 2 m p − ( − 1 2 m 2 + 1 2 m − 3 ) p 2 + 4 8 m 2 + 3 6 + 4 m 2 + 3 4 m 2 p − 2 m p + ( − 1 2 m 2 + 1 2 m − 3 ) p 2 + 4 8 m 2 + 3 6 ) = p , which solves to m = − 2 3 .
Substituting m = − 2 3 , the coordinates A and B simplify to A ( p − 3 1 − 3 p 2 + 9 , 2 1 p + 2 1 − 3 p 2 + 9 ) and B ( p + 3 1 − 3 p 2 + 9 , 2 1 p − 2 1 − 3 p 2 + 9 ) .
The area of △ A P B is then A △ A P B = 2 1 ( ( A x − 2 ) ( B y − 1 ) − ( B x − 2 ) ( A y − 1 ) ) , which after substituting the above values and simplifying solves to A △ A P B = 3 2 ( 2 − p ) − 3 p 2 + 9 .
Since A △ A P B ′ ′ ≤ 0 , the area of the triangle reaches a maximum when the derivative is equal to zero, or A △ A P B ′ = − 3 p 2 + 9 4 p 2 − 4 p − 6 = 0 , which solves to p = 2 1 ( 1 − 7 ) for p < 0 .
Therefore, after substituting m = − 2 3 and p = 2 1 ( 1 − 7 ) into the equation of the line A B given above as y = m x − m p + 2 1 p , it solves to y = − 2 3 x + 1 − 7 , so k = − 2 3 , b = 1 − 7 , and ⌊ 1 0 0 0 ( k + b ) ⌋ = − 3 1 4 6 .