SAT1000 - P838
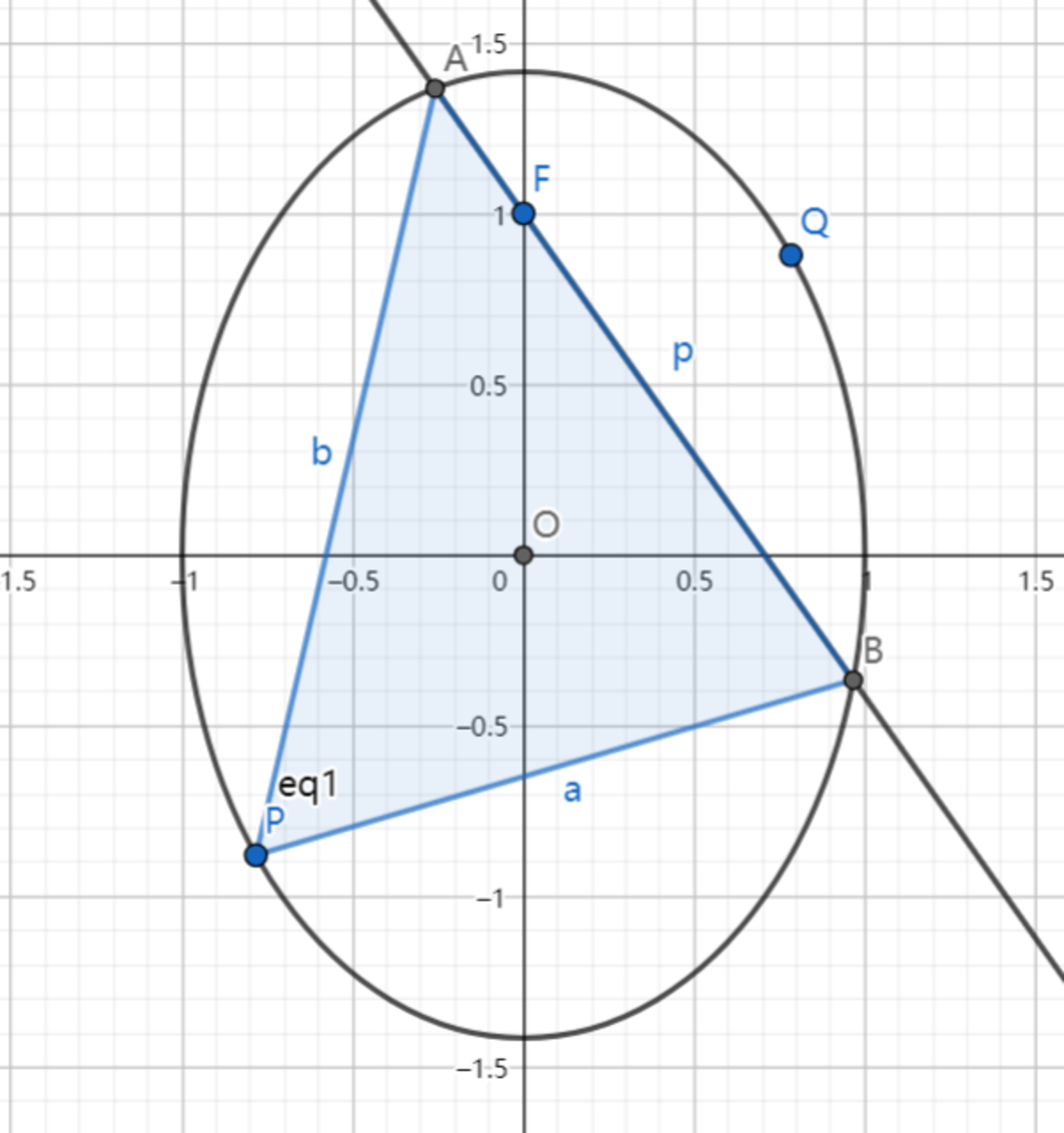
As shown above, the ellipse has equation: , , line intersects with the ellipse at point , and is a point on the ellipse so that .
Point are symmetry about point , and it turns out are on the same circle.
The radius of the circle is . Submit .
Have a look at my problem set: SAT 1000 problems
The answer is 1243.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The intersection of x 2 + 2 y 2 = 1 and y = − 2 x + 1 is at A ( 4 2 − 4 6 , 2 1 + 2 3 ) and B ( 4 2 + 4 6 , 2 1 − 2 3 ) .
Since O A + O B + O P = 0 , A x + B x + P x = 0 and A y + B y + P y = 0 , so 4 2 − 4 6 + 4 2 + 4 6 + P x = 0 and 2 1 + 2 3 + 2 1 − 2 3 + P y = 0 , which solves to P ( − 2 2 , − 1 ) . Since Q is symmetrical to P about O , Q has coordinates Q ( 2 2 , 1 ) .
The center C of the circle with A , P , B , and Q is on the perpendicular bisector of P Q , which is y = − 2 2 x , and the perpendicular bisector of A B , which is y = 2 2 x + 4 1 , and these intersect at C ( − 8 2 , 8 1 ) .
The radius r of the circle can be found by finding the distance C Q , which is r = ( 2 2 − − 8 2 ) 2 + ( 1 − 8 1 ) 2 = 8 3 1 1 .
Therefore, ⌊ 1 0 0 0 r ⌋ = 1 2 4 3 .