Seesaw
In the playground there is a seesaw of length held in the middle by two springs at a distance of from each other. The springs each have a spring constant of . Without load, the seesaw has a height of .
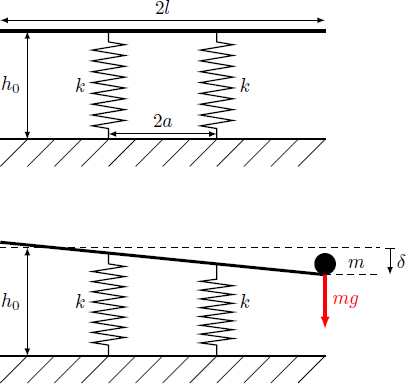
Now a stone of mass is placed on one end of the seesaw.
What is the vertical deflection of the seesaw at the place where the stone lies?
Assume that the seesaw and the springs are massless.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
To the deflection of the mass and the lengths of springs arise geometrically based on the following diagram:
The position and orientation of the seesaw can be described by the height h of its center and the angle of inclination α . The vertical deflection then gives δ = ( h 0 − h ) + l sin α ≈ ( h 0 − h ) + l α + O ( α 3 ) The lengths s 1 and s 2 of the springs results acccordingly s 1 , 2 = a 2 ( 1 − cos α ) 2 + ( h ± a sin α ) 2 = h 2 + 2 a 2 ( 1 − cos α ) ± 2 a h sin α Now we make an Taylor expansion of length s 1 and s 2 as a function of the variables h and α . Therefore, we need the partial derivatives up the the second order ∂ h ∂ s i ∂ α ∂ s i ∂ h 2 ∂ 2 s i ∂ α 2 ∂ 2 s i ∂ α ∂ h ∂ 2 s i = ∂ h ∂ α ∂ 2 s i = s i h ± a sin α = s i a 2 sin α ± a h cos α = s i 1 − s i 3 ( h + a sin α ) 2 = s i a 2 cos α ∓ a h sin α − s i 3 ( a 2 sin α ± a h cos α ) 2 = ± s i a cos α − s i 3 ( h ± a sin α ) ( a 2 sin α ± a h cos α ) The second order Taylor expansion results to s i ≈ s i ∣ h 0 , 0 + ∂ h ∂ s i ∣ ∣ ∣ ∣ h 0 , 0 ( h − h 0 ) + ∂ α ∂ s i ∣ ∣ ∣ ∣ h 0 , 0 α + 2 1 ∂ h 2 ∂ 2 s i ∣ ∣ ∣ ∣ h 0 , 0 ( h − h 0 ) 2 + 2 1 ∂ α 2 ∂ 2 s i ∣ ∣ ∣ ∣ h 0 , 0 α 2 + ∂ h ∂ α ∂ 2 s i ∣ ∣ ∣ ∣ h 0 , 0 ( h 0 − h ) α = h ± a α The total energy of the system is the sum of the elastic energies of the springs and the potential energy of the mass: E ( h , α ) = 2 k ( s 1 − h 0 ) 2 + 2 k ( s 2 − h 0 ) 2 − m g δ ≈ 2 k ( h − h 0 + a α ) 2 + 2 k ( h − h 0 − a α ) 2 − m g ( h 0 − h + l α ) ≈ k ( h − h 0 ) 2 + k a 2 α 2 − m g ( h 0 − h + l α ) In equilibrium, the energy als function of h and α is minimal. Therefore, both partial derivatives must be zero 0 = ! ∂ h ∂ E ⇒ h 0 = ! ∂ α ∂ E ⇒ α = 2 k ( h − h 0 ) + m g ≈ h 0 − 2 k m g = 2 k a 2 α − m g l ≈ 2 k a 2 m g l Thus, we get the final result δ ≈ h 0 − h + l α ≈ 2 k m g ( 1 + a 2 l 2 ) ≈ 2 ⋅ 1 0 0 0 1 ⋅ 1 0 ( 1 + 9 ) m = 5 cm