Shadow doesn't always follow you!

Classical Mechanics Level 4

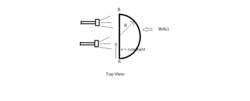A small block can move in a straight horizontal line A B AB .Flash lights from one side projects its shadow on a curved vertical wall which has a horizontal cross section as a circle. If tangential and normal acceleration of shadow of the block on the wall as a function of time can be represented as:

a N = c v R ( 2 R t − v t 2 ) , a T = R ( v t − R ) v d e ( 2 R t − v t 2 ) f g a_N=\frac{cvR}{(2Rt-vt^2)} \quad , \quad a_T=\frac{R(vt-R)v^{\frac{d}{e}}}{(2Rt-vt^2)^{\frac{f}{g}}} .

Find ∣ c ∣ + ∣ d ∣ + ∣ e ∣ + ∣ f ∣ + ∣ g ∣ |c|+|d|+|e|+|f|+|g|

Details and Assumptions

  • As shown in figure v v is the constant along A B AB .

  • Given Figure is top view of the setup.

  • c , d , e , f , g c,d,e,f,g are natural numbers and d e , f g \frac{d}{e} ,\frac{f}{g} are in simplest forms.

  • a N , a T a_N,a_T represent normal and tangential accelerations respectively.

  • you can neglect minus sign in accelerations due to directions.


The answer is 9.

View wiki
Gautam Sharma by Gautam Sharma , 23, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Gautam Sharma
Mar 20, 2015

Let us assume origin to be at Mid point of AB.

Equation of curve ⇒ x 2 + y 2 = R 2 \Rightarrow x^2+y^2=R^2

After time t t

Co-ordinates of shadow ( 2 v t R − v 2 t 2 , v t − R ) (\sqrt{2vtR-v^2t^2},vt-R)

Hence v x = d x d t = v R − v 2 t 2 v t R − v 2 t 2 v_x=\frac{dx}{dt}=\frac{vR-v^2t}{\sqrt{2vtR-v^2t^2}} and v y = v v_y=v

Now a x = d v x d t = − v 2 R 2 2 v t R − v 2 t 2 3 2 a_x=\frac{dv_x}{dt}=\frac{-v^2R^2}{{2vtR-v^2t^2}^\frac{3}{2}} and a y = 0 a_y=0

Now we see that a x c o s ( z ) = a N a_x cos(z)=a_N and a x s i n ( z ) = a T a_x sin(z)=a_T

c o s ( z ) = 2 v t R − v 2 t 2 R cos(z)=\frac{\sqrt{2vtR-v^2t^2}}{R}

s i n ( z ) = v t − R R sin(z)=\frac{vt-R}{R} .

Substituting values we get

a N = v R ( 2 R t − v t 2 ) , a T = R ( v t − R ) v 1 2 ( 2 R t − v t 2 ) 3 2 a_N=\frac{vR}{(2Rt-vt^2)} \quad , \quad a_T=\frac{R(vt-R)v^{\frac{1}{2}}}{(2Rt-vt^2)^{\frac{3}{2}}} .

So c = 1 , d = 1 , e = 2 , f = 3 , g = 2 c=1,d=1,e=2,f=3,g=2

Hence ∣ c ∣ + ∣ d ∣ + ∣ e ∣ + ∣ f ∣ + ∣ g ∣ |c|+|d|+|e|+|f|+|g| =9

5 Helpful 0 Interesting 0 Brilliant 0 Confused

It is just not a level 5 problem, it must be level 4.

Ronak Agarwal - 6 years, 2 months ago

Easy but good problem. I did in this way:

sin ⁡ θ = 2 v r t − v 2 t 2 R . . . . . ( 1 ) cos ⁡ θ = R − v t R . . . . . . . ( 2 ) \displaystyle{\sin { \theta } =\cfrac { \sqrt { 2vrt-{ v }^{ 2 }{ t }^{ 2 } } }{ R } .....(1)\\ \cos { \theta } =\cfrac { R-vt }{ R } .......(2)}

differentiating equation 2nd w.r.t time (using chain rule)

− sin ⁡ θ d θ d t = − v R ω = v R csc ⁡ θ . . . . . . ( 3 ) a N = ω 2 R a T = R α ≡ R ω d ω d θ \displaystyle{-\sin { \theta } \cfrac { d\theta }{ dt } =-\cfrac { v }{ R } \\ \omega =\cfrac { v }{ R } \csc { \theta } ......(3)\\ { a }_{ N }=\cfrac { { \omega }^{ 2 } }{ R } \\ { a }_{ T }=R\alpha \equiv R\omega \cfrac { d\omega }{ d\theta } }

Deepanshu Gupta - 6 years, 2 months ago

Log in to reply

Yeah same I thought of this way just after posting the solution.It makes it much easier to solve but the concept is same.

Gautam Sharma - 6 years, 2 months ago
Vivek Bhagat
Apr 10, 2015

I found the constants d,e,f,g by dimensional analysis, and c was guess, I know its not a proper method!! But I also tried to find it from conventional method

0 Helpful 0 Interesting 0 Brilliant 0 Confused

Did above solution helped u?If yes upvote it so others also see it and reshare the problem too.

Gautam Sharma - 6 years, 2 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...