Shifting Movement

Classical Mechanics Level 2

A cart of mass $M$ is at rest on a frictionless surface. The inside of this cart consists of a ball of mass $m$ that is hanging down from a light string. The string is broken and the ball falls and undergoes many collisions in unpredictable paths until it comes to a rest at a slit made at the bottom of the cart. Let the horizontal distance from the slit in the floor and the ball's initial position be $L$ . What is the displacement of the cart when the ball comes to a rest when it is in the slit?

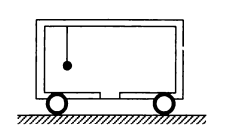
Details and Assumptions

Let the center of mass of the cart be at the center of the slit.

ML / (m + M)

(m + M) / ML

L

mL / (m + M)

1 solution

Raghu Alluri
Mar 30, 2019

We will go about solving this question by looking at the center of mass of the system and comparing the center of masses from the before and after situation. All we really need to deal with is the $X$ center of mass as the $Y$ component does not concern us.

We will choose the position of the center of the slit to be the origin of our coordinate system $K'$ .

Thus we can then express the initial center of mass of the system as such,

$X_{cm} = mL / (m + M)$

The center of mass of the cart after the collisions and when the ball is in the slot would be,

$X_{cm}' = 0$

This is because both the center of mass of the cart and the ball are at the origin.

Therefore,

$ΔX_{cm} = X_{cm} - X_{cm}' = mL / (m + M)$

To conclude, since the center of mass of the system as shifted over by $mL / (m + M)$ , the whole system must have also shifted over by that distance meaning that the cart would have also moved that same distance.

Shifting Movement

1 solution

0 pending reports